Dr. Vadym Zayets
v.zayets(at)gmail.com
My Research and Inventions
click here to see all content |

Dr. Vadym Zayetsv.zayets(at)gmail.com |
|
 |
more Chapters on this topic:IntroductionScatteringsSpin-polarized/ unpolarized electronsSpin statisticselectron gas in Magnetic FieldFerromagnetic metalsSpin TorqueSpin-Torque CurrentSpin-Transfer TorqueQuantum Nature of SpinQuestions & Answers
more Chapters on this topic:IntroductionScatteringsSpin-polarized/ unpolarized electronsSpin statisticselectron gas in Magnetic FieldFerromagnetic metalsSpin TorqueSpin-Torque CurrentSpin-Transfer TorqueQuantum Nature of SpinQuestions & Answers
more Chapters on this topic:IntroductionScatteringsSpin-polarized/ unpolarized electronsSpin statisticselectron gas in Magnetic FieldFerromagnetic metalsSpin TorqueSpin-Torque CurrentSpin-Transfer TorqueQuantum Nature of SpinQuestions & Answers
more Chapters on this topic:IntroductionScatteringsSpin-polarized/ unpolarized electronsSpin statisticselectron gas in Magnetic FieldFerromagnetic metalsSpin TorqueSpin-Torque CurrentSpin-Transfer TorqueQuantum Nature of SpinQuestions & Answers
more Chapters on this topic:IntroductionTransport Eqs.Spin Proximity/ Spin InjectionSpin DetectionBoltzmann Eqs.Band currentScattering currentMean-free pathCurrent near InterfaceOrdinary Hall effectAnomalous Hall effect, AMR effectSpin-Orbit interactionSpin Hall effectNon-local Spin DetectionLandau -Lifshitz equationExchange interactionsp-d exchange interactionCoercive fieldPerpendicular magnetic anisotropy (PMA)Voltage- controlled magnetism (VCMA effect)All-metal transistorSpin-orbit torque (SO torque)What is a hole?spin polarizationCharge accumulationMgO-based MTJMagneto-opticsSpin vs Orbital momentWhat is the Spin?model comparisonQuestions & AnswersEB nanotechnologyReticle 11
more Chapters on this topic:IntroductionScatteringsSpin-polarized/ unpolarized electronsSpin statisticselectron gas in Magnetic FieldFerromagnetic metalsSpin TorqueSpin-Torque CurrentSpin-Transfer TorqueQuantum Nature of SpinQuestions & Answers
more Chapters on this topic:IntroductionScatteringsSpin-polarized/ unpolarized electronsSpin statisticselectron gas in Magnetic FieldFerromagnetic metalsSpin TorqueSpin-Torque CurrentSpin-Transfer TorqueQuantum Nature of SpinQuestions & Answers
more Chapters on this topic:IntroductionScatteringsSpin-polarized/ unpolarized electronsSpin statisticselectron gas in Magnetic FieldFerromagnetic metalsSpin TorqueSpin-Torque CurrentSpin-Transfer TorqueQuantum Nature of SpinQuestions & Answers
more Chapters on this topic:IntroductionScatteringsSpin-polarized/ unpolarized electronsSpin statisticselectron gas in Magnetic FieldFerromagnetic metalsSpin TorqueSpin-Torque CurrentSpin-Transfer TorqueQuantum Nature of SpinQuestions & Answers
more Chapters on this topic:IntroductionTransport Eqs.Spin Proximity/ Spin InjectionSpin DetectionBoltzmann Eqs.Band currentScattering currentMean-free pathCurrent near InterfaceOrdinary Hall effectAnomalous Hall effect, AMR effectSpin-Orbit interactionSpin Hall effectNon-local Spin DetectionLandau -Lifshitz equationExchange interactionsp-d exchange interactionCoercive fieldPerpendicular magnetic anisotropy (PMA)Voltage- controlled magnetism (VCMA effect)All-metal transistorSpin-orbit torque (SO torque)What is a hole?spin polarizationCharge accumulationMgO-based MTJMagneto-opticsSpin vs Orbital momentWhat is the Spin?model comparisonQuestions & AnswersEB nanotechnologyReticle 11
more Chapters on this topic:IntroductionScatteringsSpin-polarized/ unpolarized electronsSpin statisticselectron gas in Magnetic FieldFerromagnetic metalsSpin TorqueSpin-Torque CurrentSpin-Transfer TorqueQuantum Nature of SpinQuestions & Answers
more Chapters on this topic:IntroductionScatteringsSpin-polarized/ unpolarized electronsSpin statisticselectron gas in Magnetic FieldFerromagnetic metalsSpin TorqueSpin-Torque CurrentSpin-Transfer TorqueQuantum Nature of SpinQuestions & Answers |
Spin Statistic
Magnetism of electron gasThe energy distributions of all electrons (spin-polarized electrons+spin-unpolarized electrons) is described by the Fermi-Dirac distribution. The individual distribution of the spin-polarized and spin-unpolarized electrons is described by the spin statistics (see below). The spin-polarized and spin-unpolarized electrons have different energy distributions, because of their different scattering probabilities. The spin statistics is critically important for description of different spin-dependent effect in a solid. For example, the different distributions of the spin-polarized and spin-unpolarized electrons is a reason of a difference of conductivities of the spin-polarized and spin-unpolarized electrons.
|
Energy distribution of single- occupied and double- occupied states for conduction electrons in a non-magnetic material |
| A quantum state can be occupied either by one electron or two electrons of opposite spins. The energy distributions of these single- and double- occupied states are different |
| The energy distribution of all conduction electrons are describes by the Fermi- Dirac distribution |
| (Why distributions are different) |
| (Difference of scattering probabilities) |
| method has been developed in 2014 by Zayets |
| Click on image to enlarge it |
![]() The energy distributions of conduction electrons in a quantum states, which occupied by a single electron and by two electrons of opposite spins, are different, because of difference of their scattering probabilities
The energy distributions of conduction electrons in a quantum states, which occupied by a single electron and by two electrons of opposite spins, are different, because of difference of their scattering probabilities
(reason wthy there is a difference of scattering probabilities) ![]() The electron spin influences the probability of the electron scattering. An electron can be scattered into a quantum state, which is already occupied by one electron, only if the electron spin is opposite to spin of other electron. In contrast, when an electron is scattered in a fully- unoccupied state, there is no limitation on spin direction.
The electron spin influences the probability of the electron scattering. An electron can be scattered into a quantum state, which is already occupied by one electron, only if the electron spin is opposite to spin of other electron. In contrast, when an electron is scattered in a fully- unoccupied state, there is no limitation on spin direction.
There are two groups of conduction electrons in a non-magnetic material: (group 1)![]()
![]() Conduction electrons in states, which are occupied by one electron; (group 2)
Conduction electrons in states, which are occupied by one electron; (group 2) ![]()
![]() Conduction electrons in states, which are occupied by two electrons of opposite spins.
Conduction electrons in states, which are occupied by two electrons of opposite spins.
(group 1)![]()
![]() Conduction electrons in states, which are occupied by one electron. Their distribution is calculated as
Conduction electrons in states, which are occupied by one electron. Their distribution is calculated as
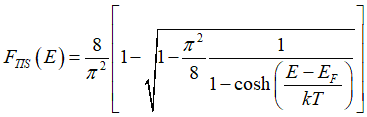
where E is the electron energy
(group 2) ![]()
![]() Conduction electrons in states, which are occupied by two electrons of opposite spins. Their distribution is calculated as
Conduction electrons in states, which are occupied by two electrons of opposite spins. Their distribution is calculated as
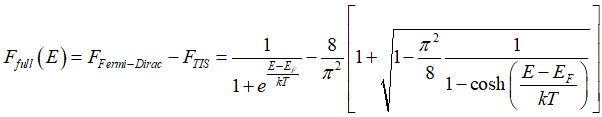
the number of empty places in states, which is not occupied either by one or two electrons is cuclculated as
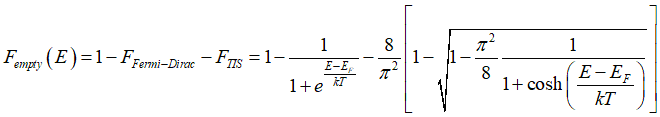
.
![]() The energy distribution of spin-polarized electrons:
The energy distribution of spin-polarized electrons:
Probability FTIA(E), that a conduction electron of energy E is spin-polarized, is calculated as
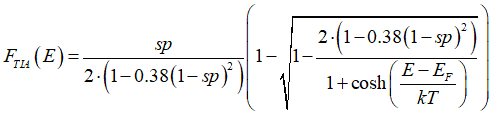
![]() The energy distribution of spin-unpolarized electrons
The energy distribution of spin-unpolarized electrons
Probability FTIS(E), that a conduction electron of energy E is spin-unpolarized, is calculated as

![]() The energy distribution of spin-inactive electrons
The energy distribution of spin-inactive electrons
Probability Ffull(E), that a conduction electron of energy E is spin-inactive, is calculated as

![]() The energy distribution of all electrons (the Fermi-Dirac distribution)
The energy distribution of all electrons (the Fermi-Dirac distribution)
Probability Fall(E) that a conduction electron has energy E is calculated as

![]() Q. Why quantum states filled by one and two electrons should be distinguished???
Q. Why quantum states filled by one and two electrons should be distinguished???
 |
Probability that a quantum state in electron gas is occupied by an electron from group of spin-polarized electrons (TIA assembly) (blue line) or from group of spin-unpolarized electrons (TIS assembly) (green line) or occupied by two electrons of opposite spins (full) (red line) or a state is not occupied by electrons (empty) (cyan line). |
A quantum state, which is filled by two electrons of opposite spins, has zero spin. In contrast, a quantum state, which is filled only by one electron, has spin 1/2. The spin makes a big difference for energy distributions of these states.
It is because of different electron- scattering probabilities between states, which is occupied only by one electron.
When an electron scattered into a state, which is already occupied by one electron, the spin of the scattered electron should be opposite to the spin of the electron, which is already occupying one of two places in the quantum state.
Since spins of all spin-polarized electrons are directed in the same direction, there is no direct scatterings between states occupying by spin-polarized electrons. In contrast, spins of spin-unpolarized electrons are directed in different directions. There is a probability of scatter ins between states, which are occupied by spin-unpolarized electrons. The difference in the scattering probabilities makes different the distributions of spin-polarized and spin-unpolarized electrons.
Q. Why we should care about the energy distributions?
![]() They are critically important for a description of charge and spin transports.
They are critically important for a description of charge and spin transports.
For example, the difference in energy distribution for spin-polarized and spin-unpolarized electrons causes the difference in electrical conductivity for spin-polarized and spin-unpolarized electrons (See here).
Another example is the hole. Only using the spin statistics it is possible to explain the charge and spin transport properties of holes in metals and semiconductors (See here).
Firstly, the electron scatterings probabilities between groups of spin-polarized and spin-unpolarized electrons are calculated for each electron energy. It fixes the ratio between the number of states, which are occupied by two electrons, the number of states, which are occupied by one electron of group of spin-polarized electrons, and the number of states, which are occupied by one electron of group of spin-unpolarized electrons.
Secondly, the energy distributions are calculated from the condition that the sum of 3 probabilities (an electron is either in the group of spin-polarized electrons or in the group of spin-unpolarized electrons or in the group of spin-inactive electrons (which fill the full-filled states) is described by the Fermi-Dirac distribution.
Scattering probabilities |
Spin-polarized to spin-polarized. Scattering probability=0 |
All spin-polarized electrons have spin-up direction,for example. All available empty spaces for scattering are spin-down. Therefore, there is no scattering. click on image to enlarge it |
Spin-polarized to spin-unpolarized. Scattering probability between 0 and 1 |
 |
Only in case when the spin of electron of group of spin-unpolarized electrons is opposite to spin of a electron of group spin-polarized electrons, the scattering occur. Since spins of spin-unpolarized electrons equally distributed in all directions, the scattering probability between 0 and 1. click on image to enlarge it |
Full-filled to half-filled. Scattering probability=1 |
 |
Full-filled state, which is filled by two electrons of opposite spins, has spin direction. Therefore, the spin scattering electron is opposite to the spin of half-filled state. click on image to enlarge it |
The electron scatterings are spin-dependent because an electron only of a fixed spin direction may be scattered into a quantum state, which is already filled by another electron. For example, only an electron with spin-up spin direction can be scattered into a quantum state, which is already filled by a spin-down electron.
(case 1) Scattering probability=0
(a) A scatter of a spin-polarized electron into a state, which already filled by one spin-polarized electron
(case 2) Scattering probability is between 0 and 1.
(a) A scatter of a spin-polarized electron into a state, which already filled by one spin-unpolarized electron
(b) A scatter of a spin-unpolarized electron into a state, which already filled by one spin-unpolarized electron
(c) A scatter of a spin-unpolarized electron into a state, which already filled by one spin-polarized electron
An electron can be scattered into state, which is already filled by one electron, only if spin of scattered electron is opposite to the spin of of the electron, which is already occupying the state. In the group of the spin-unpolarized electrons the spin directions are distributed equally in all directions. Therefore, there is always non-zero probability that an electron has required direction of the spin and the electron can be scattered into a state, which is already occupied by one electron.
(case 3) Scattering probability = 1
(a) A scatter of an electron into a state, which is not occupied by any electron
(b) A scatter of an electron from a full-filled state, which is occupied by two electrons of opposite spins, into a state, which is occupied by one electron.
Total spin of a full-filled state, which is filled by two electrons of opposite spins, is zero and there is no spin direction for this state. Therefore, an electron, which scattered from a full-filled state into a half-filled state, always has spin opposite to the spin of the electron, which is already occupying the half-filled state.
Main part
|
||||
The distribution of the spin directions. (left) small spin polarization (right) large spin polarization. Yellow surface shows distribution of spins in the group of spin-unpolarized electrons. Blue surface shows distribution of spin in the group of the spin-polarized electrons. The distance from (0,0,0) point to the surface is proportional to the number of states, which is filled by one electron. The spin directed along corresponded direction. |
A. The energy distribution F(E) is a probability to find electron at energy E.
The energy distributions of electrons and holes in a metal are determined by the Fermi-Dirac statistic

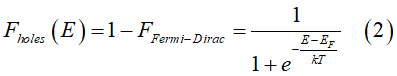
![]() All conduction electrons consist of groups of spin-polarized, spin-unpolarized and spin-inactive electrons. Each group is described by its own distribution: (1) FTIS is the distribution of spin-polarized electrons; (2) FTIA is the distribution of spin-unpolarized electrons; (3) Ffull is the distribution of full states. Each full state is occupied by two spin-inactive electrons; (4) Fempty is the distribution of states, which is not occupied.;
All conduction electrons consist of groups of spin-polarized, spin-unpolarized and spin-inactive electrons. Each group is described by its own distribution: (1) FTIS is the distribution of spin-polarized electrons; (2) FTIA is the distribution of spin-unpolarized electrons; (3) Ffull is the distribution of full states. Each full state is occupied by two spin-inactive electrons; (4) Fempty is the distribution of states, which is not occupied.;
![]() Condition 1: The sum of three distributions of each group of electrons gives the distribution of all electrons (Eq.1):
Condition 1: The sum of three distributions of each group of electrons gives the distribution of all electrons (Eq.1):
Electron gas in the absence of a spin accumulation consists of (1) spin-unpolarized electrons; (2) spin-inactive electrons. The condition 1 are described as
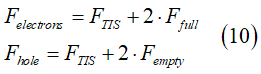
Electron gas in the spin-polarized electron gas consists of (1) spin-polarized electrons; (2) spin-unpolarized electrons; (3) spin-inactive electrons. The condition 1 are described as
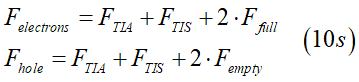
The symmetry of scatterings fixes the the relations between FTIS , FTIA and Ffull. Substitution them into Eqs.(10) or Eqs.(10s) gives the system of equations from which FTIS , FTIA and Ffull are calculated
A. Only dependencies of scattering probabilities on the spin direction is calculated. For example, an spin-up electron can be scattered into a "spin" state, which is already occupied by one electron, only if the spin-up place is available or the same if the state is occupied by a spin-down electron. As a result, the scattering probability of spin-up electron into spin-down "spin" state is the largest (= one). The scattering probability of spin-up electron into spin-up "spin" state is the smallest (= zero). The scattering probability for other directions is between zero and one. The spin direction of spin unpolarized electrons are distributed equally in all directions. In order to calculate the scattering probability of a spin-unpolarized electron, the scattering probability is integrated over all possible spin directions.
In the case when the electron gas is not spin-polarized, a conduction electron may occupy ether a "spin" state or "full" states. There are also "empty" states, which are not occupied.. The ratio between the numbers of these 3 states is determined by the condition that the rate of conversion of "spin" states into "full" states and "empty" states (scattering event 4) is equal to the rate of back conversion of "full" states and "empty" states into "spin" states (scattering event 5).
![]() Main idea of the calculation:
Main idea of the calculation:![]() Firstly, the scattering probabilities between spin-unpolarized electrons and spin-inactive electrons are calculated. It gives the ratio between numbers between electrons in each group.
Firstly, the scattering probabilities between spin-unpolarized electrons and spin-inactive electrons are calculated. It gives the ratio between numbers between electrons in each group.![]() Secondly, the distribution for each group is calculated from the condition that the energy distribution of all electrons is described by the Fermi-Dirac distribution.
Secondly, the distribution for each group is calculated from the condition that the energy distribution of all electrons is described by the Fermi-Dirac distribution.
![]() What is calculated below?:
What is calculated below?:![]() Probability FTIS that a quantum state is occupied by one electron or probability, that a conduction electron belong to the group of spin-unpolarized electrons;
Probability FTIS that a quantum state is occupied by one electron or probability, that a conduction electron belong to the group of spin-unpolarized electrons; ![]() Probability Ffull that a quantum state is occupied by two electron or probability, that a conduction electron belong to the group of spin-inactive electrons.
Probability Ffull that a quantum state is occupied by two electron or probability, that a conduction electron belong to the group of spin-inactive electrons.
Three groups of conduction electrons |
|||||||||
|
|||||||||
Each quantum state can be occupied either by one or two conduction electrons. The amount of filling and distribution of spin directions distinguish between electrons of different groups. See more details here. click on image to enlarge it |
A. It is because an electron with a fixed direction of spin can be scattered only to an unoccupied quantum state in which this spin direction is allowed. For example, a spin-up electron can be scattered only ether into a fully empty state or a state, which is occupied by one spin-down electron and in which spin-up position is not occupied.
A. Firstly, the scattering probability is calculated for a fixed spin angle of a scattered electron. Secondly, the probability is integrated over all possible spin angles of a scattered electron and spin angles of possible quantum state, to which the electron is scattered. Spins of spin-polarized electrons are directed in one direction. Spins of spin-unpolarized electrons are distributed equally in all directions. The spin-inactive electrons have no defined spin direction. Therefore, their scatterings is not limited by the spin direction.
Main part:
A. If in the case when the electron gas is not spin-polarized, a quantum state can be occupied either by one or by two electrons. The spin properties of these two kinds of electrons are very different. Their equilibrium concentration is calculated from the condition that the scattering rate from one group to another group is equal to the scattering rate in the opposite direction.
 Main idea beyond the calculations of the spin statistics: take into account spin features of all possible scatterings
Main idea beyond the calculations of the spin statistics: take into account spin features of all possible scatteringsIn the scattering event 4 an electron from a “full” state is scattered into an “empty” state. As a result, the number of “full” and “empty” states decreases and the number of “spin” states increases. In the scattering event 5 an electron from a “spin” state is scattered into another “spin” state. As a result of this scattering, the number of “full” and “empty” states increases and the number of “spin” states decreases. The average number of “spin”, “full” and “empty” states in a metal is determined by the condition, that scatterings of “spin” states into “full” + “empty” states are balanced by the scatterings of “full” + “empty” states back into “spin” states.
The ratio of "spin" states to "empty"/"full" states is determined by the balance of the conversion of "spin" states into "full"/"empty" states (scattering event 5) and the reverse conversion of the "full"/"empty" states into "spin" states (scattering event 4).
The result of an electron scattering out of a “full” state into an “empty” is two “spin” states. Therefore, scattering event 4 causes an increase of the number of “spin” states with the rate

where Pscattering is is the probability of an electron scattering event per unit time.
Spin statistics Electron gas is not spin-polarized |
|---|
|
| Fig 2. Occupation probability of "spin", "full" and "empty" states in absence of spin accumulation. The electrons occupying the "spin" states are called spin-unpolarized electrons. Electrons occupying the "full" states are called spin-inactive electrons. (See here) |
A scattering of an electron out of a “spin” state into an empty place of another “spin” state (the scattering event 5) reduces the number of “spin” states. The result of such a scattering is either two "spin" states or a "full" state +a "empty" state. The probability of a scattering of spin states into "empty" +"full" states by this scattering event depends on the angle between the spin directions of “spin” states
![]()
where φ is the angle between the spin directions of the spin states.
If Fspin(φ) is the distribution of “spin” states, which have the angle φ with respect to the z-axis, the probability of scattering of "spin" states with angles φ1 , φ2 is calculated as
![]()
Since the spin of a spin- unpolarized electron can have any direction with equal probability, the angular distribution Fspin(φ) of the spin-unpolarized electrons can be calculated as
![]()
where Fspin is the number of “spin” sates at energy E.
From Eqs. (14) ,(15), the reduction rate of “spin” states due to the the scattering event 5 can be calculated by integrating Eq.(14) over all possible angles φ1 , φ2 as
 |
Fig 3 Ratio of electrons in TIS assembly in "spin" states to the total number of electrons (red line) and ratio of holes in "spin" states to the total number of holes (black line) |

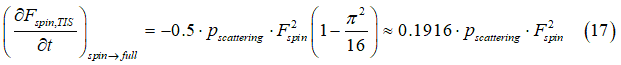
In equilibrium, the conversion rate of “spin” states into “full” states Eq.(17) is equal to the rate of the back conversion Eq.(12)
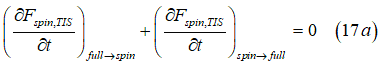
Substituting Eq. (11) and Eq. (17) into Eq. (17a) gives
![]()
Solving the system of Eqs. (10), (17b) and using Eqs. (1) and (2), the distribution FTIS of spin-unpolarized electrons in spin-unpolarized electron gas can be calculated as

and distribution Ffull of spin-inactive electrons in spin-unpolarized electron gas is calculated as
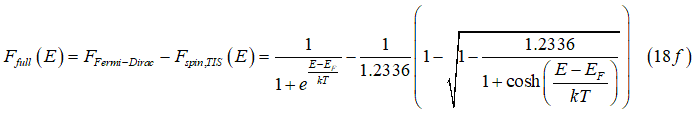
The system of Eqs. (10) and (17b) is

Substituting the 2d and 3d Eqs. (18.1) into the 1st Eq. gives

The solution of the last equation of (18.2) gives
![]()
From Eqs. (1) and (2) we have

Substituting (18.4) into (18.3) gives
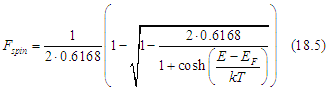
Figure 2 shows the distribution of "spin", "full" and "empty" states in a spin-unpolarized electron gas calculated from Eqs. (18). The "spin" states are mainly distributed near the Fermi energy. The "full" states are mainly distributed at lower energies and "empty" states are mainly distributed at higher energies. Figure 3 shows the ratio of electrons in “spin” states to the total number of electrons (red line) and the ratio of holes in “spin” states to the total number of holes (black line). It should be noticed that for energies larger than ~2 kT above the Fermi energy the number of "spin" states is significantly greater than the number of "full" states. This means that in this case almost all electrons are in "spin" states. Similarly, for energies smaller than ~2 kT below the Fermi energy, all holes are in "spin" states. (more about the holes is here)
The total number of “spin” states in the group of spin-unpolarized electrons can be calculated as
![]()
where D(E) is the density of states
In the case of a metal, which has a nearly constant density of states near the Fermi energy, the total number of “spin” states can be calculated as
![]()
![]()
![]() Therefore, the number of “spin” states increases linearly with temperature.
Therefore, the number of “spin” states increases linearly with temperature.
 |
Fig 5. Animated picture. Occupation probability vs. electron energy. The animated parameter is the spin polarization of the electron gas. Occupation probability of a "spin" state by a spin-polarized electron (spin TIA). Occupation probability of a "spin" state by an electron from the group of spin-unpolarized electrons (spin TIS). Occupation probability of a "full" state by a spin-inactive electron (full). Probability that a quantum states is not occupied (empty). |
![]() Additional calculations (comparing to the above case of spin-unpolarized gas):
Additional calculations (comparing to the above case of spin-unpolarized gas): ![]() The scatterings of spin-polarized electrons are taking into account;
The scatterings of spin-polarized electrons are taking into account; ![]() The rate equation for the conversion between spin-polarized and spin-unpolarized electrons are solved.
The rate equation for the conversion between spin-polarized and spin-unpolarized electrons are solved.
![]() Main idea of the calculation:
Main idea of the calculation:![]() Firstly, the scattering probabilities between spin-polarized electrons, spin-unpolarized electrons and spin-inactive electrons are calculated. It gives the ratio between numbers between electrons in each group.
Firstly, the scattering probabilities between spin-polarized electrons, spin-unpolarized electrons and spin-inactive electrons are calculated. It gives the ratio between numbers between electrons in each group.![]() Secondly,conversion between groups due to the spin pumping and the spin relaxation is calculated.
Secondly,conversion between groups due to the spin pumping and the spin relaxation is calculated. ![]() Thirdly,the distribution for each group is calculated from the condition that the energy distribution of all electrons is described by the Fermi-Dirac distribution.
Thirdly,the distribution for each group is calculated from the condition that the energy distribution of all electrons is described by the Fermi-Dirac distribution.
![]() What is calculated below?:
What is calculated below?:![]() Probability FTIA that a quantum state is occupied by one electron or probability, that a conduction electron belong to the group of spin-polarized electrons;
Probability FTIA that a quantum state is occupied by one electron or probability, that a conduction electron belong to the group of spin-polarized electrons; ![]() Probability FTIS that a quantum state is occupied by one electron or probability, that a conduction electron belong to the group of spin-unpolarized electrons;
Probability FTIS that a quantum state is occupied by one electron or probability, that a conduction electron belong to the group of spin-unpolarized electrons; ![]() Probability Ffull that a quantum state is occupied by two electron or probability, that a conduction electron belong to the group of spin-inactive electrons.
Probability Ffull that a quantum state is occupied by two electron or probability, that a conduction electron belong to the group of spin-inactive electrons.
A. Not at all. It is even opposite. For example, the spin-polarized can be scattered into a quantum state of spin-polarized electrons. E.g. if the spins of all spin-polarized electrons are up, a spin-polarized electron can be scattered only into a quantum state where spin-up position is empty. However, in the case of spin-polarized electrons, the spin-up position is occupied and only spin-down position is empty. Therefore, there is no scatterings between spin-polarized electrons.
A. No. The numbers of spin-polarized and spin-unpolarized electrons remain the same. The total spin of whole electron gas remains the same as well.
A. The spin- independent scattering is a scattering inside of the electron gas, which does not change the total spin of the electron gas. Such scattering can be considered as occurring inside the close system of the electron gas. In contrast, a spin-dependent scattering is the interaction of the electron gas with an external spin particle. The spin-dependent scattering may change the total spin of the electron gas. The effect, which occur due to the spin-dependent scatterings, are the Anomalous Hall effect, the Spin Hall effect, the spin relaxation.
rate equations:
![]() Spin-pumping rate:
Spin-pumping rate:
The spin pumping describes the conversion of electrons from the group of the spin-polarized electrons into the group of spin-unpolarized electrons. The conversion rate of the spin-pumping is described as (details are here)
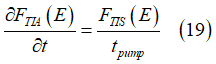
where tpump is the spin pumping time.
![]() Spin-relaxation rate:
Spin-relaxation rate:
Energy distribution of electrons |
||||
|
||||
Probability that a quantum state in electron gas is occupied by an electron from group of spin-polarized electrons (red line) or from group of spin-unpolarized electrons (black line) or from group of spin-inactive electrons (blue line). Click on image to enlarge it |
The spin damping describes the conversion of electrons from the group of the spin-polarized electrons into the group of spin-unpolarized electrons. The conversion rate of spin-relaxation can be described as (details are here)
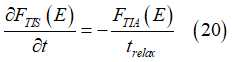
where trelax is the spin relaxation time.
In equilibrium there is a balance between spin relaxation and spin pumping, which gives

Eq.(19e) gives the ratio between distribution FTIA of spin-polarized electrons and the distribution FTIS of spin-unpolarized electrons as
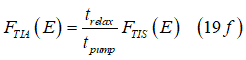
The spin polarization sp of the electron gas is defined as a ratio of the number of spin-polarized electrons to the total number of the spin-polarized and spin-unpolarized electrons. At each electron energy E, the spin polarization can be defined as:

At each energy the spin polarization sp(E) is determined by a balance between the spin pumping and spin relaxation. Substitution of Eq.(19f) into Eq.(20s) gives

Energy distribution of states filled by one or two electrons |
||||
|---|---|---|---|---|
|
||||
Probability that a quantum state that the state is occupied by one electron (spin-polarized + spin-unpolarized electrons) (red line); probability that the state is occupied by two electrons of opposite spins (spin-inactive electrons) (blue line); and their sum (Fermi-Dirac distribution) (black line).click on image to enlarge it. |
Eq.(19e) gives the ratio between distribution FTIA of spin-polarized electrons and the distribution FTIS of spin-unpolarized electrons as

![]() Approximation (soft): It can be assume that sp(E) of Eq.(20e) is energy-independent and equal to the spin polarization sp of the electron gas. Discussion on the validity of this approximation is below. All calculations below are valid also for the case when sp(E) depends on the electron energy E
Approximation (soft): It can be assume that sp(E) of Eq.(20e) is energy-independent and equal to the spin polarization sp of the electron gas. Discussion on the validity of this approximation is below. All calculations below are valid also for the case when sp(E) depends on the electron energy E
A conduction electron can be either in the group of spin-polarized electrons, or group of spin-unpolarized electrons or group of spin-inactive electrons. This condition gives
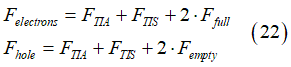
Substituting the Fermi-Dirac distribution (1) and (2) and Eq.(23) into Eq.(22) gives

The ratio between Ffull, Fempty and FTIA , FTIS are found from calculation of scatterings (check on extended menu below). Solution of Eqs.(22e) gives distributions of the spin-polarized FTIA and spin-unpolarized electrons FTIS as
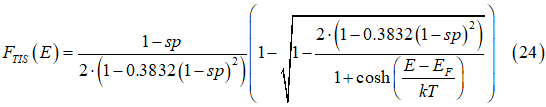
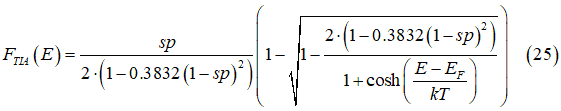
where sp is the spin polarization of the electron gas
![]() The scatterings probabilities between spin-polarized, spin-unpolarized and spin-inactive electrons
The scatterings probabilities between spin-polarized, spin-unpolarized and spin-inactive electrons
![]()
![]() Integrating of possible spin direction of the spin-unpolarized electrons
Integrating of possible spin direction of the spin-unpolarized electrons
Substituting Eq. (23) into Eq. (22) gives

Therefore, the system to be solved consists of Eqs.(17b) and (24.1)

Substituting 2d and 3d equations of (24.2) into the 1st equation gives
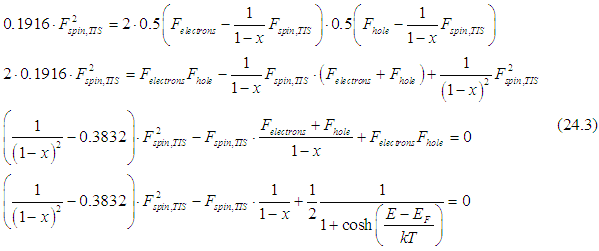
Solving the last Eq. of (24.3) gives
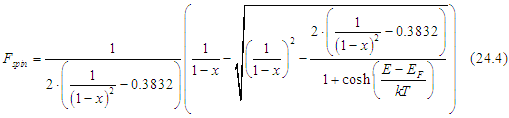
|
|||
| The mean-free path λmean as a function of electron energy E for spin-polarized electrons (black line); spin- unpolarized electrons (red line) and spin-inactive electrons (blue line) See here for calculations of λmean and more details on λmean. click on image to enlarge it |
![]() A. Both the spin pumping and spin relaxation depend on the electron energy. The approximation of these dependencies to the constant average values of the spin pumping and spin relaxation is a zero-order approximation. Taking into account these dependencies are straightforward (See Eqs.(24),(25))
A. Both the spin pumping and spin relaxation depend on the electron energy. The approximation of these dependencies to the constant average values of the spin pumping and spin relaxation is a zero-order approximation. Taking into account these dependencies are straightforward (See Eqs.(24),(25))
Each mechanism of the spin pumping has its own reason for its dependency on E.
![]() Dependency of each mechanism of spin-pumping on the electron energy E:
Dependency of each mechanism of spin-pumping on the electron energy E:
spin-pumping mechanism (1): sp-d exchange interaction. Dependency on electron energy E: moderate.
The strength of the sp-d exchange interaction depends on the overlap of wave functions of a conduction electron and a localized d-electron. When λmean becomes larger, the overlap becomes smaller.
spin-pumping mechanism (2): sp-d scatterings; Dependency on electron energy E:moderate.
The probability of a scattering between of a localized d-electron into a state of conduction electron depends on the overlap of wave functions of a conduction electron and a localized d-electron. When λmean becomes larger, the overlap becomes smaller.
spin-pumping mechanism (3): precession damping in a magnetic field; Dependency on electron energy E:moderate.
The spin damping is not a spin conserving mechanism. The spin damping occurs due to an electron interaction with another spin particle (See here for details). Such interaction depends on the electron size and therefore on λmean.
spin-pumping mechanism (4): spin-dependent scatterings & spin accumulation due to Spin Hall effect; Dependency on electron energy E:moderate.
A spin-dependent scattering depends on which side of scattering center the electron is (See here). When λmean becomes larger, the size of conduction electron becomes larger, the conduction electron overlaps more scattering centers and the spin dependency of the scatterings becomes weaker.
Each mechanism of the spin relaxation has its own reason for its dependency on E.
![]() Dependency of each mechanism of spin-relaxation on the electron energy E:
Dependency of each mechanism of spin-relaxation on the electron energy E:
spin-relaxation mechanism (1): spin-dependent scatterings; Dependency on electron energy E:moderate.
A spin-dependent scattering depends on which side of scattering center the electron is (See here). When λmean becomes larger, the size of conduction electron becomes larger, the conduction electron overlaps more scattering centers and the spin dependency of the scatterings becomes weaker.
spin-relaxation mechanism (2): incoherent spin precession in a spatially inhomogeneous magnetic field: Dependency on electron energy E:moderate.
When λmean becomes larger, the size of conduction electron becomes larger and influence of the spatial inhomogeneities of magnetic field becomes weaker.
spin-relaxation mechanism (3): local fluctuation of magnetization direction:Dependency on electron energy E:moderate.
When λmean becomes larger, the size of conduction electron becomes larger and influence of the local fluctuations of magnetic field becomes weaker.
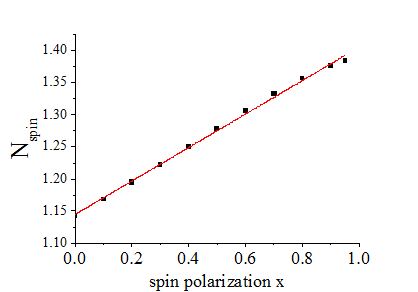 |
Fig 6. The total number Nspin=(nTIA+ nTIS)/D/kT of spin-polarized and spin-unpolarized conduction electrons in a metal as it is calculated in Eq. (62), where nTIA and nTIS is the numbers of spin-polarized and spin-unpolarized electrons and D is the density of states at EFermi |
The integration over all quantum states gives the total numbers of spin-polarized nTIA and spin-unpolarized nTIS electrons as
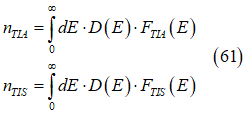
where D(E) is the density of states, FTIA , FTIS are energy distributions of spin polarized and spin-unpolarized electrons (See Eqs. (24),(25))
In the case of a metal, which has a nearly constant density of states near the Fermi energy, the number nTIA of spin-polarized electrons and the number nTIS of spin-unpolarized electrons and the their total number (all conduction electrons except spin-inactive electrons) can calculated as

where D is the density of states at the Fermi energy and sp is the spin polarization of the electron gas
The number of in a metal linearly increases with temperature.
A. It is because only the spin-polarized and spin-unpolarized electrons determine the property of charge and spin transport (See here and here). The contribution of spin-inactive electrons is very weak. The properties of the "full" state is nearly identical to the properties of "empty" states. Both these types of quantum states do not contribute much to the transport.
Approximation of of spin-up/spin-down bands is
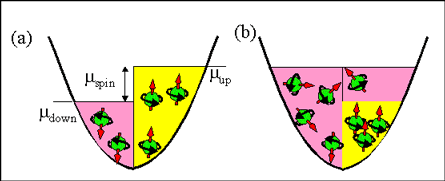 |
Fig 1. Spin accumulation in solid described by (a) model of spin-up/spin-down bands (b) correct description |
The model of spin-up/spin-down bands ignores that the spin rotates during the scattering events N4, N5. It assumes that spin-up and spin-down electrons do not interact (or there is only a very weakly interaction between them). Therefore, the classical model assumes that spin-up and spin-down electrons are staying inside their own bands for a relatively long time.
Since there is no interactions between the bands, the energy distributions of spin-up and spin-down electrons are independent. The distribution of electrons in each band is assumed to be described by its own Fermi-Dirac distribution and the Fermi energy for each band can be different. Thus, the total amount of electrons may not be described by the Fermi-Dirac distribution.
For example, in the case of a spin accumulation, one band (in the case of Fig. 1(a), it is the spin-up band) is filled by a greater number of the electrons. The Fermi energy for the spin-up band is larger than the Fermi energy for the spin-down band and the distribution of all electrons is not the Fermi-Dirac distribution.
All conduction electrons can be divided into the groups of spin-polarized and spin-unpolarized electrons (See Fig. 1 (b)). There is a frequent exchange of electrons between the groups, because of frequent spin-independent scatterings (scattering events N4 and N5). However, the spin-independent scatterings do not change the number of electrons in each group.
Because of the significant exchange of electrons between the groups of spin-polarized and spin-unpolarized electrons, the Fermi energy should be the same for both spin-polarized and spin-unpolarized electrons
In the model spin-up/spin-down bands assumes that there are two independent distributions for spin-up and spin-down electrons.
Both the distribution of spin-up electrons and the distribution of spin-down electrons are described by the independent Fermi-Dirac distribution (See Fig. 1a):
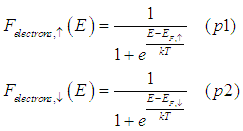
where ![]() are the Fermi energies for the spin-up and spin-down electrons, respectively.
are the Fermi energies for the spin-up and spin-down electrons, respectively.
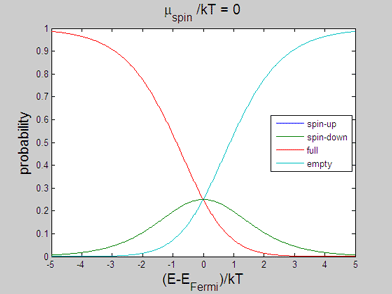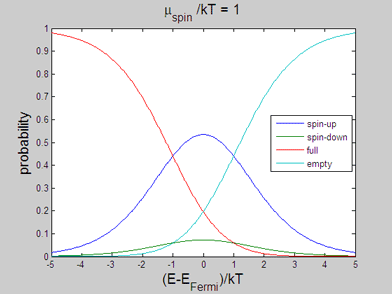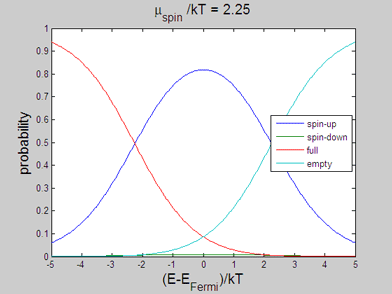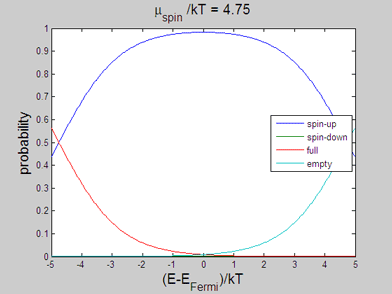 |
Fig 2. Animated graph. Model of spin-up/spin-down bands. The occupation probability of "spin-up", "spin-down", "full" and "empty" states. The animated parameter is the spin chemical potential |
The energy distributions of "spin-up", "spin-down", "full" and "empty" states may be also calculated within the classical model. In the following the spin statistics is described using the classical model of spin-up/spin-down bands. For this purpose the probability of each state is calculated using the assumption that the energy distribution of the electrons of each band is the Fermi-Dirac distribution
The probability of a "spin-up" state, in which only one of the two places is occupied by electrons, will be a product of the probabilities that the spin-up place is occupied and the spin-down place is not occupied
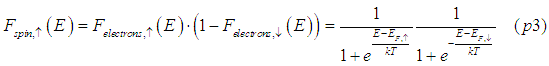
The probability of a "spin-down"state, in which the spin-down place is occupied and the spin-up place is not occupied, will be

The probability of a "full" state, in which both the spin-up and spin-down places are occupied, will be

The probability of a "full" state, in which both the spin-up and spin-down places are occupied, will be
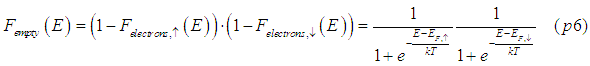
In the classical model, the number of accumulated spins is determined by the spin chemical potential as
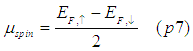
![]() it is the case when energy distribution calculated from the approximation of the spin-up/spin-down bands is correct
it is the case when energy distribution calculated from the approximation of the spin-up/spin-down bands is correct
![]() Even in this case, some spin properties of electron gas are described correctly by the approximation of the spin-up/spin-down bands
Even in this case, some spin properties of electron gas are described correctly by the approximation of the spin-up/spin-down bands
![]() A semiconductor, in which the Fermi energy is in its band gap and does not cross either the conduction or valence band, is called a non-degenerated semiconductor.
A semiconductor, in which the Fermi energy is in its band gap and does not cross either the conduction or valence band, is called a non-degenerated semiconductor.
In the case when electron energy is sufficiently greater than the Fermi energy
![]()
the energy distribution of the spin-polarized and spin-unpolarized electrons (Eqs. (24),(25)) can be simplified as

Using condition (26), Eq. (24) can be simplified as
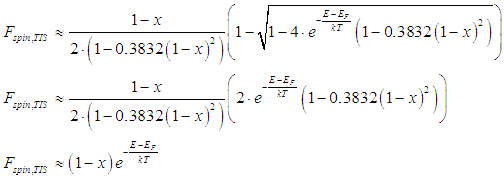
The analysis of the spin transport using the model of spin-up/spin-down bands may be simpler and sometimes more understandable.
Eqs. (27) can be rewritten as

where the effective chemical potentials for spin-polarized and spin-unpolarized electrons are defined as
![]()
In the case of the model of spin-up/spin-down bands, the distribution of electrons in the spin-up and spin-down bands of a non-degenerated semiconductor are described as

The similarities of the distributions (53) and (55) implies that the the spin transport in a non-degenerated semiconductor can be approximated by the classical model of spin-up/spin-down bands by utilizing the individual chemical potential Eqs. (54) for spin-polarized and spin-unpolarized electrons.
It is important to emphasize that the simple form of the distribution (53) leads to a simple description of the spin transport . When the spin direction of the spin-polarized electrons is the same over whole sample, the electron transport of each assembly is independent and it satisfies the Ohm law:

where the conductivities for spin-polarized and spin-unpolarized electrons are linearly proportional to number of electrons in each group of spin-polarized and spin-unpolarized electrons. Therefore, generally they are different.
 or here
or here
I will try to answer your questions as soon as possible