Dr. Vadym Zayets
v.zayets(at)gmail.com
My Research and Inventions
click here to see all content |

Dr. Vadym Zayetsv.zayets(at)gmail.com |
|
 |
more Chapters on this topic:IntroductionScatteringsSpin-polarized/ unpolarized electronsSpin statisticselectron gas in Magnetic FieldFerromagnetic metalsSpin TorqueSpin-Torque CurrentSpin-Transfer TorqueQuantum Nature of SpinQuestions & Answers
more Chapters on this topic:IntroductionScatteringsSpin-polarized/ unpolarized electronsSpin statisticselectron gas in Magnetic FieldFerromagnetic metalsSpin TorqueSpin-Torque CurrentSpin-Transfer TorqueQuantum Nature of SpinQuestions & Answers
more Chapters on this topic:IntroductionScatteringsSpin-polarized/ unpolarized electronsSpin statisticselectron gas in Magnetic FieldFerromagnetic metalsSpin TorqueSpin-Torque CurrentSpin-Transfer TorqueQuantum Nature of SpinQuestions & Answers
more Chapters on this topic:IntroductionScatteringsSpin-polarized/ unpolarized electronsSpin statisticselectron gas in Magnetic FieldFerromagnetic metalsSpin TorqueSpin-Torque CurrentSpin-Transfer TorqueQuantum Nature of SpinQuestions & Answers
more Chapters on this topic:IntroductionTransport Eqs.Spin Proximity/ Spin InjectionSpin DetectionBoltzmann Eqs.Band currentScattering currentMean-free pathCurrent near InterfaceOrdinary Hall effectAnomalous Hall effect, AMR effectSpin-Orbit interactionSpin Hall effectNon-local Spin DetectionLandau -Lifshitz equationExchange interactionsp-d exchange interactionCoercive fieldPerpendicular magnetic anisotropy (PMA)Voltage- controlled magnetism (VCMA effect)All-metal transistorSpin-orbit torque (SO torque)What is a hole?spin polarizationCharge accumulationMgO-based MTJMagneto-opticsSpin vs Orbital momentWhat is the Spin?model comparisonQuestions & AnswersEB nanotechnologyReticle 11
more Chapters on this topic:IntroductionScatteringsSpin-polarized/ unpolarized electronsSpin statisticselectron gas in Magnetic FieldFerromagnetic metalsSpin TorqueSpin-Torque CurrentSpin-Transfer TorqueQuantum Nature of SpinQuestions & Answers
more Chapters on this topic:IntroductionScatteringsSpin-polarized/ unpolarized electronsSpin statisticselectron gas in Magnetic FieldFerromagnetic metalsSpin TorqueSpin-Torque CurrentSpin-Transfer TorqueQuantum Nature of SpinQuestions & Answers
more Chapters on this topic:IntroductionScatteringsSpin-polarized/ unpolarized electronsSpin statisticselectron gas in Magnetic FieldFerromagnetic metalsSpin TorqueSpin-Torque CurrentSpin-Transfer TorqueQuantum Nature of SpinQuestions & Answers
more Chapters on this topic:IntroductionTransport Eqs.Spin Proximity/ Spin InjectionSpin DetectionBoltzmann Eqs.Band currentScattering currentMean-free pathCurrent near InterfaceOrdinary Hall effectAnomalous Hall effect, AMR effectSpin-Orbit interactionSpin Hall effectNon-local Spin DetectionLandau -Lifshitz equationExchange interactionsp-d exchange interactionCoercive fieldPerpendicular magnetic anisotropy (PMA)Voltage- controlled magnetism (VCMA effect)All-metal transistorSpin-orbit torque (SO torque)What is a hole?spin polarizationCharge accumulationMgO-based MTJMagneto-opticsSpin vs Orbital momentWhat is the Spin?model comparisonQuestions & AnswersEB nanotechnologyReticle 11
|
Spin Pumping induced by a Magnetic field
Spin and Charge TransportA magnetic field generates a spin polarization in an electron gas (Spin pumping). The spin polarization of a ferromagnetic metal increases in a magnetic field. An electron gas, which is not spin polarized without magnetic field, becomes spin-polarized when an magnetic field is applied. There is a spin precession around the magnetic field. Due to the precession damping, the spins of spin-unpolarized electrons are aligned along magnetic field. Therefore, the electron gas becomes spin-polarized.
|
| Alignment of spins of conduction electrons along a magnetic field is the reason of increase of the spin polarization of the electron gas | ||||
|---|---|---|---|---|
|
||||
![]() fact 1: In a magnetic field, conduction electrons from the group of the spin-unpolarized electrons are converted into the group of the spin-polarized electrons. The magnetic field induces an additional spin pumping
fact 1: In a magnetic field, conduction electrons from the group of the spin-unpolarized electrons are converted into the group of the spin-polarized electrons. The magnetic field induces an additional spin pumping
![]() Mechanism of the spin pumping in magnetic field:
Mechanism of the spin pumping in magnetic field:
In a magnetic field, the spins of spin-unpolarized electrons aligns along the magnetic field due to the precession damping (See here). However, scatterings quickly re aligns spins of electrons into two groups of spin- polarized (all spins are one direction) and spin-unpolarized electrons (spins are equally distributed in all directions). (details See here) As a result, there are more spin-polarized electrons.
![]() fact 2: Spin polarization of electron gas increases in a magnetic field.
fact 2: Spin polarization of electron gas increases in a magnetic field.
(1) major reason: The magnetic field induces an additional spin pumping; (2) minor reason: The magnetic field partially suppresses (reduces) the spin relaxation.
Spin polarization sp of electron gas increases in a magnetic field and it is calculated as
Measured spin polarization of a ferromagnetic metal in a magnetic field |
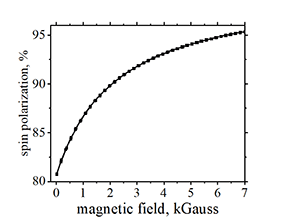 |
| Measured spin polarization of FeB nanomagnet as a function of external magnetic field |
Volt 54B ud40 Ta(2.5)/FeB(1.1)/ MgO(6)/ nanowire width 1000 nm nanomagnet length 500 nm. Fit by Eq.(1.8) gives (region of nanomagnet):sp0=81.2% ; Hpump=0.425 kG ; |
| Click on image to enlarge it. |
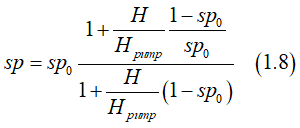
where sp0 is the spin polarization in absence of an external magnetic field (a material parameter), Hpump is the pumping magnetic field (a material parameter)
In a magnetic field, electrons from the group of spin-unpolarized electrons are converted into the group of spin-polarized electrons. This process is called the spin-pumping..
In case of a weak magnetic field when
![]()
the spin-pumping rate can be calculated as
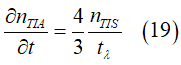
where nTIA and nTIS are the numbers of spin-polarized and spin-unpolarized electrons, respectively , tscattering is the effective time of scattering event 5,
![]()
is the precession damping time, λ is a phenomenological damping parameter of Landau-Lifshitz Equations, g is the g-factor and is μB the Bohr magneton.
In the case of a strong magnetic field when condition (13) is not satisfied, the spin-pumping rate can be calculated as
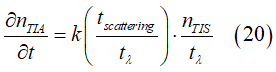
where 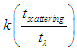 is the coefficient, which depends on the ratio of the scattering time to the spin damping time.
is the coefficient, which depends on the ratio of the scattering time to the spin damping time.
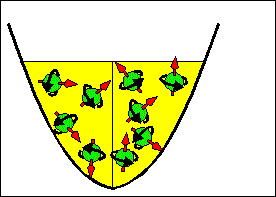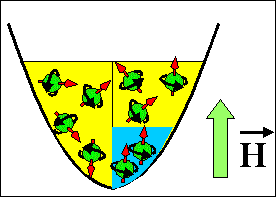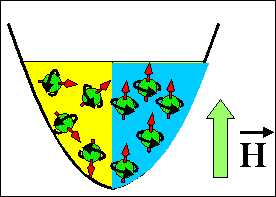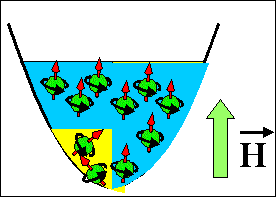 |
Fig.1. Animated picture. Conversion of electrons from group of spin-unpolarized electrons into the group of the spin-polarized electrons under applied magnetic field. The full conversion is only possible when there is no spin relaxation (back conversion) |
Non-magnetic metal
Electron gas is not-spin polarized without magnetic field. When a magnetic field is applied, the electron gas become spin-polarized. Direction of spin polarization is along the magnetic field.
The magnetic filed converts the spin-unpolarized electrons into spin-unpolarized electrons, until this conversion is balanced by the reversed conversion due to spin relaxation mechanisms.
The spin relaxation rate increases when the spin polarization increases. The spin polarization can be found from the condition that rates of the spin pumping and spin relaxation are equal.
|
||
Distribution of spin-directions of electron gas in non-magnetic metal. (left) Without magnetic field. Spins are equally distributed in all directions. All electrons are spin-unpolarized; (right) When magnetic field is applied, spins of some electrons are directed along the magnetic field. The electron gas becomes the spin-polarized. |
Ferromagnetic metal
Electron gas is spin-polarized in a ferromagnetic metal even without an external magnetic field. The sp-d exchange interaction and the intrinsic magnetic field of metal magnetization generate the spin polarization in the electron gas, which spin direction is along the magnetization.
![]() When an external magnetic field is applied along the metal magnetization, the spin polarization becomes larger.
When an external magnetic field is applied along the metal magnetization, the spin polarization becomes larger.
![]() When an external magnetic field is applied at an angle in respect to magnetization direction,
When an external magnetic field is applied at an angle in respect to magnetization direction, ![]() (1) there is a spin precession of spin-polarized electron (2)
(1) there is a spin precession of spin-polarized electron (2) ![]() the magnitude of the spin polarization becomes either larger or smaller depending on the angle of magnetic field..
the magnitude of the spin polarization becomes either larger or smaller depending on the angle of magnetic field..
When the angle between magnetic field and magnetization is:
smaller than 67 degrees -----> spin polarization becomes larger.
larger than 67 degrees -----> spin polarization becomes smaller.
See the interaction of two groups of spin-polarized electrons for more details.
The spin polarization sp of the electron gas is defined as a ratio of the number of spin-polarized electrons to the total number of the spin-polarized and spin-unpolarized electrons (see more here):
where nTIA and nTIS are the numbers of spin-polarized and spin-unpolarized electrons, respectively.
The amounts of spin-polarized and spin-unpolarized electrons is determined by a balance between the spin pumping and the spin relaxation. The spin pumping is the conversion of electrons from groups of spin-unpolarized electrons into the group of the spin-polarized electrons. The spin relaxation is the conversion in the opposite direction.
![]() Spin-relaxation rate:
Spin-relaxation rate:
The spin damping describes the conversion of electrons from the group of the spin-polarized electrons into the group of spin-unpolarized electrons(See here). The conversion rate of spin-relaxation is described as:
where trelax is the spin relaxation time.
![]() Spin-pumping rate:
Spin-pumping rate:
The spin pumping describes the conversion of electrons from the group of the spin-polarized electrons into the group of spin-unpolarized electrons (See here). The conversion rate of the spin-pumping is described as
where tpump is the spin pumping time.
![]() Spin-pumping rate induced by a magnetic field:
Spin-pumping rate induced by a magnetic field:
In a magnetic field, the spins of spin-unpolarized electrons aligns along the magnetic field due to the precession damping (See here). However, scatterings quickly re aligns spins of electrons into two groups of spin- polarized (all spins are one direction) and spin-unpolarized electrons (spins are equally distributed in all directions). (details See here) As a result, there are more spin-polarized electrons. The spin-pumping (See Eq.19 below)
where tH,pump is the spin pumping time in a magnetic field.
In the case that magnetic field is not large, so following condition is satisfied (see below for details)
![]()
The spin pumping time in a magnetic field can be calculated as
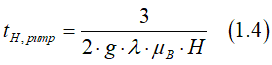
where λ is a phenomenological damping parameter of Landau-Lifshitz Equations, g is the g-factor and is μB the Bohr magneton.
![]() Spin polarization
Spin polarization
Measured spin polarization of a ferromagnetic metal in a magnetic field |
 |
| Measured spin polarization of FeB nanomagnet as a function of external magnetic field |
Volt 54B ud40 Ta(2.5)/FeB(1.1)/ MgO(6)/ nanowire width 1000 nm nanomagnet length 500 nm. Fit by Eq.(1.8) gives (region of nanomagnet):sp0=81.2% ; Hpump=0.425 kG ; |
| Click on image to enlarge it. |
The spin polarization sp of electron gas can be found from the condition that in an equilibrium there is a balance between the spin pumping and the spin relaxation, which is described from Eqs (1.2),(1.5) (1.6) by the condition:
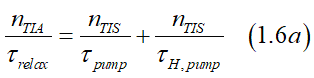
Substitution of Eq.(1.4) in Eq. (1.6a) gives
where Hpump is the pumping magnetic field (a material parameter), which is calculated as
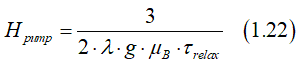
Substitution of Eq.(1.1) into Eq.(1.21) gives the spin polarization sp of electron gas in a magnetic field as
where sp0 is the spin polarization in absence of an external magnetic field (a material parameter) , which can be calculated as
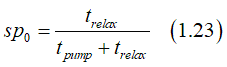
![]() (step 1): The Landau-Lifshitz Equations are solved to find the angle, at which the electron spin turns over time t toward the magnetic field due to the precession damping.
(step 1): The Landau-Lifshitz Equations are solved to find the angle, at which the electron spin turns over time t toward the magnetic field due to the precession damping.
![]() (step 2): The modification of the distribution of spin directions in the group of spin-unpolarized electrons due precession damping are calculated.
(step 2): The modification of the distribution of spin directions in the group of spin-unpolarized electrons due precession damping are calculated.
![]() (step 3): Spin pumping rate is calculated. The modified distribution are decomposed into a sum of the distribution of spin-polarized electrons (all spins are in one direction) and the distribution of spin-unpolarized electrons (spins are equally distributed in all directions). Note, the electron scatterings quickly (near immediately) re distribute electrons of any spin distribution into two group of spin polarized and spin-unpolarized electrons (details See here).
(step 3): Spin pumping rate is calculated. The modified distribution are decomposed into a sum of the distribution of spin-polarized electrons (all spins are in one direction) and the distribution of spin-unpolarized electrons (spins are equally distributed in all directions). Note, the electron scatterings quickly (near immediately) re distribute electrons of any spin distribution into two group of spin polarized and spin-unpolarized electrons (details See here).
No. the spin precession itself does not induce any spin pumping. Only the precession damping induces the spin pumping.
Both the spin-polarized and spin-unpolarized electrons precess in the magnetic field. The precession itself does not affect the time inverse symmetry of both groups of electrons and it does not induce any spin pumping ( the conversion from group of spin-unpolarized electrons into the group of spin-polarized electron).
|
||||
Fig. 2. Spin distribution of electron gas in a ferrimagnetic metal. (a) Without magnetic field. (b) Magnetic field is applied along the spin direction of spin-polarized electrons. (c) Magnetic field is applied opposite to the spin direction of spin-polarized electrons. (d) Magnetic field is applied perpendicularly to the spin direction of spin-polarized electrons. |
During the spin precession, the direction of spin slowly turns in the direction of the magnetic field. This effect is called the precession damping and there are many physical reasons for it (some of them are described here).
The spin precession and the precession damping are described by the Landau-Lifshiz equation
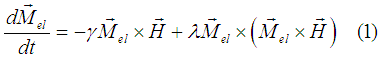
where gamma is the electron gyromagnetic ratio, lambda is a phenomenological damping parameter, ![]() is the magnetic moment of an electron, g is the g-factor and
is the magnetic moment of an electron, g is the g-factor and ![]() is the Bohr magneton. Eq. (1) can be simplify to
is the Bohr magneton. Eq. (1) can be simplify to
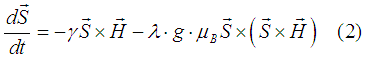
In the case when a magnetic field is applied along the z-axis, a solution of the Landau-Lifshiz equation for the z- component of a vector directed along the direction of electron spin is
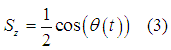
 |
Fig.3 Animated picture. Precession of electron spin in a magnetic field. The precession damping is the reason of conversion of electrons from the group of spin-unpolarized electrons into the group of spin-unpolarized electrons (spin pumping). The red arrow represents electron spin. The grey arrow shows the direction of magnetic field. The data was calculated by solving Landau-Lifshiz equation Eq. (1) |
where theta is the angle between the direction of the magnetic field and the spin direction. Theta can be found from the differential equation:
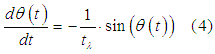
where ![]() is the precession damping time.
is the precession damping time.
The solution of Eq.(4) is
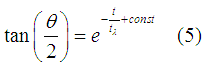
Eq. (5) describes the rotation of the spin towards the direction of a magnetic field. When ![]() , the direction of spin approaches the direction of the magnetic field (The magnetic moment of electron will be opposite to the magnetic field). If at time t0 the angle between spin and magnetic field is theta0, Eq. (5) will be
, the direction of spin approaches the direction of the magnetic field (The magnetic moment of electron will be opposite to the magnetic field). If at time t0 the angle between spin and magnetic field is theta0, Eq. (5) will be
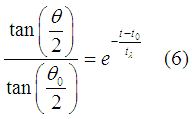
Noticing that
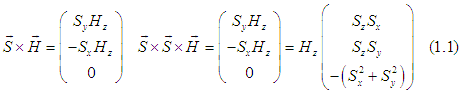
and introducing new unknowns
![]()
Eq. (1) is simplified to
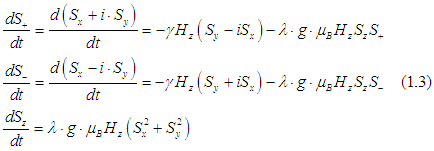
or using definition (5a)
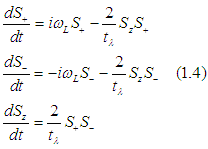
where![]() is the Larmor frequency. A solution of Eqs. (1.4) can be found as
is the Larmor frequency. A solution of Eqs. (1.4) can be found as
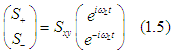
where Sxy is the projection of the spin vector onto the xy-plane. Solution (1.5) describes the precession of the spin in the xy-plane with the Larmor frequency. It can be noticed that the Larmor frequency does not depend on the angle between the spin vector and the magnetic field. Substituting Eqs.(1.5) into Eqs.(1.4) gives
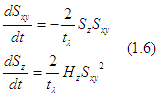
The solution of Eqs. (1.6) can be found as
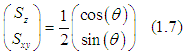
where theta is the angle between the spin direction and the z-axis. S is the magnitude of the spin vector, which equals ½. Theta is an unknown function of time. Substituting (1.7) into (1.6) gives
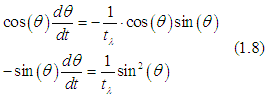
Two equations of (1.8) are the identical and they can be express as
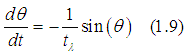
Integration of Eq. (1.9) gives
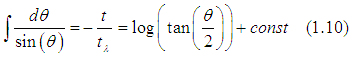
the solution of Eq. (1.10) is
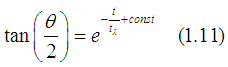
A magnetic field converts the electrons from the group of spin-polarized electrons (group is time-inverse symmetrical (TIS) and its total spin is zero) into the group of the spin- polarized electrons (group is time-inverse asymmetrical (TIA) and its total spin is proportional to the number of electron in this group) . The conversion occurs due to the precession damping. In the group of spin-unpolarized electrons, the spins are distributed equally in all directions. However, the precession damping aligns the spins along the direction of the magnetic field (see Fig.4) and the distribution becomes different.
In the following the temporal evolution of spin directions in the group of the spin-unpolarized electrons are calculated. At this step, the scatterings are not included into the calculations
After some time, the spins of all spin-unpolarized electron would align along the magnetic field and the spin polarization of the electron gas would become 100%.
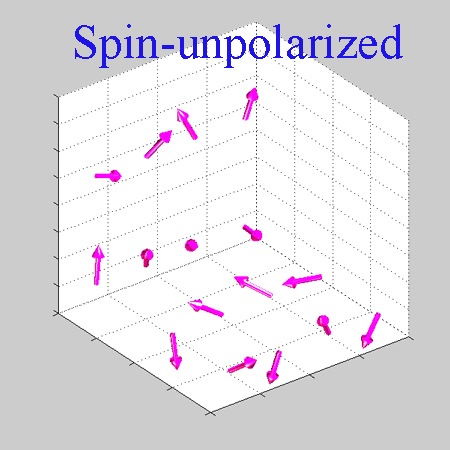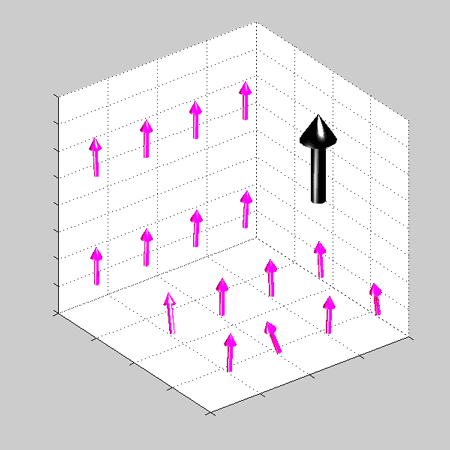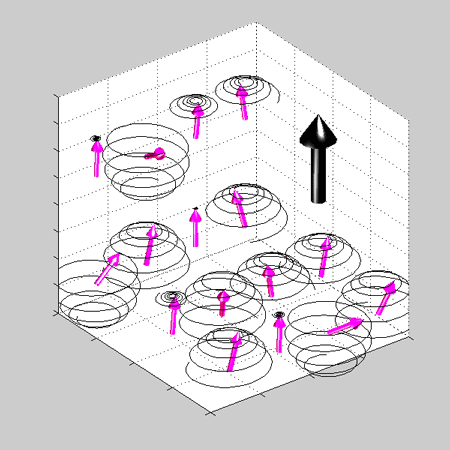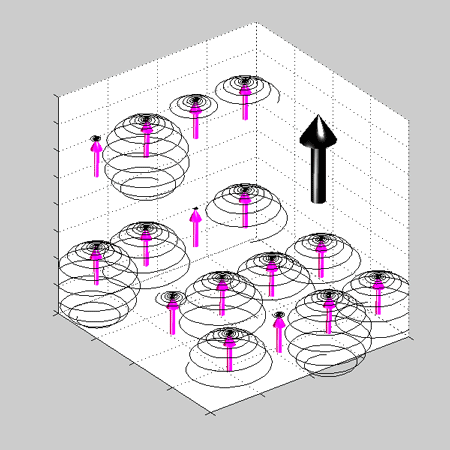 |
Fig.4. Animated picture. The temporal evolution of the angular spin distribution of the group of spin-unpolarized electrons in a magnetic field. Red arrows represents the electron spin . The black arrow shows the direction of the magnetic field. In absence of the magnetic field the spin directions are equally distributed in all directions. Under magnetic field there is a spin precession. Because of the precession damping, spins are aligned along the magnetic field. The dynamic of each spin was calculated by solving Landau-Lifshiz equation Eq. (1). This is the case of no scatterings and no spin relaxation. In an imaginary case without spin relaxation, the electron gas become 100 % spin polarized in a magnetic field. |
In a magnetic field, spins precess around the direction of the magnetic field. Also, spins turn toward the direction of the magnetic field with the angular rotation speed Vθ (See Eq (4))
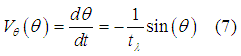
where θ is the angle of the spin direction with respect to the z-axis and t λ is the effective damping time for spin precession (Eq. 5a)
It is assumed that the magnetic field is applied along the z-axis. The angular distribution of spin direction![]() describes the probabilities for a “spin” state to have the spin direction in the interval between θ and θ+dθ with respect to the z-axis.
describes the probabilities for a “spin” state to have the spin direction in the interval between θ and θ+dθ with respect to the z-axis.
Assuming that we known the angular distribution of the spin directions ![]() at time t, it is possible to calculate the distribution at time t+dt. For example, if some states had a spin angle in the interval
at time t, it is possible to calculate the distribution at time t+dt. For example, if some states had a spin angle in the interval ![]() at time t, due to precession damping their spins turn toward the z-axis and at time t+dt their spin angle will be in the interval
at time t, due to precession damping their spins turn toward the z-axis and at time t+dt their spin angle will be in the interval ![]() . Assuming that time dt is sufficiently short, theta1 and d_theta1 can be calculated as
. Assuming that time dt is sufficiently short, theta1 and d_theta1 can be calculated as
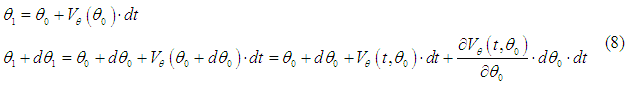
The precession damping does not change the number of spins, it only changes their directions. Therefore, the number of “spin” states is the same in the interval ![]() as in the interval
as in the interval ![]() . Using this fact and Eq (8), the angular distribution of spin direction at time t+dt is calculated as
. Using this fact and Eq (8), the angular distribution of spin direction at time t+dt is calculated as
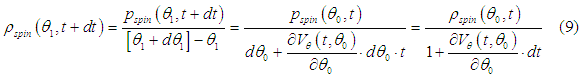
where ![]() is the probability for a “spin” state to have spin direction in the interval
is the probability for a “spin” state to have spin direction in the interval![]() and
and 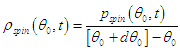 is the angular spin distribution at time t
is the angular spin distribution at time t
|
|||
Fig.5. Animated pictures. Temporal evolution the angular spin distribution as it is calculated from Eq.(11). |
Substituting Eq.(8) into Eq.(9) gives
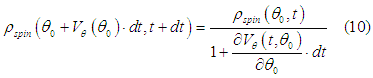
Substituting Eq (7) into Eq. (10) gives the equation, which describes the temporal evolution of the angular distribution of spin directions as
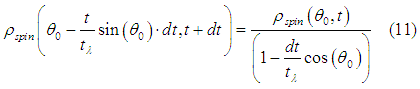
For example, a 1D spring extends so that each point of the spring has different extension speed. In this task it is necessary to find the density of the spring as a function of time.
At time t, the density of the spring at point x0 can be calculated as
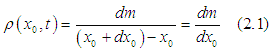
where dm is the spring mass of the spring between points ![]() . Each point of the spring is moving with different speeds V(x), after a short time delta_t the position of points
. Each point of the spring is moving with different speeds V(x), after a short time delta_t the position of points ![]() will be
will be ![]() , where
, where
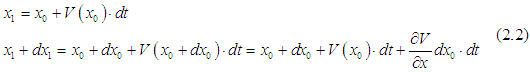
The amount of spring mass between points ![]() at time t is same as the amount of spring mass between points
at time t is same as the amount of spring mass between points![]() at time t+dt, because of the conservation of the spring mass. However, the distance between the points does change. Therefore the spring density at time t+dt can be calculated as
at time t+dt, because of the conservation of the spring mass. However, the distance between the points does change. Therefore the spring density at time t+dt can be calculated as
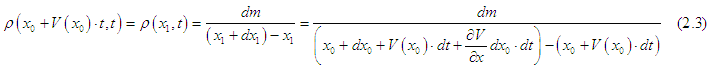
Substituting Eq. (2.1) into Eq. (2.3) gives the equation, which describes the spring density at time t+dt
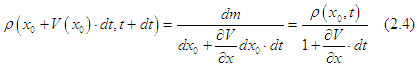
In order to solve Eq. (11), an initial condition should be used. For example, let us assume that at time t=0 a magnetic field has been applied, and the spins starts to rotate toward the magnetic field. At the time t=0 before the magnetic field is applied. Therefore, at time t=0, in the group of spin-unpolarized electrons the spins are distributed equally in any direction. This conditions gives the angular spin distribution at time t=0 as
![]()
Figure 5 shows the time evolution of the spin angular distribution obtained by solving numerically Eq (11) using the initial condition (12). For time longer than![]() , spins are mainly directed along the magnetic field. After an even longer time, eventually all spins will be aligned along the magnetic field (See Fig.4). This could only happen in the case when there are no scatterings between electron states.
, spins are mainly directed along the magnetic field. After an even longer time, eventually all spins will be aligned along the magnetic field (See Fig.4). This could only happen in the case when there are no scatterings between electron states.
A. Frequent electron scatterings prevent such alignment. In fact, the spins are trying to align themselves along the magnetic field, but they are scattered back. However, after such scatterings some electrons become spin-polarized.
The scattering event 5 always converts electrons of any spin distribution into two groups of spin-polarized and spin-unpolarized electrons. Therefore, the scattering event 5 does not give a sufficient time to spin-unpolarized electrons to turn their spins fully toward the magnetic field. Even after a slight rotation toward the magnetic field, the electrons are scattered back into two groups of spin-polarized and spin-unpolarized electrons. Most of electrons remain spin-unpolarized and spins of these electrons are distributed equally in all directions. A small amount of electrons, which represent "the excess of spins” in one direction towards the magnetic field, is converted into the group of the spin-polarized electrons.
 |
Fig.6. Animated picture. The electron conversion from group of spin-unpolarized electrons into the group of spin-polarized electrons. The yellow sphere represents the distribution of spin directions in the group of the spin-unpolarized electrons. The blue surface represents distribution of spin directions in the group of the spin-polarized electrons. The length of a vector directed from center to surface is proportional to the number of electrons, which spin is this direction. Red arrows shows the direction of the magnetic field. |
Schematically, this process of the conversion from the spin-unpolarized electrons into the spin-polarized electrons is shown in Fig.6. Even “spin” states experience the precession damping and the scattering event 5 simultaneously, for clearer understanding these two processes are shown separately in time.
Explanation of Fig.6.
Before the magnetic field has been applied, all spins of all spin-unpolarized electrons were distributed equally in all directions (represented by the yellow sphere). After the magnetic field has been applied, spins start to turn towards the direction of the magnetic field, which corresponds to the elongation of the yellow sphere along the magnetic field. The scattering event converts the spin distribution back into the distributions of spin-polarized and spin-unpolarized electrons. As the result of such conversion, some electrons remain with spin directed along magnetic field (shown by the blue surface) and therefore they become spin polarized. Since some electrons were converted to be spin-polarized, the number of spin-unpolarized electron decreases and therefore the radius of the yellow sphere decreases as well.
How the calculations are done ?
1. At previous step 2, we have calculated how an applied magnetic field modifies the distribution of spin directions of spin-unpolarized electrons
2. In the following, the modified distribution are decomposed into a sum of the distribution of spin-polarized electrons (all spins are in one direction) and the distribution of spin-unpolarized electrons(spins are equally distributed in all directions). It gives the conversion rate of spin-unpolarized to spin -polarized electrons or the spin pumping rate
The scattering event 5 is rather frequent. The precession damping time ![]() is inversely proportional to the intensity of the magnetic field. In the case of a small or moderate magnetic field,
is inversely proportional to the intensity of the magnetic field. In the case of a small or moderate magnetic field, ![]() should be significantly longer than the scattering time
should be significantly longer than the scattering time ![]()
![]()
This means that the rotation angle of spins towards the magnetic field is very small between scatterings. In this case it is possible to solve analytically Eq. (11) and to find the conversion rate.
Using condition (13), Eq. (11) is simplified to
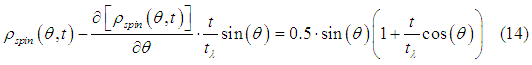
The angular spin distribution can be expressed as
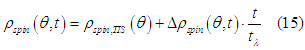
where ![]() is the angular spin distribution of the group of the spin-unpolarized electrons (Eq. (12)) and
is the angular spin distribution of the group of the spin-unpolarized electrons (Eq. (12)) and
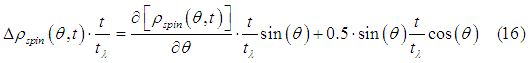
For time significantly shorter than ![]() ,
, ![]() can be used in Eq. (16) instead of
can be used in Eq. (16) instead of ![]() (See Eq. (15)). Using Eq. (12), Eq. (16) can be further simplified to
(See Eq. (15)). Using Eq. (12), Eq. (16) can be further simplified to
![]()
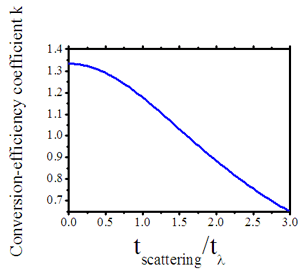 |
Fig.7 Conversion-efficiency coefficient k (See Eq.20) as function of a ratio of the scattering time to the precession damping time Eq. (5a). |
The scatterings quickly convert any inhomogeneous distribution of “spin” states into one distribution of the spin-polarized electrons and one distribution of the spin-unpolarized electrons. The probability for “spin” states to be in the group of the spin-polarized electrons can be calculated from Eq. (17) using Eq. (60) from here as
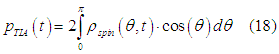
Substituting Eqs. (16),(17) into (18), the integration gives the spin pumping (in case of scattering time significantly shorter than ![]() ) as
) as
where nTIA and nTIS are the numbers of spin-polarized and spin-unpolarized electrons, respectively , tscattering is the effective time of scattering event 5,
The spin damping time ![]() decreases when the intensity of the magnetic field increases. In the case of a large magnetic field, the condition (13) may be not satisfied, in this case the conversion rate can be calculated as
decreases when the intensity of the magnetic field increases. In the case of a large magnetic field, the condition (13) may be not satisfied, in this case the conversion rate can be calculated as
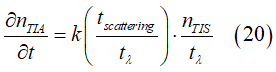
where is 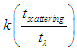 is the conversion-efficiency coefficient, which depends on the ratio of the scattering time to the spin damping time.
is the conversion-efficiency coefficient, which depends on the ratio of the scattering time to the spin damping time.
Figure 7 shows the conversion-efficiency coefficient k ( See Eq (20)) , which was evaluated from a numerical solution of Eq.(11). The conversion-efficiency coefficient k becomes smaller than 4/3 for a stronger magnetic field..
![]() The spin polarization increases when the magnetic field field increases!
The spin polarization increases when the magnetic field field increases!
 or here
or here
![]() Possible confusion!!: from 2014 to 2017 I have used names TIA and TIS for groups of spin-polarized and spin-unpolarized electrons, respectively. The reasons are explained here.
Possible confusion!!: from 2014 to 2017 I have used names TIA and TIS for groups of spin-polarized and spin-unpolarized electrons, respectively. The reasons are explained here.
I will try to answer your questions as soon as possible