Dr. Vadym Zayets
v.zayets(at)gmail.com
My Research and Inventions
click here to see all content |

Dr. Vadym Zayetsv.zayets(at)gmail.com |
|
 |
more Chapters on this topic:IntroductionTransport Eqs.Spin Proximity/ Spin InjectionSpin DetectionBoltzmann Eqs.Band currentScattering currentMean-free pathCurrent near InterfaceOrdinary Hall effectAnomalous Hall effect, AMR effectSpin-Orbit interactionSpin Hall effectNon-local Spin DetectionLandau -Lifshitz equationExchange interactionsp-d exchange interactionCoercive fieldPerpendicular magnetic anisotropy (PMA)Voltage- controlled magnetism (VCMA effect)All-metal transistorSpin-orbit torque (SO torque)What is a hole?spin polarizationCharge accumulationMgO-based MTJMagneto-opticsSpin vs Orbital momentWhat is the Spin?model comparisonQuestions & AnswersEB nanotechnologyReticle 11
|
Spin Precession. Precession Damping. Landau-Lifshitz Equations Spin and Charge TransportThe Landau-Lifshitz Equation describes the precession of the magnetic moment around a magnetic field and alignment of the spin along the magnetic field (spin damping)
|
Electron in an Electrical field In an electrical field an electron accelerates along the electrical field. The electric field interacts with electron charge, but not spin.The interaction is described by the Newton's second laws of motion and the Coulomb's law click here to enlarge |
Electron in a Magnetic Field In a magnetic field, there is a precession of the electron spin (the electron magnetic moment) around the magnetic field. The magnetic field interacts with electron spin (magnetic moment), but not charge.The interaction is described by the Landau -Lifshitz equation click here to enlarge |
 |
It should be noted that due the relativistic nature of the electromagnetic field when an electron moves
in a static electrical field it experiences an effective magnetic field. (See here)
in a static magnetic field it experiences an effective electrical field. (See here)
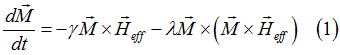
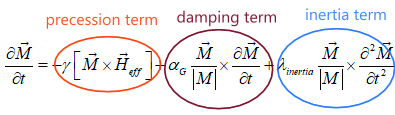
where γ is is the electron gyromagnetic ratio, M is magnetization and Heff is the total magnetic field, which includes the external magnetic field, demagnetization field and effective magnetic field of spin-orbit interaction; λ is the damping coefficient.
The Landau–Lifshitz–Gilbert equation is similar, but it describes differently the damping term:
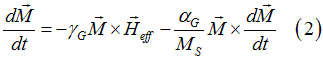
A. Both.
The Landau-Lifshitz Equation describes the Larmor precession , which is the classical effect.
Also, The Landau-Lifshitz Equation describes oscillations between two wavefunction of the spinor, which is a Quantum-Mechanical effect and is a general feature of the broken time-inverse symmetry.
![]() note: Landau-Lifshitz Equation describes two very different processes:
note: Landau-Lifshitz Equation describes two very different processes:
(1) the first term describes spin precession. It is a basic quantum mechanical properties of the spin (the time-inverse symmetry). It a quantum state, in which the electron spin is between its two equilibrium states: (equilibrium state 1) a lower- energy state, in which spin is along the external magnetic field and (equilibrium state 2) a higher- energy state, in which spin is opposite to the external magnetic field. The is a spin-conserving effect. As soon as an electron does not interact with another non-zero particle, theoretically the precision can be going forever (See note below). However, the the oscillations of magnetic moment, which are due to the spin precession, causes an emission of a photon and therefore the damping of the spin precession.
(2) the second term describes the spin damping. This is a process of interaction of the electron with a non-zero particle (e.g. a photon, a phonon, another electron, a magnon(spin wave)). The spin damping is a spin- non-conserving process. The electron spin is aligned along one direction and therefore it is changed.
(Part 9b): Spin dynamic: Landau-Lifshitz Eq vs Quantum mechanic |
|---|
| (short content 1:) Landau - Lifshitz (LL) equitations vs. Quantum Mechanical description of spin dynamic |
| (short content 2:) Why the 1st precession term of LL eq. describes breaking of the time-inverse symmetry and why the 2nd precession damping term of LL eq. describes the quantum transition between spin-up and spin-down energy levels. |
| (short content 3:) why the newly-introduced field-like torque and the reasons why it severely violates Laws of Quantum mechanics |
| click on image to play it |
![]() Equation of spin precession
Equation of spin precession
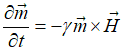
(fact) ![]() The spin precesses around a magnetic field with the Larmor frequency:
The spin precesses around a magnetic field with the Larmor frequency: ![]()
(fact) ![]() The spin precesses counter-clockwise about the direction of the magnetic field.
The spin precesses counter-clockwise about the direction of the magnetic field.
(origin of the spin precession ) 
The spin precession is a quantum-mechanical effect. It describes an electron state when electron energy is between the spin-up and spin-down energy. The wave function of electron during the precession is intermixture of the wave function spin-down and spin-up state.
| Number of spin-up/spin-down electrons vs. precession angle |
|---|
 |
| Fig2 Two representations of the identical spin precession (left) as partially filled spin-up and spin-down energy levels; (right) as magnetization precession around magnetic field H: |
| click on image to enlarge it |

(calculation)![]() Number of spin-up/spin-down electrons vs. precession angle
Number of spin-up/spin-down electrons vs. precession angle
When an electron in a magnetic field, the electron energy is different when the spin direction is along and opposite to the magnetic field H. The energy difference is called the Zeeman energy and calculated as:
![]()
.where g is the g- factor and μB is the Bohr magneton. The wavefunction of the spin-up and spin-down electrons can be expressed as
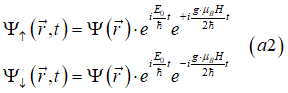
Wavefunctions of (a2) can be expressed using the spinor representation (See here) as
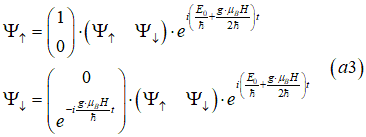
The spinor S of a quantum state, the spin direction of which is describes by angles θ and φ (See Fig) can be described as (See here)
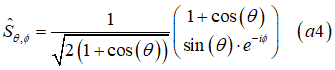
Eq. (a3.) are spinors for for the spin-up state (θ=00 ) and the spin-down state (θ=1800). Its comparison with a general case of Eq.(a4) gives the expression for spinor at an arbitrary angle θ as
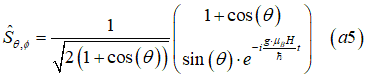
The spinor Eq.(a5) describes a spin precession at precession angle q and with the Larmor frequency ωL:

| Number of spin-up/spin-down electrons vs. precession angle |
|---|
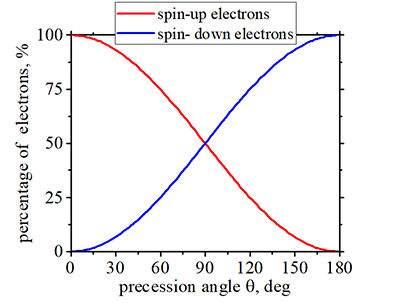 |
| Fig.3 Percentage of spin-up and spin-down electrons as a function of the precession angle |
| click on image to enlarge it |
the wavefunction of the system of electrons with the spin S can be described as a sum of wavefunctions for spin-up and spin-down electrons as
![]()
expression (a6a) corresponds to spinor:
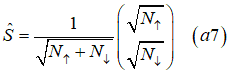
Comparison of Eqs. (a7) and (a5) gives the percentage of filling of the spin-up and spin-down energy level at precession angle θ as
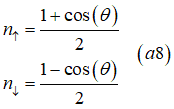


A. No, the spin precession does not violate the spin conservation law. During the spin precession the electron spin does not change.
The electron spin can be either parallel to the applied magnetic field or antiparallel or between these direction. The electron wavefunction for the case when the spin is between parallel and antiparallel directions is a combination of the wavefunction of parallel and antiparallel directions. Such combination describes a state of the spin precession. The states, when electron spin is parallel, antiparallel or at angle to magnetic field and precess, are absolutely equal and describe eigen state of an electron. Even more, it is correct to say that there is a spin precession even for case spin is parallel and antiparallel to the magnetic field, but the precession radius is zero.
(classical (incorrect) view on the spin precession) 
(quantum- mechanical nature of the spin precession)  In the classical case, the magnetic field acts on the magnetic moment of spin and creates torque, which acts on the orbital moment of electron. The torque makes a precession of the orbital moment and therefore a spin precession. Such classical mechanism is also described by the Landau-Lifshitz Equations, but this mechanism is not correct. The spin is fully quantum- mechanical feature and the spin precession is a quantum mechanical process (See details here)
In the classical case, the magnetic field acts on the magnetic moment of spin and creates torque, which acts on the orbital moment of electron. The torque makes a precession of the orbital moment and therefore a spin precession. Such classical mechanism is also described by the Landau-Lifshitz Equations, but this mechanism is not correct. The spin is fully quantum- mechanical feature and the spin precession is a quantum mechanical process (See details here)
 |
 |
Fig.4 Precession of electron spin and the spin precession damping in a magnetic field. The red arrow represents electron spin. The grey arrow shows the direction of magnetic field. The data was calculated by solving Landau-Lifshitz equation (see here) |
Spin precession and precession damping in a magnetic field. During the precession the spin aligns itself along the direction of the magnetic field. Click on the image to enlarge it. |
 The spin damping describes a process of the spin alignment along an external magnetic field.
The spin damping describes a process of the spin alignment along an external magnetic field.
![]() The Equation, which describes the spin damping, (LL equation without spin precession part):
The Equation, which describes the spin damping, (LL equation without spin precession part):
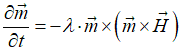
in which the damping coefficient λ depends on the precession angle θ.
(fact) ![]() During the spin damping process the direction of electron spin is changing. During the spin damping, the spin is not conserved!! Another particle with the spin should interact with the electron in order to conserve the spin during spin damping.
During the spin damping process the direction of electron spin is changing. During the spin damping, the spin is not conserved!! Another particle with the spin should interact with the electron in order to conserve the spin during spin damping.
For example, a non-zero-spin particle, which participates in the spin damping, could be a photon (spin=1) or magnon or nuclei with non-zero spin.
Precession Damping |
|---|
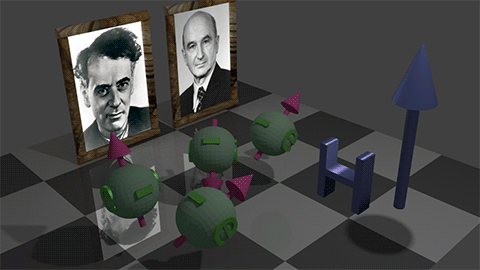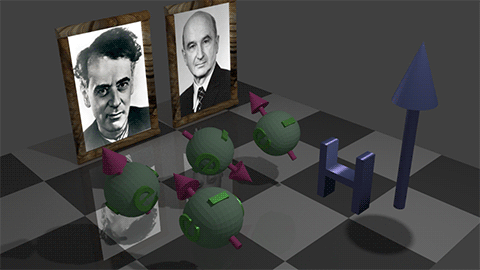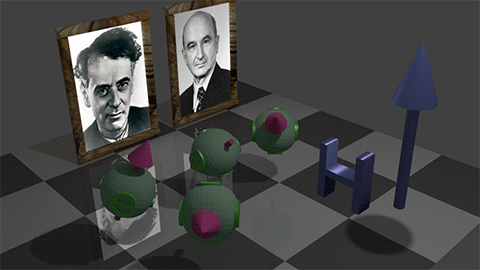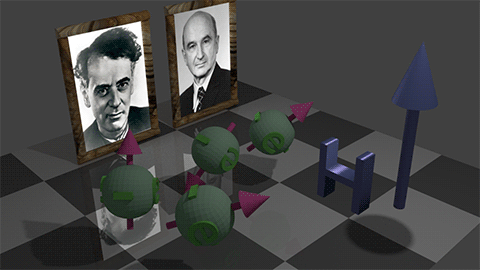
| Fig.5 (spin precession. precession damping) When both the spin-up and spin-down energy are partially occupied, there is a precession of the total spin along the magnetic field. As the electrons from the higher spin-down energy level relax to the lower spin-up level, the precession angle decreases. This process is called the precession damping |
| (initial state) When spins of localized electrons (light-green balls) are opposite to the direction of the external magnetic field H (blue arrow), their energy is larger than when the spin is along the magnetic field. The energy difference between levels is the FMR energy. When all electrons occupy either the lower or upper energy level, there is no spin precession |
| (intermediate state. Spin precession). When some spin-down electrons are relaxed to the lower spin-up energy level and there are occupied states at both energy level. There is a spin precession of the total spin. It is a property of the breaking of the time-inverse symmetry. The precession angle depends on the occupation percentage (See calculations below). The precession angle is 90 degrees when there is an equal number of electrons at each energy level. |
| (spin relaxation. the final spin state). Interaction with environment (non-zero- spin particle) the electron lowers its energy to the lower spin-down energy level and the precession angle decreases until all spins aligned along the external magnetic field H and there is no spin precession. |
| Spin of each individual localized electron(e.g. d-electron) is shown by a light-green ball, The total spin of all localized electrons is shown by the larger dark-green ball. |
| click on image to enlarge it |
For both the localized and conduction electrons the spin damping is collective process, into which simultaneously many electrons are involved.
Localized d-electrons
 All localized electrons are aligned to each other due to the strong exchange interaction. The spins of these electrons are spatially localized to the size of about one atom. As a result, the spins of neighbor electrons swing with respect to each other (similarly as balls connected by springs). E.g. the average swinging angle between to neighbor spins in Ni is 20 degrees at room temperature. (see here). That substantial swinging of all spins with respect to each other is described by an ocean of spin waves and magnons, which are main contributors to the spin damping for localized electrons.
All localized electrons are aligned to each other due to the strong exchange interaction. The spins of these electrons are spatially localized to the size of about one atom. As a result, the spins of neighbor electrons swing with respect to each other (similarly as balls connected by springs). E.g. the average swinging angle between to neighbor spins in Ni is 20 degrees at room temperature. (see here). That substantial swinging of all spins with respect to each other is described by an ocean of spin waves and magnons, which are main contributors to the spin damping for localized electrons.(1) emitting of a circularly- polarized photon; (See here)
(2) interaction with magnons (spin waves)
Conduction sp-electrons
(note)  All localized
All localized
(1) emitting of a circularly- polarized photon (See here);
(2) dephasing of precession;
![]() Why the precession damping is different for the conduction and localized electrons?
Why the precession damping is different for the conduction and localized electrons?
Because of their different probability of scatterings. The conductions electrons are scattered frequently and the scattering of a localized electron is rather a random event. This why the precession damping mechanism are different for those two types of electrons.
When spin-polarized electrons of the opposite spin direction to the magnetization direction of the nanomagnet are injected into the nanomagnet, they induce a magnetization precession in nanomagnet. The precession angle is
Magnetization reversal by spin injectionreversal of spin direction of localized electrons in nanomagnet |
|---|
| Fig.6 When the spin- polarized conduction electrons (small bright-green balls) are injected into a nanomagnet, some of them are scattered into unoccupied spin-down energy level of localized electrons. As a result, there is a precession of the total spin (shown as a light blue ball) of the localized electrons around the internal magnetic field Hint (blue arrow). When the electron number on the upper spin-down energy level becomes equal to the electron number on the lower spin-down level, the magnetization reverses its direction. |
| (prior the spin injection) There is no spin precession, the total spin M is aligned along the internal magnetic field Hint. |
| (spin precession under spin injection) Some injected spin-down conduction electrons are scattered to the unoccupied spin-down states of the localized electrons. As a result, the total spin of the localized electrons (magnetization) starts a precession. The precession is the result of simultaneous occupation spin-up and spin-down states (See above). |
| (decrease of Hint and M under spin injection ) There |
| (magnetization reversal under spin injection) There |
| click on image to enlarge it |
(fact: initial state & internal magnetic field & energy splitting) ![]() In an equilibrium, spins of all localized electrons are aligned along the magnetic easy axis. There is an internal magnetic field Hint in a nanomagnet, which holds the spins along the easy axis. There is an unoccupied higher- energy spin-down state. The difference between energy ΔEFMR=g μB Hint is the Zeeman frequency, which corresponds to the FMR resonance
In an equilibrium, spins of all localized electrons are aligned along the magnetic easy axis. There is an internal magnetic field Hint in a nanomagnet, which holds the spins along the easy axis. There is an unoccupied higher- energy spin-down state. The difference between energy ΔEFMR=g μB Hint is the Zeeman frequency, which corresponds to the FMR resonance
(fact: spin precession) ![]() When simultaneously there are states, which are occupied by a spin-down electron, and states, which are occupied by a spin-up electron, there is spin precession of the total spin. It is feature of a object having broken time-inverse symmetry. The spin describes the breaking of the time- inverse symmetry (See details below)
When simultaneously there are states, which are occupied by a spin-down electron, and states, which are occupied by a spin-up electron, there is spin precession of the total spin. It is feature of a object having broken time-inverse symmetry. The spin describes the breaking of the time- inverse symmetry (See details below)
(fact: reduction of magnetization M under spin injection) ![]() When the spin-polarized electrons of spins of direction opposite to that of the total spin (magnetization), the total spin decreases, because the total spin equals to the difference between spins- up and spins-down
When the spin-polarized electrons of spins of direction opposite to that of the total spin (magnetization), the total spin decreases, because the total spin equals to the difference between spins- up and spins-down
(fact: reduction of the internal magnetic field Hint under spin injection) ![]() Under a spin injection of spins of opposite direction to that of magnetization (the total spin), the magnetization decreases. Since the internal magnetic field Hint is proportional to the magnetization (See here). The Hint decreases following the decrease of the magnetization M.
Under a spin injection of spins of opposite direction to that of magnetization (the total spin), the magnetization decreases. Since the internal magnetic field Hint is proportional to the magnetization (See here). The Hint decreases following the decrease of the magnetization M.
(fact: reversal of direction of the internal magnetic field) ![]() When under the spin injection the number of spin-down localized electrons exceeds the number of spin-up electrons, the magnetization reverses its direction. Following the reversal of M, the internal magnetic field Hint is also reversed.
When under the spin injection the number of spin-down localized electrons exceeds the number of spin-up electrons, the magnetization reverses its direction. Following the reversal of M, the internal magnetic field Hint is also reversed.
(fact: influence of the spin relaxation) ![]() The electrons of the upper- energy level relaxes to the lower- energy level. This process is called the spin relaxation or the precession damping. The larger the split ΔEFMR between the energy levels of the opposite spin, the faster the spin relaxation is. Since split ΔEFMR between decreases when the the internal magnetic field Hint is decreases, the spin relaxation or the precession damping are faster at initial moment of the spin injection and it decreases as the spin precession angle increases.
The electrons of the upper- energy level relaxes to the lower- energy level. This process is called the spin relaxation or the precession damping. The larger the split ΔEFMR between the energy levels of the opposite spin, the faster the spin relaxation is. Since split ΔEFMR between decreases when the the internal magnetic field Hint is decreases, the spin relaxation or the precession damping are faster at initial moment of the spin injection and it decreases as the spin precession angle increases.
(fact: interaction between conduction and localized electrons) ![]() There are continuous scattering between pool of the conduction electrons and the localized electrons. Such a scattering substantially contributes to sp-d exchange interaction (See here)
There are continuous scattering between pool of the conduction electrons and the localized electrons. Such a scattering substantially contributes to sp-d exchange interaction (See here)
Magnetization (total spin), FMR energy splitting (Zeeman splitting), internal magnetic field & precession angle vs spin pumping |
||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
|
||||||||||||||||||
 Q. Why conduction electrons do not support magnons and spin waves?
Q. Why conduction electrons do not support magnons and spin waves?
| only-possible states of spin in a magnetic field | ||||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
|
||||||||||||||||||||||||||
| Fig. 10 click on image to enlarge it |
![]() Note:Usually the spin damping is a long process. It takes many spin-precession periods during the spin damping until the spin is aligned along the magnetic field. The spin damping is the long process because it is not spin- conserved process and it requires the interaction of the electron with another non-zero-spin particle.
Note:Usually the spin damping is a long process. It takes many spin-precession periods during the spin damping until the spin is aligned along the magnetic field. The spin damping is the long process because it is not spin- conserved process and it requires the interaction of the electron with another non-zero-spin particle.
![]() The atomic nucleus very weakly interacts with photons and electrons. As a result, precession damping of nucleus spin is very weak and therefore the peak of the nucleus magnetic resonance (NMR) is very sharp.
The atomic nucleus very weakly interacts with photons and electrons. As a result, precession damping of nucleus spin is very weak and therefore the peak of the nucleus magnetic resonance (NMR) is very sharp.
The reason: The different size. The localized d-electrons have a size about the size of atomic orbital ~ 1 nm. The conduction electrons have a size of ~3-1000 nm.
In case of conductive electrons, the spin damping is the collective process when the different contributions of many conduction electrons causes the spin damping. Many conduction electrons experience the spin damping together at the same.
In case of localized electrons, the spin damping is the individual process when each localized electron experiences the spin damping individually and independently from other localized electrons.
The Landau-Lifshitz (LL) equations without the precession term can be written as
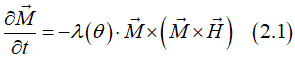
where M is the magnetization, H is total magnetic field applied to the magnetization, which is the sum of external magnetic field and the effective internal magnetic field (See here); λ is damping coefficient, which depends on the precession angle θ.
General solution of Eq.(2.1):
In the case the spin precession around the magnetic field directed in the direction, the damping torque can be calculated as
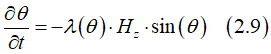
The integration of Eq.(2.9) gives the temporal evolution of the precession angle θ as
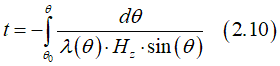
It is the case when a large external magnetic field is applied to a nanomagnet and the the internal magnetic field can be ignored.
The damping torque can be calculated as
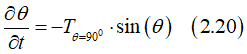
where Hθ=90 is the damping torque at precession angle θ=90 deg
The temporal evolution of the precession angle can be calculated as as
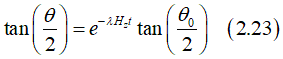
where θ0 is the precession angle at the time moment t=0
It is correct when the precession damping (or pumping) is independent of the precession frequency and of the precession phase. It is often the case.
As a prove, the damping torque, which is found from analytical solutions of LL equations with and without the precession term are identical for many cases (See below)
However, there are cases when the precession damping does depend on the precession frequency and of the precession phase. The parametric damping and pumping are such cases, in which the precession term in the LL equation should not be ignored. (See here and here and here)
 Analytical solution of Landau-Lifshitz Equations
Analytical solution of Landau-Lifshitz Equations
 (Approximation & simplification): precession frequency ω and damping constants λ are constants and independent of the precession angle θ (an incorrect assumption)
(Approximation & simplification): precession frequency ω and damping constants λ are constants and independent of the precession angle θ (an incorrect assumption)
 (Purpose 1) : To demonstrate that a simple analytical solution exists for a simplest case of Landau-Lifshitz Eq.
(Purpose 1) : To demonstrate that a simple analytical solution exists for a simplest case of Landau-Lifshitz Eq.
 (Purpose 2) : To demonstrate that the simplest case when the precession frequency and damping constants are independent of precession angle, gives incorrect results, which do not match to the correct quantum description of the spin precession and which do not fit to the experimental observations.
(Purpose 2) : To demonstrate that the simplest case when the precession frequency and damping constants are independent of precession angle, gives incorrect results, which do not match to the correct quantum description of the spin precession and which do not fit to the experimental observations.
The Landau-Lifshitz (LL) equations can be written as:
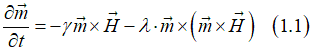
where m is an unit vector directed along the magnetization. |m|=1
 The solution of LL equations (1.1) :
The solution of LL equations (1.1) :
Temporal evolution of magnetization is described as
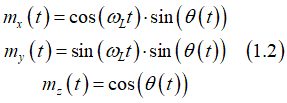
where θ is magnetization angle with respect to direction of the magnetic field H and it is calculated as:
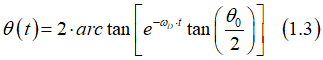
and ![]() is the Larmor frequency,
is the Larmor frequency, ![]() is the damping rate.
is the damping rate.
| Solution of the simplest LL equations | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| (note): This the solution of LL equations under the incorrect assumption that precession frequency ω and damping constants λ are constants and independent of precession angle θ | ||||||||||||
|
||||||||||||
| click on image to enlarge it |
![]() (fact about a solution of the simplest LL equations): The solution of LL equations is divided into two independent solutions. The first solution describes the spin precession. The second solution describes the spin damping
(fact about a solution of the simplest LL equations): The solution of LL equations is divided into two independent solutions. The first solution describes the spin precession. The second solution describes the spin damping
(fact) ![]() The Landau-Lifshitz equations in form of Eq.(1.1) is a very case of a ferromagnet of a spherical shape without any magnetic anisotropy. The most magnetic materials have a magnetic anisotropy. It means there is an axis, which is called the easy axis. When the magnetization is along the easy, the magnetic energy is smallest. There is an intrinsic magnetic field in a nanomagnet, which holds the magnetization along the easy axis. The strength of the intrinsic magnetic field depends on the magnetization angle. The intrinsic magnetic field is largest when the magnetization is along the easy axis and vanishes or becomes smaller, whent the magnetization direction is along the hard axis.
The Landau-Lifshitz equations in form of Eq.(1.1) is a very case of a ferromagnet of a spherical shape without any magnetic anisotropy. The most magnetic materials have a magnetic anisotropy. It means there is an axis, which is called the easy axis. When the magnetization is along the easy, the magnetic energy is smallest. There is an intrinsic magnetic field in a nanomagnet, which holds the magnetization along the easy axis. The strength of the intrinsic magnetic field depends on the magnetization angle. The intrinsic magnetic field is largest when the magnetization is along the easy axis and vanishes or becomes smaller, whent the magnetization direction is along the hard axis.
(fact) ![]() In the most magnetic materials, the PMA is due to the spin-orbit interaction. The feature of the spin-orbit interaction is that it induces magnetic field HSO, which direction is perpendicular to the nanomagnet interface. The HSO is the largest when the magnetization is perpendicular to the interface and it smallest (absent) when the magnetization is perpendicular to interface.
In the most magnetic materials, the PMA is due to the spin-orbit interaction. The feature of the spin-orbit interaction is that it induces magnetic field HSO, which direction is perpendicular to the nanomagnet interface. The HSO is the largest when the magnetization is perpendicular to the interface and it smallest (absent) when the magnetization is perpendicular to interface.
 Dependence of the Larmor frequency ωL and damping frequency ωD on the FMR precession angle
Dependence of the Larmor frequency ωL and damping frequency ωD on the FMR precession angleIn a nanomagnet with the perpendicular magnetic anisotropy (PMA), there is a magnetic field directed perpendicularly to its interface. The magnetic field due to the spin- orbit interaction contributes substantially to this intrinsic magnetic field.
The feature of SO interaction is that the SO dependents substantially on the magnetization direction. As a result, when the magnetization direction turns out of the perpendicularly-to- interface direction, the intrinsic magnetic field decreases. It affects the FMR resonance.
 (fact) As precession angle increases, both the FMR frequency and damping frequency decrease.
(fact) As precession angle increases, both the FMR frequency and damping frequency decrease.
 Precession pumping
Precession pumping 
Precession Pumping |
|---|
|
| Fig. 25 The precession damping as view as energy (left) of the spin-up and spin-down component. |
| click on image to enlarge it |
Emission of electromagnetic waves due to alternating of electrical moment or magnetic moment (fig. 27) |
||||
|
![]() Note: It could be a pumping of the spin precession due to absorption of a photon. The electron magnetic resonance (EMR) and nucleus magnetic resonance (NMR) the nu are based on this effect.
Note: It could be a pumping of the spin precession due to absorption of a photon. The electron magnetic resonance (EMR) and nucleus magnetic resonance (NMR) the nu are based on this effect.
![]() Q. Only circular -polarized wave has spin. Is in EMR and NMR, circular polarized microwave radiation is used.
Q. Only circular -polarized wave has spin. Is in EMR and NMR, circular polarized microwave radiation is used.
A. No. The electromagnetic wave, which are used in the EMR and NMR, is not polarized. The spin absorbed the required polarization. The wave of other polarization remains unabsorbed.
As the Quantum Mechanics predicts, the probability of an interaction between two objects is largest when the objects have similar sizes. The probability of the interaction decreases as the sizes becomes different. In a ferromagnetic material, all spins are glued together very strongly by the strong exchange interaction. The smallest volume of spins, which can be an independent magnetic object is a nucleation domain (see here). The interaction of the spins with magnons or photons is most efficient when the size of the magnons or photons is about the same as the size of the nucleation domain. In the case of a FeCoB nanomagnet, the measured size of the nucleation domain is between 30 nm and 70 nm.
![]() An antenna interacts with an electromagnetic wave most efficiently, when the length of the antenna is a multiple of the wavelength. Similarly, a photon and a magnon with a ferromagnetic material most efficient, when the size of the nucleation domain is a multiple of the wavelength of the photon or the magnon
An antenna interacts with an electromagnetic wave most efficiently, when the length of the antenna is a multiple of the wavelength. Similarly, a photon and a magnon with a ferromagnetic material most efficient, when the size of the nucleation domain is a multiple of the wavelength of the photon or the magnon

Stable Magnetization Precession |
|---|
| Fig. 28 Stable magnetization precession occurs at the precession angle, at which the pumping torque equals to the damping torque |
| The pumping and the damping torques as a function of the precession angle θ. |
| click on image to enlarge it |
(fact) ![]() Stable magnetization precession occurs at precession angle at which the precession damping is equal to the precession pumping.
Stable magnetization precession occurs at precession angle at which the precession damping is equal to the precession pumping.
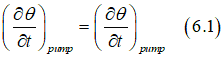
(note) Usually precession damping increases and precession pumping decreases when the precession angle increases. (See here and here)
At a smaller precession angle, the precession pumping exceeds the precession damping and the precession angle increase. However, at a larger precession angle, the precession damping becomes equal to the precession pumping and the magnetization precession is stabilized.
 Magnetization reversal time.
Magnetization reversal time.Condition for magnetization reversal: pumping torque is larger the damping torque at any precession angle |
|---|
| Fig. 29. The pumping and the damping torques as a function of the precession angle θ. At any the damping torque does not exceed the pumping torque. |
| The case of a strong intrinsic magnetic field. The polarity of the damping torque is reversed after =90 deg due the the reversal of the intrinsic magnetic field. |
| click on image to enlarge it |
(fact) ![]() Magnetization reversal occurs, when the precession pumping is larger than the precession damping at any precession angle θ.
Magnetization reversal occurs, when the precession pumping is larger than the precession damping at any precession angle θ.
![]() Condition for the magnetization reversal: precession pumping torque is larger than the precession damping torque torque at any precession angle θ.
Condition for the magnetization reversal: precession pumping torque is larger than the precession damping torque torque at any precession angle θ.
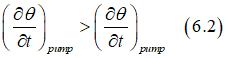
![]() The total torque should be always positive:
The total torque should be always positive:
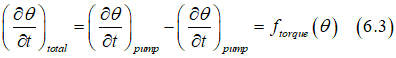
From Eq.(6.3) the time, during which the magnetization is reversed, is calculated as:
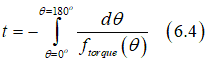
where the initial precession angle θ= 0 deg and at the final (reversed) precession angle is θ= 180 deg.
(fact) ![]() The stronger pumping torque and the weaker damping make the magnetization reversal faster.
The stronger pumping torque and the weaker damping make the magnetization reversal faster.![]()
The quantum-mechanical limitation on possible precession angles:
Spin damping mechanisms:
- emission of a photon
-interaction with a photon
In an atom in a gas, both the spin and electron orbital moment contribute to the atom magnetic moment. In crystal the orbital moment usually is ignored. It is only partially true. There can be a large orbital moment for both the localized and delocalized electrons in a crystal, but interaction of the orbital moment with magnetic field is different in the crystal than in a gas.
1) Orbital moment in a crystal does not precess around a magnetic field
2) There is a difference in energies for orbital moment directed along and in opposite to magnetic field (Zeeman effect).
3) Because of the orbital moment, a magnetic field breaks the time-inverse symmetry for the orbitals. The distribution of the orbitals with the orbital moment along and opposite to the magnetic field are different. Because of the breaking of the time-inverse symmetry, there could be a significant spin-orbit interaction.
Breaking of the time-inverse symmetry in magnetic field due to an orbital moment Due to the Lorentz electrical field the orbital distribution become different for electrons with opposite orbital moments. Red arrow shows direction of the Lorentz force (electrical field). Blue arrow shows the direction of the magnetic field |
||||
|
 The spin and the orbital moment interact differently with a magnetic field
The spin and the orbital moment interact differently with a magnetic field
If the spin interacts only directly with the magnetic field, the orbital moment additionally interacts with relativistic electrical field (Lorentz electric field) induced by the magnetic field.
This field is different for the electrons, which rotate in clockwise and counterclockwise directions. Therefore, the orbital distribution becomes different for two electrons, which rotates in the opposite directions. The time-inverse symmetry is broken !!!
This breaking of the time inverse symmetry may cause a significant enhancement of the magnetic field due to the spin orbit interaction.
Note: For simplicity of understanding, the electron orbit is shown as a 2D circle. The 2D circle can represent a 3D spherical orbit deformed in one direction. This effect exists for any realistic orbit.
Note: Even though the figure shows the classical view of the electron orbit, the quantum mechanical treatment gives exactly the same result.
All electrons, including the inner-orbit electrons and the electrons of an inert gas, experiences.
This effect contributes substantially to diamagnetic properties of gases and solids.
Torque induced by the Lorentz force in a magnetic field for an electron with non-zero orbital moment The electron orbit experiences a torque in a magnetic field applied perpendicularly to the orbital moment. This torque forces the orbital moment to turn to be parallel to the magnetic field. The origin of this torque is the Lorentz force. Red arrow shows direction of the Lorentz force (electrical field). Blue arrow shows the direction of the magnetic fieldclick here to enlarge the image |
In a crystal the electron orbital can not be rotated, even though the electron may experience some orbital torque (See below). Only the electron spin can precess around a magnetic field
This effect also can break the time-inverse symmetry of the orbit.
Direct relation between the shape of the electron orbit and the orbital moment The precession of the orbital moment literally means the precession of electron orbit. Green arrow shows the orbital moment, electron orbit is |
||||
|
Direction and value of orbital moment is directly related to the shape electron orbital. The orbital is asymmetrical in the direction of the orbital moment.
Precession of orbital moment in a magnetic field The precession of the orbital moment literally means the precession of electron orbit. Green arrow shows direction of the orbital moment. Blue arrow shows the direction of the magnetic field |
||||
|
The precession of the orbital moment literally means the precession of electron orbit as well.
The electron
Why there can not be a precession of the orbital moment in a crystal? The precession of the orbital moment literally means the precession of electron orbit. Green arrow shows direction of the orbital moment. Blue arrow shows direction of the magnetic field |
||||
|
The orbital moment of electrons in a solid can not precess around a magnetic field!!!
The non-existing "inertia effect" for spin precession implies acceleration or deceleration of the spin’s precessional motion. The "inertia effect" is based on the incorrect assumption that spin precession is analogous to the precession of a classical object. This effect is typically modeled by introducing an additional term into the Landau–Lifshitz equation. However, such a term contradicts the fundamental quantum-mechanical nature of spin and violates the core principles of time-reversal symmetry. To date, there is no theoretical justification or experimental evidence supporting the existence of an inertial effect in spin precession.
 The core idea of introducing an inertia term into spin dynamics stems from an attempt to draw analogies with classical rotational motion. However, this approach is fundamentally flawed. Spin is an intrinsically quantum-mechanical property, not a classical vector, and there is no classical analog to the quantum spin. In particular, the concept of "spin inertia" contradicts established quantum mechanical understanding: spin does not experience classical inertia. It describes the broken time-reversal symmetry of a particle in a quantum system, and all aspects of spin dynamics are determined by properties of the time-reversal symmetry and by quantum transitions between spin states
The core idea of introducing an inertia term into spin dynamics stems from an attempt to draw analogies with classical rotational motion. However, this approach is fundamentally flawed. Spin is an intrinsically quantum-mechanical property, not a classical vector, and there is no classical analog to the quantum spin. In particular, the concept of "spin inertia" contradicts established quantum mechanical understanding: spin does not experience classical inertia. It describes the broken time-reversal symmetry of a particle in a quantum system, and all aspects of spin dynamics are determined by properties of the time-reversal symmetry and by quantum transitions between spin states
 Incorrect foem of LL equation with inertia term
Incorrect foem of LL equation with inertia term
.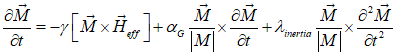
 Incorrect foem of LL equation with inertia term
Incorrect foem of LL equation with inertia term
![]() (Incorrect reason 1): Acceleration of spin precession from a ground state to a precessing state
(Incorrect reason 1): Acceleration of spin precession from a ground state to a precessing state 
One of main arguments for existence of the inertia effect is that spin must be "accelerated" from a ground state (where no precession is visible) to a precessing state, implying an inertial delay. This interpretation is inappropriate.
![]() (correct explaination): From the quantum perspective, spin describes a broken time-reversal symmetry for a particle. In the ground state, the precession angle is zero, making the precession undetectable—but it is still fundamentally present. Excitation to higher-energy states increases this angle, making the precession observable, but the underlying quantum state evolves smoothly, the spin precession exists for both states. There is no need for "acceleration" in the classical sense.
(correct explaination): From the quantum perspective, spin describes a broken time-reversal symmetry for a particle. In the ground state, the precession angle is zero, making the precession undetectable—but it is still fundamentally present. Excitation to higher-energy states increases this angle, making the precession observable, but the underlying quantum state evolves smoothly, the spin precession exists for both states. There is no need for "acceleration" in the classical sense.
![]() (Incorrect reason 2): On Change of Precession Frequency with Increasing Precession Angle
(Incorrect reason 2): On Change of Precession Frequency with Increasing Precession Angle 
Another possible argument for the existence of spin inertia is the experimentally- observed reduction of precession (FMR) frequency with increasing precession angle as if some slowing-down/ accelerating force affects the spin precession. .
![]() (correct explaination): The reason why the spin precession is slowing-down or accelerating with change of the precession angle has a well-understood quantum mechanical origin:
(correct explaination): The reason why the spin precession is slowing-down or accelerating with change of the precession angle has a well-understood quantum mechanical origin:
In absence of an external field, the ferromagnetic resonance (FMR) frequency is determined by the internal magnetic field, which is itself proportional to the difference between numbers of spin-up and spin-down electrons.
As the precession angle increases, more spins are excited to the higher-energy (spin-down) state, reducing imbalance between spin-up and spin-down electrons and thus reducing the internal magnetic field. This results in a lower FMR frequency. This mechanism is purely quantum mechanical and should not be attributed to any inertial effect.
It is well-established in quantum mechanics that spin dynamics are governed by symmetry principles and transitions between quantized states, not by classical inertia. The Landau-Lifshitz (LL) equation correctly describes spin precession as a quantum transition between spin-up and spin-down states. The first term accounts for energy splitting between spin states , while the second term models transition between spin states (spin damping or spinpumping). It is a remarkable coincidence that this classical form captures aspects of both classical and quantum precession, but its success does not justify the addition of arbitrary classical terms.
 Any modification to the LL equation must be derived from quantum mechanical principles—not classical analogies. Introducing terms like inertia, unless rigorously derived from quantum mechanics, misrepresents the physical system.
Any modification to the LL equation must be derived from quantum mechanical principles—not classical analogies. Introducing terms like inertia, unless rigorously derived from quantum mechanics, misrepresents the physical system.The introduction of the inertia effect is a good example when the roles of classical mechanics become ridiculous in quantum mechanics. The usage of the inertia effect in the spin dynamics is fully equivalent as an usage of the inertia for a quantum transition between atomic levels of an atom. It makes no sense at all.
![]() Relation between precession damping and exchange. Spin relaxation: individual or collective?
Relation between precession damping and exchange. Spin relaxation: individual or collective?
![]() ( from Sky) Q. I have some confusion about the precession damping for the localized electrons. There are two statements in this subject: (1)"All localized electrons are aligned to each other due to the strong exchange interaction. The spins of these electrons are spatially localized to the size of about one atom. As a result, the spins of neighbor electrons swing with respect to each other (similarly as balls connected by springs)." and (2) "In case of localized electrons, the spin damping is the individual process when each electron experiences the spin damping individually and independently from other localized electrons." In my opinion, the statement (1) means that the precession damping of localized electrons is strongly connected with each other, which seems contradict with statement (2).
( from Sky) Q. I have some confusion about the precession damping for the localized electrons. There are two statements in this subject: (1)"All localized electrons are aligned to each other due to the strong exchange interaction. The spins of these electrons are spatially localized to the size of about one atom. As a result, the spins of neighbor electrons swing with respect to each other (similarly as balls connected by springs)." and (2) "In case of localized electrons, the spin damping is the individual process when each electron experiences the spin damping individually and independently from other localized electrons." In my opinion, the statement (1) means that the precession damping of localized electrons is strongly connected with each other, which seems contradict with statement (2).
The spin precession and the precession damping are a collective effect, when the directions of all spins are parallel all the time. It is because the exchange interaction between spins is very strong. Exceptions are the spin waves and domain walls. The exchange interaction between localized neighbor electrons is very strong, but is not infinitely strong. As a result, a slight misalignment between neighboring spins are possible (spin waves). Also, when some strength is accumulated over many spins (over millions or billions of spins) the parallel alignment between neighboring spins can be broken (a domain wall).
The spin damping is a collective process of the total spin. There is no individual spin damping. The spin-down to spin-up quantum transition of one electron means only change of one component of the total spin and is not related to individual spin of one localized electron.
Since the exchange interaction is not infinitely strong, a slight misalignment between two localized neighbor electrons is possible. Due to such a tiny misalignment, a spin wave exists in a ferromagnetic material. A spin wave is a mixture of an electromagnetic wave and spin precession. The magnetic component of an electromagnetic wave is slightly different at a position of each localized electron. As a result, the spin precession is slightly different between neighboring localized electrons. As you said, the spins of neighboring electrons slightly swing with respect to each other. Even though the spin misalignment between neighboring electrons is very small and the spins of neighboring electrons are still nearly parallel, the misalignment is accumulated with a distance and can be substantial for the electrons separated by a long distance.
(spin wave as a source of the spin damping)
The spin wave is a particle with a non-zero spin. It interacts with the total spin of the nanomagnet causing an electron transition from the higher- energy spin-down energy level to the lower- energy spin-up energy level. This process is called the spin damping and this quantum transition is fully equivalent to the classical precession damping. It is important that the spin wave interacts with the total spin of the whole nanomagnet, but not with individual spin of localized electrons. The interaction is the most efficient when the size of the nanomagnet or size of a magnetic domain matches the wavelength of the spin wave.
(strength of the exchange interaction)
The strength of the effective exchange magnetic field is possible to estimate from Curie temperature (see my web page on exchange interaction). The magnetic field of the exchange interaction is rather high. It is about 1900 Tesla for Co and 900 Tesla for Ni. For example, a large superconducting magnet produces a magnetic field of about 20-40 Tesla. Because of the high strength of the exchange interaction, it is nearly impossible that the spin of one individual localized electron is reversed with respect to the spin direction of all neighboring electrons. Only many electrons can reverse their spins simultaneously and coherently ( a magnetic domain)
(spin dumping for an individual electron)
All individual localized electrons are so strongly glued to each other by the exchange interaction, they behave as one quantum object. Spin of a localized electron is aligned strongly to be parallel to the spins of its neighboring localized electrons. The total spin behaves as one quantum object. It precesses as one object or tilts its direction as one object and interacts with spin waves (magnons) and circularly- polarized photons as one object.
(spin of one individual electrons vs. the spin as a component of the total spin)
Even when there is a quantum transition of an electron from the spin-down to spin-up energy level (spin damping), it does not mean that one localized electron becomes spin-up in the surroundings of neighboring spin- down electrons. The spins of all neighboring electrons remain parallel (nearly) all the time. The meaning of the transition of one electron from the spin-down to spin-up energy level means that one component of the total spin is changed and, as a result, the precession angle of the total spin becomes larger. All the time the spin of all localized electrons are glued to each other. All spins precess coherently and are always parallel to each other.
(magnetic domain & spin damping)
The strong exchange interaction can be broken at a boundary between magnetic domains. Some effects can accumulate for a larger number of localized electrons. When the number of localized electrons reaches some critical number, a domain wall is formed. The behavior of two neighbor domains may be rather independent. E.g., the magnetic dipole interaction makes magnetization of neighbor domains to be antiparallel. Similarly, the spin precession of the neighbor domains can be at slightly different frequency and the precession angle.
I will try to answer your questions as soon as possible