Dr. Vadym Zayets
v.zayets(at)gmail.com
My Research and Inventions
click here to see all content |

Dr. Vadym Zayetsv.zayets(at)gmail.com |
|
 |
more Chapters on this topic:IntroductionScatteringsSpin-polarized/ unpolarized electronsSpin statisticselectron gas in Magnetic FieldFerromagnetic metalsSpin TorqueSpin-Torque CurrentSpin-Transfer TorqueQuantum Nature of SpinQuestions & Answers
more Chapters on this topic:IntroductionScatteringsSpin-polarized/ unpolarized electronsSpin statisticselectron gas in Magnetic FieldFerromagnetic metalsSpin TorqueSpin-Torque CurrentSpin-Transfer TorqueQuantum Nature of SpinQuestions & Answers
more Chapters on this topic:IntroductionScatteringsSpin-polarized/ unpolarized electronsSpin statisticselectron gas in Magnetic FieldFerromagnetic metalsSpin TorqueSpin-Torque CurrentSpin-Transfer TorqueQuantum Nature of SpinQuestions & Answers
more Chapters on this topic:IntroductionTransport Eqs.Spin Proximity/ Spin InjectionSpin DetectionBoltzmann Eqs.Band currentScattering currentMean-free pathCurrent near InterfaceOrdinary Hall effectAnomalous Hall effect, AMR effectSpin-Orbit interactionSpin Hall effectNon-local Spin DetectionLandau -Lifshitz equationExchange interactionsp-d exchange interactionCoercive fieldPerpendicular magnetic anisotropy (PMA)Voltage- controlled magnetism (VCMA effect)All-metal transistorSpin-orbit torque (SO torque)What is a hole?spin polarizationCharge accumulationMgO-based MTJMagneto-opticsSpin vs Orbital momentWhat is the Spin?model comparisonQuestions & AnswersEB nanotechnologyReticle 11
more Chapters on this topic:IntroductionScatteringsSpin-polarized/ unpolarized electronsSpin statisticselectron gas in Magnetic FieldFerromagnetic metalsSpin TorqueSpin-Torque CurrentSpin-Transfer TorqueQuantum Nature of SpinQuestions & Answers |
Basic properties of electron spin. Spinors.
Magnetism of electron gasAbstract: This page describes the basic properties of the electron spin. It describes what is the spin direction, spin projection and spin precession and how they should be treated in a description of electron gas.
|
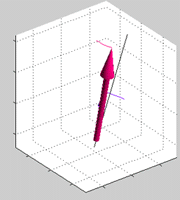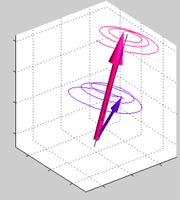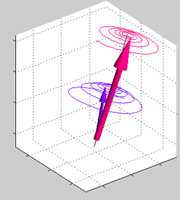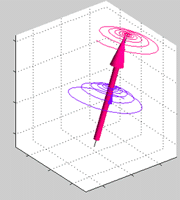 |
Precession of electron spins. |
A. The wavefunction of an electron is a scalar, which describes the probability to find the electron at each point in space. The spinor describes, which scalar wave function the electron has for each direction of its spin. Since the spin direction may change in time, it is convenient to describe an electron by a spinor.
A. The spin is intrinsic feature of an electron. One electron has one spin value and one spin direction over its all spacial size. In a system of several electrons there may be a spacial variation of spin value and spin direction. For example, in an atom the nucleus and electrons have different spin directions and different spin value.
The spin direction of an electron may change in time. The rotation of the spin direction in a magnetic field is called the spin precession.

![]() How to find spinor from a known spin direction. Basic procedure:
How to find spinor from a known spin direction. Basic procedure:
A quantum state of an electron, which spin direction is at angles θ and φ as shown in Fig.5, is described by a spinor S, which is an eigenvector of the following Pauli matrix:
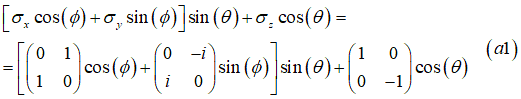
Calculation of the eigenvector of (a1) gives the spinor of an electron, which spin direction is at angles θ and φ as shown in Fig.5, as
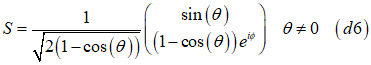
or
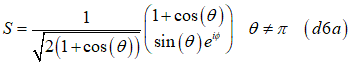
example 1: Spin is in the xy-plane θ= π/2
When the spin direction is in the xy-plane and φ is the angle of spin with respect to the x-axis, the Pauli matrix (a1) becomes
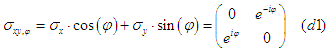
The spinor for the example 1 (eigenvector of (d1) is calculates as
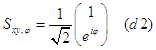
example 2:Spin is in the xz-plane φ= 0
The Pauli matrix (a1) becomes
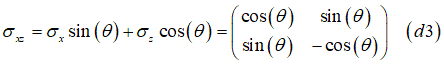
The spinor for the example 2 (eigenvector of (d3) is calculates as
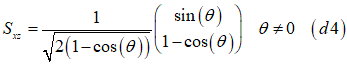
or
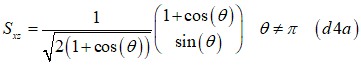
In order to find the wavefunction for any spin direction, it is necessary to to know the wavefunction at least for two directions. For example, if the wavefunction is ![]() , when the spin along the z-axis, and the wavefunction is
, when the spin along the z-axis, and the wavefunction is ![]() , when the spin opposite to the z-axis, the wavefunction for other direction can be calculated as follows:
, when the spin opposite to the z-axis, the wavefunction for other direction can be calculated as follows:
along z- axis ![]()
![]() spinor:
spinor: 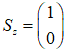
![]() wavefunction:
wavefunction: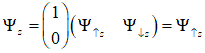
-----
![]() opposite to z- axis
opposite to z- axis
![]() spinor:
spinor: 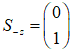
![]() wavefunction:
wavefunction: 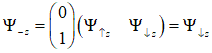
-----
along x- axis ![]()
![]() spinor:
spinor: 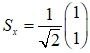
![]() wavefunction:
wavefunction: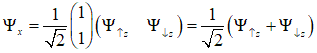
-----
![]() opposite to x- axis
opposite to x- axis
![]() spinor:
spinor: 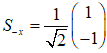
![]() wavefunction:
wavefunction: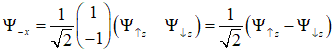
-----

Three Pauli matrixes are only matrixes, which satisfy the following conditions:
(condition 1) ![]() They should be 2x2 matrixes, because there are only two independent wavefunctions, which correspond to the direct and reversed time flow.
They should be 2x2 matrixes, because there are only two independent wavefunctions, which correspond to the direct and reversed time flow.
(condition 2) ![]() They should be an unit matrixes. Obviously, the spin rotation should not change the number of electrons.
They should be an unit matrixes. Obviously, the spin rotation should not change the number of electrons.
(condition 3) ![]() Double rotation over 90 degrees around any axis should reverse the spin or the same should give the time-inverse wavefunction.
Double rotation over 90 degrees around any axis should reverse the spin or the same should give the time-inverse wavefunction.

It is possible to find a projection of spin onto any direction. It is also possible to decompose spin directed in one direction as a sum of spins directed parallel and anti parallel to some other direction (for example, a sum of spin-up and spin-down wavefunctions).
It should be noted that spinors of two spins, which are directed parallel and antiparallel to some axis, are orthogonal. Therefore, their dot product equals zero
![]()
Using the property (p1), a spin can be represented as a sum of two spins of opposite directions as
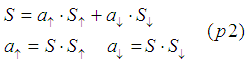
Example 1 Spin directed along the x-axis is decomposed as a sum of opposite spins directed along the z-axis.
It should be noted that this is impossible for a conventional spacial vector. From (2) and (5), we have
![]()
Example 2 spin directed in the xy-plane is decomposed as a sum of opposite spins directed along the x-axis.
Rewriting (d2) and (3)
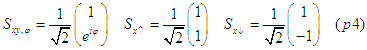
where phi is the angle of spin with respect to the x-axis. The spinor of spin directed in the xy-plane is
![]()
From Eq.(p1)
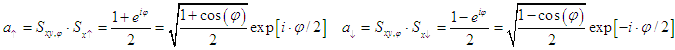
or
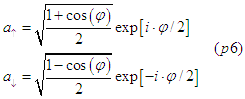
the probability to be in spin up and spin down states will be
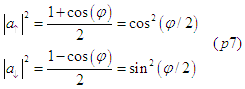
in the case of small phi Eq. (p7) is simplified to
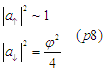
Below it is shown how to describe the Zeeman effect and the Larmor precession of spin in a magnetic field using spinors.
Step 1. The calculation of the spinor for the case when the spin direction is either parallel or antiparallel to the magnetic field.
In a magnetic field the electrons, whose spin directed along and opposite to the magnetic field, have different energy (Zeeman effect). The difference in energy is given as
![]()
where g is the g- factor, 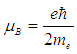 is the Bohr magneton.
is the Bohr magneton.
For example, in the case when a magnetic field H is applied along the z-axis, the electrons with spin directed parallel and anti parallel to the z-axis have energy
![]()
where E0 is the electron energy in the absence of a magnetic field.
From Eq.(21), the wavefunction of spin-down and spin-up electrons can be expressed as
![]()
or
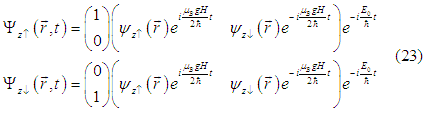
Eqs. (23) describe electron wavefunction for two directions of the spin by taking the projection on the z axis of following spinor:

Step 2. The calculation of the spinor for the case when the spin direction is either parallel or antiparallel to the magnetic field.
The Eqs. (23) describe the case when both the spin direction and the direction of the magnetic field are along the z-axis. When the spin direction turns away from the z-axis through angle theta, the electron wavefunction should still be described by Eq.(23) but using the spinor corresponding to the turned spin. Substituting Eq. (d.4) for the spinor turned by angle theta away from the z-axis into Eq(23) gives
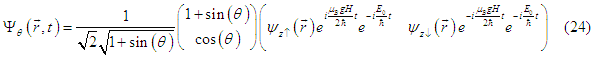
Eq. (24) can be simplified to
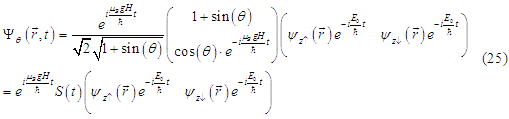
where S(t) is the time-dependent spinor
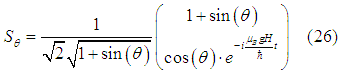
which describes an electron, whose spin direction has time-independent angle theta with respect to the z-axis and the time –dependent angle phi in the xy- plane. Comparing Eqs. (d5) and (26), the angle of spin in the xy-plane with respect to the x-axis is
![]()
Therefore, Eq (25) described a precession of spin around the magnetic field with the Larmor frequency
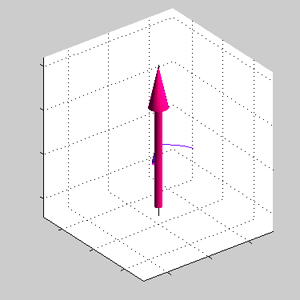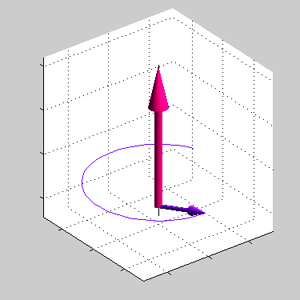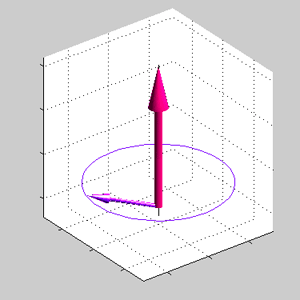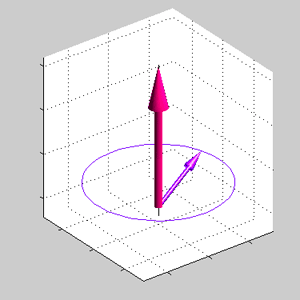 |
Fig 3. Animated figure. Precession of spins of a an conduction electron (blue arrows) due to the exchange interaction with the local d-electrons. The precession axis is along the spin direction of the local d-electrons (red arrow). The angle between spin directions of sp- and d-electrons is 70 deg. |
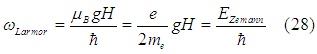
Eq.(24) and Eq (25) are equivalent. In Eq (24) the spinor is time-independent and it describes two electron states of different energy. In contrast, in Eq. (25) the spinor is time-dependent and it describes electron states of the same energy, but with spin precession.
Therefore, there are two fully equivalent representations of spin in a magnetic field. In the first representation the spin state is described as a superposition of two spin states with different energy and opposite time-independent spin-directions. In the second representation the spin state is described as a state with a constant energy, but with time-dependent spinor. It corresponds to the time-dependent spin direction or the spin precession.
The spin precession is not specific for the interaction of spin with magnetic field, but is a general property of spin.
Along any direction (for example, the direction of the magnetic field, spin can have only two projections ½ and -½). In contrast, spin can have any direction in the 3D space. This looks like as two controversial statements and sometimes it confuses the understanding of spin properties.
In fact, this is just two identical representations of a "spin" state in a magnetic field. In the first representation a "spin" state is represented as a sum of two projections with different energies. The energies are different for the projection with spin parallel or anti parallel to the magnetic field. There is a finite probability for a “spin” state to be in a state with either higher energy or lower energy. In the second representation, the “spin” state has a defined energy, but there is spin precession around the magnetic field. It should be noted that the precession frequency (the Larmor frequency) does not depend on the angle between the spin direction and the magnetic field and there is a spin precession even in the case when the spin parallel to the magnetic field.
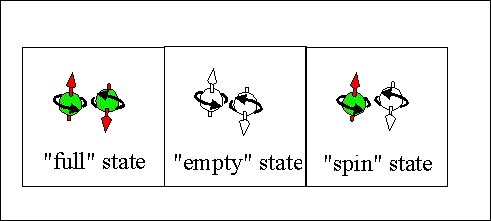 |
Fig 1. Three possibilities of occupation of an electron state. Only a state, which is filled by one electron, has a defined spin direction. For the spin- inactive state (also called "full" state) and the "empty" state, the time-inverse symmetry is not broken. As a result, they both do not have a spin direction. |
1. The conduction electrons can be in "spin", spin- inactive state and "empty" states. Only "spin" states have spin directions. The spin of spin- inactive state and "empty" states is zero and these states do not have a spin direction.
2. There are two electrons of opposite directions in a spin- inactive state, but the information about the spin direction of electrons before been scattered into a "full" state is not memorized in the "full" state. Therefore, the two electrons of opposite spin directions can have spin directed in any direction with equal probability.
3. "spin" states have a spin direction. spin- inactive state and "empty" states do not have a spin direction
4. There are two identical representations of a "spin" state in a magnetic field. In the first representation a "spin" states is represented as a sum of two projections with different energies. The energies are different for the projections with spin parallel are antiparallel to the magnetic field. In the second representation, there is a spin precession around the magnetic field. The energy of the "spin" state is constant.
An electron quantum state can either be filled by no electrons or one electron or two electrons of opposite spins. We define these three states as “full”, “empty” and “spin” states (See Fig.1).
Only the "spin" state can have a spin direction. The spin of "full" and "empty" states is zero and these states do not have a spin direction. This can be easily understood by comparing an electron with a photon. The spin direction can be thought of as analogous to the polarization direction of a photon. The analog of a "spin" state is linearly polarized light. It always has a defined direction of polarization (it could be s- or p- polarized or somewhere in between). The analog of a "full" state is unpolarized light (light of equal p- and s- polarizations). The polarization direction in this case can not be defined (The intensity of light is equal for any polarization direction).
1. "spin" state
"spin" state is an electron state in which one of the two possible places is occupied by an electron. The wavefunction for a "spin" state is
![]()
The time inverse symmetry is broken for a "spin" state. When the time inverse symmetry is broken, there is always a direction along which it is broken. Of course, it is the spin direction.
The "spin" state always has a spin direction along which the time inverse symmetry is broken.
A change of the spin direction can be described by a corresponding change of the coefficients a1 and a2 in the spinor (7).
2. spin- inactive state or "full" state
A spin-inactive state (also called a "full" state) is a electron state, in which both of two possible places for an electron are occupied. The spin-inactive state state has spin "0" and it is described by a scalar wavefunction, which remains unchanged when the coordinate system is rotated.
Such a wavefunction can be expressed as the scalar product of spinors for spin-up and spin-down electrons.
![]()
It is important to emphasize that the wave functions (8) is the same for electrons with any spin direction. This means that the wavefunction (8) is the same for any combination of the spinors of opposite spins.
The time inverse symmetry is not broken for a spin-inactive state. A wavefunction of a spin-inactive state is invariant under axis rotation.
 |
Fig 2.A spin-inactive state state. The time-inverse symmetry is not broken for this electron state. As a result, the spin-inactive state does not have spin direction. Therefore, all these representation of the spin-inactive state are identical and indistinguishable. A wavefunction of a spin-inactive state is invariant under axis rotation. |
3. "empty" state
"empty" is a electron state in which both of the two possible places for an electron are unoccupied.
Similar to the spin-inactive state, the time-inverse symmetry is not broken for the "empty" state. As a result, the " empty" state has no spin direction.
(about broken time-inverse symmetry)
The time- inverse symmetry for an object means that no property of the object changes when the direction of the time is reversed. Let me give you some examples, when the time- inverse symmetry is broken. (example 1): An object rotating around some axis. When the time is inverse, the clockwise rotation changes into the counterclockwise rotation. (example 2): An electrical current. When the time is inverse, the electron flow to the left changes to the flow to the right. The direction of a magnetic field, which is induced by the current, reverses its direction. In contrast, the electrical charge, the electrical field, and electron mass are not affected by the time-reversal.
Symmetry is critically important in Physics. Emptiness or "nothing" or vacuum is a state of full symmetry. As a result, the "nothing" can not be distinguished by any means. In contrast, an elementary particle is a bunch of broken symmetries, which is stable in time. As a result, an elementary particle can be distinguished from the "nothing". Additionally, any symmetry corresponds to one conserved parameter. For example, the symmetry of absolute value of time corresponds to a conserved parameter called the energy. The symmetry of absolute value of time means that all Law of Physics is absolutely the same yesterday, today and tomorrow. The existence itself of any elementary particle breaks this symmetry. The particle might exist today, but will not exist tomorrow. As a result, any elementary particle has the conserved parameter called the energy. Similarly, any property of an elementary particle has corresponding symmetry. For example, the electrical charge corresponds to the symmetry of a change of the phase of the wavefunction. It means if the phase of the wavefunction of one separated electron is changed, nothing happens to the electron. The spin is the conserved parameter corresponding to the symmetry of the time reversal. If an elementary particle remains exactly the same, when the time is reversed, the particle has no spin. If some property of a particle changes, when the time is reversed, the particle has a non-zero spin.
![]() The same content can be found in V. Zayets JMMM 356 (2014)52–67 (click here to download pdf)
The same content can be found in V. Zayets JMMM 356 (2014)52–67 (click here to download pdf)
I will try to answer your questions as soon as possible