Scatterings. Distribution of spin directions in electron gas

Magnetism of electron gas

A spin direction of an electron may not be conserved after a spin-independent scattering. The spin rotations occur due to a quantum-mechanical fact that, when a quantum state is occupied by two electrons of opposite spins, the total spin of the state is 0 and the spin direction of each electron in this state can not be determined.

Because the time-inversion symmetry is conserved after a spin-independent scattering, the electrons in an electron gas can be divided into groups of spin-polarized and spin-unpolarized electrons. Spin-independent scatterings do not change the number of electrons in each group. This is why it is possible to treat the groups of spin-polarized and spin-unpolarized electrons as independent objects in the electron gas.

After these scatterings the spin direction rotates by an arbitrary angle.

Results in short

Important fact: The rotation of the electron spin after a spin-independent scattering!!

(1) Spin direction rotates after frequent spin-independent scatterings.

(2) The time-inverse symmetry is conserved after the

Why it is important??

Because of these frequent scatterings:

(1) it is possible to divide electrons into groups of spin-polarized and spin-unpolarized electrons. (Proof is here)

(2) Only one group of spin-polarized electrons can exists in electron gas. Two groups of spin-polarized electrons of different spin direction can not coexist at same place at the same time. (See spin torque)

(3) Spin-polarized and spin-unpolarized electrons have different energy distributions. (see spin statistics)

(4) The spin-polarized and spin-unpolarized electrons have a different conductivities. (see here)

Note: A conduction electron experience a spin- independent scatterings each 10-100 femtoseconds. It is very frequent.

What is the spin-independent scattering?

It is a scattering, after which the total spin of electrons are conserved.

Could be a scattering spin-dependent.

Yes. It is the case when the conduction electrons also interact with localized electrons. These scatterings are very seldom. The spin-dependent scatterings is the origin of the Anomalous Hall effect and the Spin Hall effect.

Reason why spin is rotated after a spin-independent scattering?

The full-filled state, which are filled by two electrons of opposite spins. The full-filled state does not have spin direction. Therefore, all these representation of a full-filled state are identical and indistinguishable. This feature is due to of the quantum nature of an electron and it is the reason why spin is rotated after a spin-independent scattering.

When an electron state is filled up by two electrons of opposite spin, the spin direction of each electron is not distinguishable. Because of this fact, the spin direction of the electrons may not be conserved even in the case of spin-independent scatterings.

The result of scattering of two half-filled states with angle phi between their spin directions can be full-filled +"empty" states with probability

or it can be two half-filled states of the same spin direction with probability

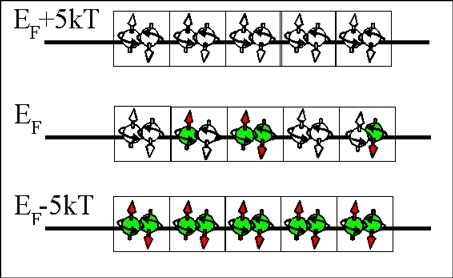This is an important fact, which is used in calculations of the spin statistics and the spin torque.

Electronics states in solid

Fig. 1. Electron states in a solid. Each square represents a delocalized electron states distinguished by a direction of its wave vector k in the Brillouin zone, its energy E and spacial symmetry. Each state can be occupied maximum by two electrons of opposite spin. The number of states is described by the density of states. At the energy E_Fermi+5kT the states are not filled by any electrons. At the energy E_Fermi-5kT almost all states are filled by two electrons of opposite spins and the spin of the states is zero. At the Fermi energy a state may be filled only by one electron and have spin ½.

The spin properties of an electron gas in a metal depend on its density of states near its Fermi energy. Each of the states is distinguished by a direction of its wave vector k in the Brillouin zone, its energy E and its spacial symmetry. Due to the Pauli exclusion principle, each state can be occupied maximum by two electrons of opposite spins. The energy distribution of electrons in the metal is the Fermi-Dirac distribution. The states of a higher energy (at least 5kT above the Fermi energy), are not occupied by electrons and almost all of them are “empty” states with no spin (See Fig. 1). Almost all states of a lower energy (at least 5kT below the Fermi energy) are occupied by two electrons and almost all of them are the “full” states with no spin. A state, energy of which is near the Fermi energy, may be filled by one electron and have spin ½. Also, there is a probability that the state is not filled or it is filled by two electrons of opposite spins (See Fig. 1).

Spin rotation after spin-independent scatterings. Example of an elastic scattering.

Fig.2 Two consecutive scattering events, which result in the rotation of a spin direction. After the first scattering at t=t1 the electron from the state A is scattered into state B. After the second scattering at t=t2 the electron from the state B is scattered into state C.

Let us consider elastic spin-independent scatterings between 3 states, which all have the same energy and this energy is close to the Fermi energy. The states are labeled as “A”, “B” and “C” (See Fig.2) and they are only distinguished by the direction of their wave vector k in the Brillouin zone. Let us assume that at time t0 the state “A” is filled by one electron, which spin is up, the state “B” is filled by one electron, which spin is down, and the state “C” is not filled. At time t1 the electron of the state “A” experiences an elastic scattering. Since the spin-up place of the state “B” is not occupied, the electron may be scattered into state “B”. In this case, at time t1 state “B” becomes occupied by two electrons, its spin is zero and it does not have a spin direction. At time t2 an electron of the state “B” experiences again an elastic scattering into an unoccupied state “C”. Since there was no electron in state “C” at time, the electron scattered into state “C” may have any spin direction. For example, it may have spin-right direction. Than, spin of the electron, which remains on the state “B”, is spin-left. With equal probability the electrons of states “B” and “C” may have spin-front and spin-back directions. Therefore, the electrons have experienced a random spin rotation after two consecutive spin-independent scatterings. The random spin rotation is because of a quantum nature of an electron. At time t1, the spin of state “B” is zero and inside of the electrons there is no record, which spin directions they had at time t0. For example, the three states shown in Fig. 1(b) are equivalent, indistinguishable and all of them equally represents one “full” state. Therefore, when an electron is scattered out off state “B”, its spin can have any direction, only the spin directions of the scattered and remaining electrons should be opposite.

Fig. 3. Three representations of a “full” state which are indistinguishable. This is because spin of the “full” state is zero and the “full” state does not have a spin direction. Because of this quantum- mechanical property of an electron, electron spin rotates after a spin-independent scattering.

Detailed example. Spin rotation in a degenerated GaAs.

Click here to expand and to see the Detailed example. Spin rotation in a degenerated GaAs.

In this example I intentionally have used many unnecessary details in order to avoid possible questions.

Let me take as an example a degenerated n-type GaAs, which Fermi level is 10 kT above the conduction band. The conduction band is isotropic. The temperature is room temperature. The density of state of GaAs at this Fermi energy is about 5.5E20 1/cm3 /eV. Each of state is distinguish by a direction of its wave vector k in the Brillouin zone and its energy E. Due to the Pauli exclusion principle, each state can be occupied maximum by two electrons of opposite spins.

Let me assume that mobility mu of the electrons in the GaAs is 2800 cm2/V/s. Than, the average time between electrons collision can be estimated as τ_collision= μ* m_eff/q=0.28*0.067*9.1E31/1.6E19 s =10 ps, where m_eff=0.067*m_electron is the effective mass of a conduction electron in GaAs and q is the elementary charge.

Let me consider an elastic scattering between 3 states labeled as A, B, C. The energy of states is the same and it is near the Fermi energy. Only the states are distinguished by their direction of the wave vector. For example, the wave vectors of states are directed as

A: , along [0, 0, 1] direction;
B: , 0.1 degree off [0, 0, 1] towards [0, 1, 1] ;
C: 0.1 degree off [0, 0, 1] towards [0, -1, 1].

Important part:

Fig.2 Two consecutive scattering events, which result in the rotation of a spin direction. After the first scattering at t=10 ps the electron from the state A is scattered into state B. After the second scattering at t=20 ps the electron from the state B is scattered into state C.

Let me assume that at time t1=0 ps:

the state A is filled by 1 electron, which spin is up;
the state B is filled by 1 electron, which spin is down;
the state C is not filled by any electrons;

At time t2=10 ps the electron of state A is experience an elastic scattering (for example, on defect). During the scattering, electron spin and electron energy do not change. Only the electron wave vector is changed. Since the spin-up place of state B is not occupied, the electron may be scattered into state B. In this case, at time t2=10 ps the state B becomes occupied by two electrons, its spin is zero and it does not have a spin direction.

At time t3=20 ps, one electron of the state B is scattered into state C due a spin-independent elastic scattering. Since there was no electron in state C at time t2=10 ps, the electron scattered into state C may have any spin direction. For example, it may have spin-left direction. Than, spin of the electron, which remains on the state B, is spin-right.

Therefore, the electrons, which had spin-up/down directions at time t1=0 ps, rotates the spin to spin-left/right directions during 20 ps because of two elastic spin-independent scatterings.

The random spin rotation is because of a quantum nature of electrons. At time t2=10 ps, the spin of state B is zero and inside of the electrons there is no record, which spin direction they had at time t1=0 ps. Therefore, when they are scattered out off state B their spin can have any direction, only the spin directions of both electrons should be opposite.

During about a half of all scatterings that an electron experiences, its spin direction is randomly rotates.

If we suggest that spin directions of all electrons are only in spin-up and spin-down directions, within a short time all directions will be mixed and all electrons are redistributed into the TIS assembly (spins are distributed in all directions with equal probabilities). As was shown in the Chapter 8 (Fig. 9), it takes about 15-30 scatterings for two spin directions to be within 1 degree. Therefore, for above example the short time means 150-300 ps.

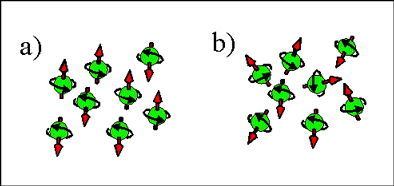General facts about the time-inverse symmetry breaking.

To see general facts about the time-inverse symmetry breaking, Click here to expand

Fig.1 Spin direction of conduction electron in cases when the space symmetry is a) broken b) not broken. In both cases there is no spin accumulation (Total spin is zero) and the time inversion symmetry is not broken.

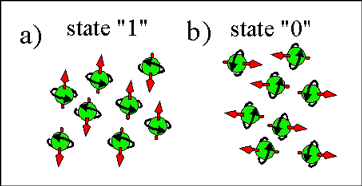Fig.3 The storage of information data by means of direction of spacial symmetry breaking. a) logical "1" b) logical "0". In both cases there is no spin accumulation and the time inverse symmetry is not broken.

The breaking of the time inversion symmetry of an electron gas is essential for any spin manipulation and spin transfer. Only in the case when the time inverse-symmetry is broken, it is possible to manipulate and store the spin data in a electron gas.

Figure 1 shows two different angular distributions of spins of conduction electrons. One distribution corresponds to the case when the spacial inversion symmetry is broken and the other distribution corresponds to the case when it is not broken. In both cases there is no spin accumulation and the time-inversion symmetry is not broken. However, the distributions are significantly different. When the spacial symmetry of an object is broken , it is possible to store a bit of information in this object. Figure 3 shows an example of how to store a bit of information in an electron gas by means of a direction of the spacial symmetry breaking without breaking of the time-inverse symmetry.

Note: Spin distribution of Fig.1(a) corresponds to two TIA assemblies (See below), which quickly relax into one TIA assembly.

Difference between the classical and proposed models

In the classical model (of spin-up/spin-down bands) it is assumed that

a full-filled state is time-inverse symmetrical, but is not symmetrical for an arbitrary spacial rotation.

The classical model ignores the fact that spin of a full-filled state is zero!

In the proposed model (of TIA/TIS assemblies)

a full-filled state is time-inverse symmetrical and it is symmetrical for an arbitrary spacial rotation.

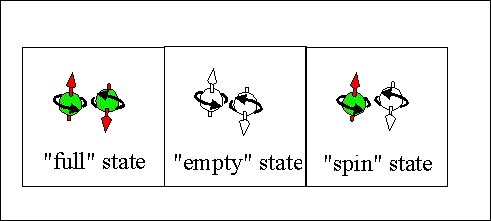This is because the spin of a full-filled state is zero!

The time-inverse symmetry of an electron state

Fig 5. Three possibilities of occupation of an electron state. A quantum state can be filled ether by two electrons of opposite spins (full-filled state or "full" sate) or it can be filled only by one electron and the second place is not filled (half-filled state or half-filled state") or the state can be not filled at all (empty state). For details, see here

In a solid an electronic state can be occupied either a) by two electrons of opposite spin; b) one electron c) no electrons. The properties of an electron state are different in each case. These 3 states are defined as half-filled, "full' and "empty" states (See Fig 5). For details, see here.

1. full-filled states and "empty" states have spin zero, they do not have a spin direction and they are time-inverse symmetrical

2. half-filled states have spin = 1/2, they do have a spin direction and they are time-inverse asymmetrical

An important fact :

this fact is due to the quantum nature of spin

Since spin of a full-filled state is zero, the full-filled state does not have a spin direction. The spin directions of individual electrons of the full-filled state are not distinguishable. This is because a wavefunction of a particle with zero spin is invariant under rotation.

Spin-independent scatterings

By a spin-independent scattering we define a scattering, which does not depend on the electron spin. This means that there is no spin operator in the Hamiltonian describing the scattering event.

During the spin-independent scattering the spin direction may not be conserved (see below), but the time-inversion symmetry/asymmetry is always conserved after any spin-independent scattering.

1. Scatterings, which do not change the spin direction

A scattering of one electron from a half-filled state into an "empty" state (Fig 6) does not change the spin direction.

A scattering of one electron from a full-filled state into an half-filled state (Fig 7) does not change the spin direction .

These two processes are responsible for establishing a thermo-equilibrium.

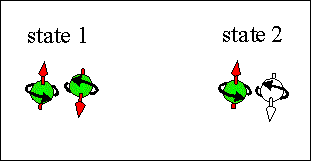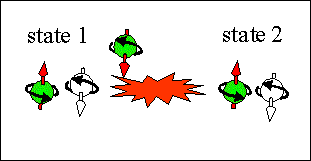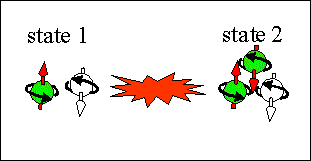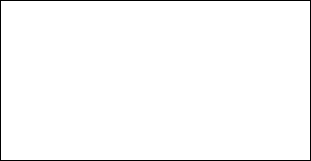Fig.6. scattering event N1. Scattering of spin into an "empty" state. Spin direction is conserved during this scattering event

Fig.7. Scattering event N2. Scattering of spin into a full-filled state. Spin direction is conserved during this scattering event

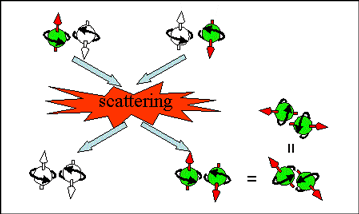
2. Scatterings, which change the spin direction

a) Scattering of two spin states into full-filled +"empty" states. (scattering event N3)

Fig.8 Scattering event N3.

During this scattering, an electron from a half-filled state is scattered into an unoccupied state of another half-filled state. The result is one full-filled state and one "empty" state.

During this scattering, two time-inverse asymmetric half-filled states, both of which have spin directions, are converted into two time-inverse symmetric states, which do not have any spin direction.

Because of the quantum nature of spin, the information about spin direction of electrons before the scattering is fully lost after this scattering

Note: Before the scattering, a system of two half-filled states is not invariant under axis rotation. After the scattering, the system of full-filled and "empty" states is invariant under axis rotation.

About the rotational invariance see Wikipedia's explanation here

b) The generation of a pair of half-filled states with an arbitrary spin direction. Scattering event N4.

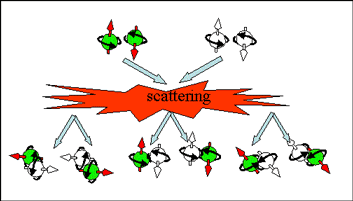
Fig.9 Scattering event N4.

For this scattering an electron from a full-filled state is scattered into an "empty" state. The result is two half-filled states with arbitrary, but opposite spin directions.

During the scattering, two time-inverse symmetric states, which do not have any spin direction, are converted into two time-inverse asymmetric half-filled states, which have spin directions.

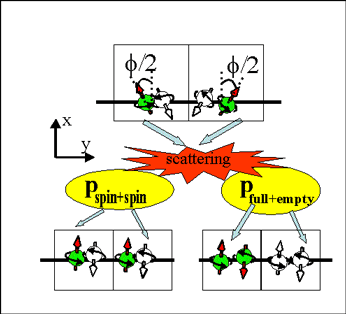c. Scatterings of two half-filled states with different spin direction. Scattering event N5

Fig.10 Scattering event N5.

The scattering of two “spin” states , which have the angle phi between their spin directions, results either two “spin” states or a “full” state + a “empty” state.

Below I the probabilities for the scattered electrons to be either in half-filled states or in full-filled plus "empty" states are found.

Let us consider a scattering of two “spin” states. The spin directions of the “spin” states are in the seaplane and have an angle -phi /2 and phi /2 with respect to the x-axis (See Fig.10). These “spin” states are described by the spinors

Two spin states can be considered as a closed system, the wavefunction of which is described by a product of the spinors S1 and S2. A spin-independent scattering can be considered as a perturbation inside the closed system and it does not change the overall wavefunction of the whole closed system. Therefore, even after the scattering the two electrons are described by the same overall wavefunction as before the scattering. Even though the wavefunction of each electron may change, the overall wavefunction of two electrons does not change. It is described by a product of the spinor S1 and S2.

The spin of a “full” and an “empty” state is zero. Therefore, the wavefunction of a “full” or an “empty” states is a scalar with respect to an axis rotation. There is only one possible scalar from a binary product of spinors S1 and S2. It is called the scalar product of spinors S1 and S2 (see Ref.1). Therefore, the wavefunction of a “full” + an “empty” state is the scalar product of spinors S1 and S2 and the probability that the scattered electrons will be in a “full” + an “empty” state is calculated as

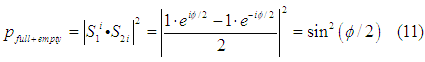
Since the result of a scattering of two half-filled states can be either “full” +”empty” states or two “spin” states, from Eq. (11) the probability of the scattered electrons to be in “spin” states is given as

[1] L.D. Landau and E.M. Lifshitz. “Quantum mechanics. Non-relativistic theory”, 3d ed., Pergamon, 1991, pp. 207-208.

To see details about the spinor of half-filled states , click here to expand

The wavefunction of two non-interacting electrons is the vector product of corresponding spinors:

The spinor describing the “spin” state, in which the electrons are scattered, is defined by the binary form (12b), from which the scalar product of spinors S1 and S2 is excluded. It is the dot product of spinors S1 and S2* and the vector product of S1 and S2

In the case when spinors S1 and S2 are given by Eqs.(10), Eq. (12c) is simplified as

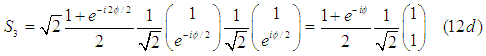
The probability that the scattered electrons will be in “spin” states is calculated from Eq.12d as

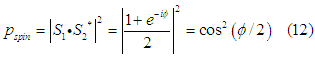
Fig 11. Animated graph. Scattering event N6. After these two consecutive spin-independent scattering the spin direction rotates by an arbitrary angle.

d) The rotation of spin direction. Scattering event N6.

This event consists of two consecutive spin-independent scatterings: event N3 and event N4 or could be also N5 and N4. The result of these consecutive spin-independent scatterings is a spin rotation. The rotation angle can be any angle with an equal probability.

Groups of spin-polarized and spin-unpolarized conduction electrons

Even though the spin direction of one electron is not conserved after scattering event N6, the total spin of all conduction electrons is conserved. Due to this fact it is possible to divide all conduction electrons into two groups. In the first group contains “spin” states with the same spin direction. The total spin of the first group is non-zero. In the second group there are “spin” states, “full” states and “empty” states. The total spin of the electrons of the second group is zero. Even though there is a frequent exchange of electrons between the groups, the number of electrons in each group does not change due to spin-independent scatterings. This division of conduction electrons into two groups significantly simplifies the analysis of the magnetic properties and spin-dependent transport of an electron gas.

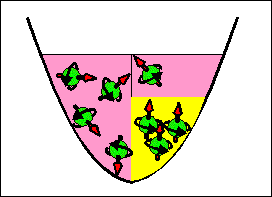Fig 12. The conduction electrons in a solid. Some electrons are in the group of spin-unpolarized electrons (pink color) and some electrons in the group of spin-polarized electrons (yellow color). The spins of spin-unpolarized electrons are distributed equally in all directions. The spins of spin-polarized electrons are in the same direction.

group of spin unpolarized electrons

It consists of full-filled states, "empty" states and half-filled states. The balance between the conversion of half-filled states into full-filled and "empty" states (scattering event N5) and the reverse conversion (scattering event N4) determines the number of full-filled, "empty" and half-filled states in the group of spin unpolarized electrons. Details see here.

The full-filled and "empty" states do not have any spin direction. There is an equal number of 'spin' states with all spin directions. Therefore, the group of spin unpolarized electrons does not have any spin direction and the total magnetic moment of the group of spin unpolarized electrons is zero.

The overall wavefunction, which describes all electrons in the group of spin unpolarized electrons, is a scalar.

In equilibrium in a non-magnetic metal all conductions electrons are only in the group of spin unpolarized electrons (there is no spin-polarized electrons and there is no spin accumulation).

Distribution of spin directions in the group of spin-polarized electrons can be described as

where 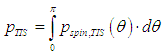is the probability of an electron to be in the group of the spin-unpolarized electrons.

group of spin polarized electrons

Fig.13 Distribution of spin directions in case of (left) a small spin accumulation and (right) a large spin accumulation. The yellow surface shows the distribution of spins in the group of spin-unpolarized electrons. The blue surface shows distribution of spin in the group of spin-polarized electrons. The length of vector from center to surface represents the number of electrons, which spin is directed along direction of the vector.

The upper pictures are 3D-representations. The distance from the (0,0,0) point to the surface is proportional to the number of electrons, whose spin is directed along the corresponded direction. The lower pictures are the 2D representations.

The group of spin polarized electrons contains only states filled by one electron. Spins of all electrons in this group are in the same direction. The group of spin polarized electrons has a direction, along which the time-inverse symmetry is broken (spin direction).

In the case when the spin direction of the spin-polarized electrons is along the z-axis (theta=0), the distribution of spin directions of the group of spin-polarized electrons is described as

where delta is the delta-function.

There is a frequent exchange of electrons between the groups of spin-polarized and spin-unpolarized electrons due to the scattering event 5. However, the spin-independent scatterings do not change the number of electrons in each group. Because of the frequent exchange of electrons, the spin-polarized and spin-unpolarized electrons are in the same thermal equilibrium (they have the same temperature).

In a magnetic field, the electrons from the group of spin-unpolarized electrons are converted into the group of spin-polarized electrons (a spin accumulation is created). (See the electron gas in a magnetic field here)

Figure 13 shows an example of the distribution of spin directions in the group of spin-polarized and spin-unpolarized electrons for two cases of the different spin accumulations. The distribution of the spin directions of spin-unpolarized electrons is a sphere. It is because in this group the spins are equally distributed in all directions.. The distribution of the spin directions of spin-polarized electrons is a delta-function. It is because all spins are directed in the same direction.

The equilibrium number of electrons in groups of spin-polarized and spin-unpolarized electrons

In the following the equilibrium number of spin-polarized and spin-unpolarized electron is calculated. Also, it is proved that spin-independent scatterings do not change the number of electrons in each group (for the proof, click the bar bellow to expand the calculations). For more details See Spin statistics.

let us imagine that it is possible to create the electron gas with an arbitrary distribution of spin directions. Below I will show that within a very short time the electrons of the electron gas are scattered and redistributed into one group of spin-polarized electrons (all spin are in one direction) and one group of spin-unpolarized electrons (spins are equally distributed in all directions). from any given arbitrary distribution of spin directions in the electron gas, it is possible to calculate the final spin polarization of the electron gas.

Let us assume that at some moment in time the angular distribution of spin directions is , where is the probability for a “spin” state to have an angle between their spin direction and the z-axis in an interval . After the scatterings have redistributed the states into one group spin-polarized electrons and one group spin-unpolarized electrons, the probability of an electron is spin-polarized can be calculated as

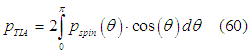
The probability that electron is in the group spin-unpolarized electrons can be calculated as

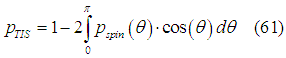
Bellow-calculations also prove that the numbers of electrons in the groups of spin-polarized and spin-unpolarized electrons do not change due to spin-independent scatterings.

To see the calculations, which were used to obtain Eqs. (60) ,(61) , click here to expand

Even though a spin-independent scattering may rotate the spin of an individual electron, spin-independent scatterings do not affect the total spin of all conduction electrons. This is because, all conduction electrons can be considered as a closed system. The spin-independent scatterings are events occurring inside this closed system and only a source from the outside can change its spin.

The conservation of the total spin during spin-independent scatterings also means the conservation of the projection of the total spin on any axis. The projection of the total spin on some axis can be calculated by summing up the spin projections of each electron on this axis.

It should be noticed that the spin is not a conventional 3D geometric vector. For summing of spins the quantum mechanical rules should be used instead of the rules for geometrical vectors.

Note: here we are calculated only half-filled states, which are filed only by one electron. The full-filled states, which are filled by two electrons of opposite spins, have zero spin and they have no influence on this calculations. The number of the full-filled states can be calculated by the Spin statistics.

If the spin direction of an electron has angle theta with respect to the z-axis, the probability that this state has spin direction along (spin-up) and opposite (spin-down) to the z-axis is

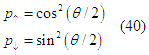
(For proof see Eq. (p.7) here (click to expand "Spin projection"))

For example, if is the probability of an electron to have an spin angle in the interval with respect to the z-axis, the probability that an electron has spin direction along (spin-up) and opposite (spin-down) to the z-axis is calculated as

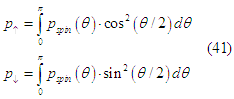
The difference in probabilities of an electron to have either spin-up or spin-down direction is calculated from Eq. (41) as

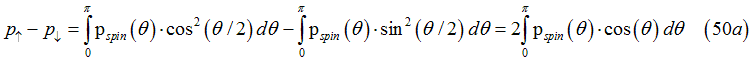
or the same

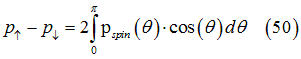
In the group of the spin-unpolarized electrons, the spins are distributed equally in all directions. It means that probabilities to find electrons with opposite spins are equal for any direction. Or the same the sum of the projections of spin onto any axis over all states should not depend on the axis direction. Spin-up, spin-left, and spin-down and any other projection should be the same. For example, the spin-up and spin-down projections are the same:

Substituting Eq.(51) into Eq. (50), we obtain

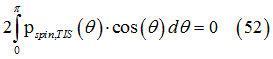
where is the probability for a spin-unpolarized electron to have an spin angle in the interval

All spins of spin-polarized electrons are directed in the same direction. Therefore, the probability to find a spin-polarized electron with spin directed opposite to the spin direction of all spin-polarized electrons is zero:

From Eq.(50) the probability to find spin-polarized electron with spin-up direction is

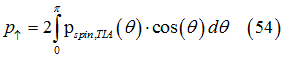
where is the probability to find a spin-polarized electron with an spin angle in the interval

Since all spin-polarized electrons are spin-up, Eq.(54) simply describe the probability for an electron to be spin-polarized.

Main part

If at some moment in time the electron gas has some arbitrary distribution of spin directions , after a short time electrons are scattered and redistributed into the group of spin-polarized electrons and the group of spin-unpolarized electrons. The spin-independent scatterings do not change the total spin of the electron gas. Therefore, the spin projection Eq.(41) does change as well. It means that does not matter how many spin-independent scatterings occur, the value of the integral of Eq.(50) remains the same. But after a sufficient number of scatterings all electrons are redistributed into the groups of spin-polarized and spin-unpolarized electrons. Therefore, the integral of Eq.(54) gives the probability for an electron to be spin-polarized.

Summing-up:

Knowing the angular spin distribution prior to equilibrium, in equilibrium the probability that an electron is spin-polarized can be calculated from Eqs.(44) and (45) as

The probability that electron is spin-unpolarized can be calculated from Eq. (47) as

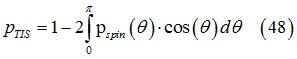
It should be noticed that Eq (47) is asymmetrical for time reversal (the integral changes its sign when time is reversed) and Eq. (48) is symmetrical for time reversal.

Since the time-inverse symmetry is conserved after a spin-independent scattering, the numbers of electrons in the groups of spin-polarized and spin-unpolarized electrons do not change due to spin-independent scatterings.

Possible confusion!!: from 2014 to 2017 I have used names TIA and TIS for groups of spin-polarized and spin-unpolarized electrons, respectively. The reasons are explained here.

The same content can be found in V. Zayets JMMM 356 (2014)52–67 (click here to download pdf) or (http://arxiv.org/abs/1304.2150 or this site) . Chapter 2 (pp.4-7) is about the rotation of the spin direction after a spin independent scattering. Chapter 3 (pp.7-9) is about the assemblies.

Dr. Vadym Zayets

v.zayets(at)gmail.com

more Chapters on this topic:

more Chapters on this topic:

more Chapters on this topic:

more Chapters on this topic:

more Chapters on this topic:

more Chapters on this topic:

more Chapters on this topic:

more Chapters on this topic: