Dr. Vadym Zayets
v.zayets(at)gmail.com
My Research and Inventions
click here to see all content |

Dr. Vadym Zayetsv.zayets(at)gmail.com |
|
 |
more Chapters on this topic:IntroductionTransport Eqs.Spin Proximity/ Spin InjectionSpin DetectionBoltzmann Eqs.Band currentScattering currentMean-free pathCurrent near InterfaceOrdinary Hall effectAnomalous Hall effect, AMR effectSpin-Orbit interactionSpin Hall effectNon-local Spin DetectionLandau -Lifshitz equationExchange interactionsp-d exchange interactionCoercive fieldPerpendicular magnetic anisotropy (PMA)Voltage- controlled magnetism (VCMA effect)All-metal transistorSpin-orbit torque (SO torque)What is a hole?spin polarizationCharge accumulationMgO-based MTJMagneto-opticsSpin vs Orbital momentWhat is the Spin?model comparisonQuestions & AnswersEB nanotechnologyReticle 11
more Chapters on this topic:IntroductionTransport Eqs.Spin Proximity/ Spin InjectionSpin DetectionBoltzmann Eqs.Band currentScattering currentMean-free pathCurrent near InterfaceOrdinary Hall effectAnomalous Hall effect, AMR effectSpin-Orbit interactionSpin Hall effectNon-local Spin DetectionLandau -Lifshitz equationExchange interactionsp-d exchange interactionCoercive fieldPerpendicular magnetic anisotropy (PMA)Voltage- controlled magnetism (VCMA effect)All-metal transistorSpin-orbit torque (SO torque)What is a hole?spin polarizationCharge accumulationMgO-based MTJMagneto-opticsSpin vs Orbital momentWhat is the Spin?model comparisonQuestions & AnswersEB nanotechnologyReticle 11
more Chapters on this topic:IntroductionTransport Eqs.Spin Proximity/ Spin InjectionSpin DetectionBoltzmann Eqs.Band currentScattering currentMean-free pathCurrent near InterfaceOrdinary Hall effectAnomalous Hall effect, AMR effectSpin-Orbit interactionSpin Hall effectNon-local Spin DetectionLandau -Lifshitz equationExchange interactionsp-d exchange interactionCoercive fieldPerpendicular magnetic anisotropy (PMA)Voltage- controlled magnetism (VCMA effect)All-metal transistorSpin-orbit torque (SO torque)What is a hole?spin polarizationCharge accumulationMgO-based MTJMagneto-opticsSpin vs Orbital momentWhat is the Spin?model comparisonQuestions & AnswersEB nanotechnologyReticle 11
more Chapters on this topic:IntroductionTransport Eqs.Spin Proximity/ Spin InjectionSpin DetectionBoltzmann Eqs.Band currentScattering currentMean-free pathCurrent near InterfaceOrdinary Hall effectAnomalous Hall effect, AMR effectSpin-Orbit interactionSpin Hall effectNon-local Spin DetectionLandau -Lifshitz equationExchange interactionsp-d exchange interactionCoercive fieldPerpendicular magnetic anisotropy (PMA)Voltage- controlled magnetism (VCMA effect)All-metal transistorSpin-orbit torque (SO torque)What is a hole?spin polarizationCharge accumulationMgO-based MTJMagneto-opticsSpin vs Orbital momentWhat is the Spin?model comparisonQuestions & AnswersEB nanotechnologyReticle 11
more Chapters on this topic:IntroductionScatteringsSpin-polarized/ unpolarized electronsSpin statisticselectron gas in Magnetic FieldFerromagnetic metalsSpin TorqueSpin-Torque CurrentSpin-Transfer TorqueQuantum Nature of SpinQuestions & Answers
more Chapters on this topic:IntroductionTransport Eqs.Spin Proximity/ Spin InjectionSpin DetectionBoltzmann Eqs.Band currentScattering currentMean-free pathCurrent near InterfaceOrdinary Hall effectAnomalous Hall effect, AMR effectSpin-Orbit interactionSpin Hall effectNon-local Spin DetectionLandau -Lifshitz equationExchange interactionsp-d exchange interactionCoercive fieldPerpendicular magnetic anisotropy (PMA)Voltage- controlled magnetism (VCMA effect)All-metal transistorSpin-orbit torque (SO torque)What is a hole?spin polarizationCharge accumulationMgO-based MTJMagneto-opticsSpin vs Orbital momentWhat is the Spin?model comparisonQuestions & AnswersEB nanotechnologyReticle 11
|
Running-wave and standing-wave electrons. The Boltzmann transport equations. Spin and Charge TransportAll conduction electrons can be divided into two groups: running-wave electrons and standing-wave electrons. The standing-wave electrons are conduction electrons, which bounce between defects or interfaces. Therefore, they do not transport the spin or the charge during their movement. The running-wave electrons freely move in along the crystal. They transport the the spin and the charge during their movement. Both the running-wave electrons and standing-wave electrons transport the spin and the charge due to scatterings.The the Boltzmann Transport Equations are used to calculate the charge σcharge, spin-diffusion σspin, detection σdetection and injection σinjection conductivities. These 4 conductivities are used in Spin/Charge Transport equations in order to describe the spin and charge transport in different materials and geometries.Importance of the Boltzmann Transport Equations is that they can describe the spin/charge transport in a case when different transport mechanisms equally contribute. For example, in the case of the Spin Hall effect or the Anomalous Hall effect the band current, which flows along applied electrical field, induces the scattering current, which flows perpendicularly to the applied electrical field.
|
|
- running-wave electrons
- standing-wave electrons
electrons of different types contribute differently to the transport
- band current
- scattering current
![]()
Running waves |
Standing waves |
|
 |
| The running-wave electrons are moving in the space. They can be scattered. Therefore, these electrons contributes to both conductivities | The quantum mechanic does not require that an object with non-zero speed necessary moves in the space. One example is a standing wave, which has non-zero speed, does not move in the space. A standing wave can be decomposed as a sum of two waves running in opposite directions. The standing-wave electrons contribute only to the scattering conductivity. |
It is important to emphasize that not all conduction electrons contribute to the band current. All conduction electrons can be divided into two different types: running-wave and standing-wave electrons. The standing-wave electrons do not contribute to the band current. This fact is explained as follows. The effective length of the conduction electrons is rather long (See Fig. 3). In a semiconductor it can be as long as a hundred of nanometers. In the case when an average distance between defects in a conductor is comparable with the effective length of a conduction electron, the electron may bounce back and forwards between defects. It is similar to the case of a photon, which are bouncing between walls of a resonator. When bouncing between defects, one electron, which is moving forward, is firmly fixed to the electron, which is moving backward. These coupled electrons do not move along crystal. They are fixed at position of defects. Importunately, when an electrical field is applied, there is still one electron moving forward and one electron moving backward. The electrical field does not change the ratio of electrons moving in the opposite directions for this type of electrons. Therefore, these electrons do not contribute to the band current. These coupled electrons are defined as the standing-wave electrons. The electrons, which can move freely along crystal and which are not fixed to one position, are defined as the running-wave electrons. Only they contribute to the band current.
The wave nature of electrons significantly influences the spin and charge transport in a solid. Since an electron is a wave, it is not necessary that an electron with non-zero speed moves and transports a spin and charge. A standing wave has a non-zero speed, but it does not move. For a calculation of the transport it is important to distinguish two classes of the electrons: the standing-wave electrons and the running-wave electrons. A standing-wave electron can be imagined as an electron bouncing back and forward between two defects. Also, a standing wave electron can be viewed as two electrons, which move in opposite directions. In order to build up a standing-wave electron two defects are not necessarily required, but one defect is often sufficient. Also, a standing-wave electron can form at an interface. The probability a standing-wave electron can be formed at a defect or an interface can be characterized by a parameter called the reflectivity R. The reflectivity R is defined as the ratio of the probability for an electron to be a standing-wave type to the probability to be a running wave type.
A standing-wave electron. An example, an electron bouncing between two defects. |
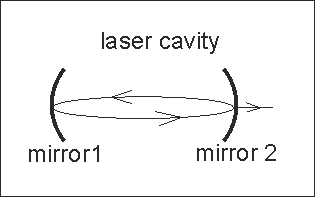
Standing-wave photon in laser cavity |
|
 |
Note: An electron can bound to only one defect making a standing wave electronNote: An electron can bound to an interface making a standing wave electron |
Photon is reflecting back and forward between two mirrors forming a standing wave. When the photon passes through the semi-transparent mirror, it becomes the running-wave photon. Similarly, a standing-wave electron is reflected between two defects (note, only one defect is sufficient to make a standing wave electron) |
A. There are standing-wave electrons in conductors with defects, in multilayers and in vicinity of the interface between two conductors( See here and here). The existence of the standing-wave electrons influences significantly the spin and charge transport. For example, in the vicinity of an interface there are more standing-wave electrons and less running-wave electrons comparing to the bulk of the conductor. As result, the ordinary conductivity σcharge decreases near the interface. The detection σdetection and injection σinjection conductivities experiences even larger changes. The values of both conductivities are near zero in the bulk, but in the vicinity of interface their values may increase substantially and their values may become comparable to the value of the ordinary conductivity σcharge. It is the reason why the spin detection, which is the effect of charge accumulation along spin diffusion, only has been observed at an interface, but not in the bulk of a conductor. It is also the reason why the spin transfer by an electrical current and the spin injection are more effective along or across an interface than in the bulk of a conductor.
In the bulk of a high-crystal-quality metal with a low density of defects and dislocations, most of the electrons are running-wave electrons. In a metal with a larger amount of defects or in the vicinity of an interface, there is a substantial amount of the standing-wave electrons. In the vicinity of a contact between two metals, there are both running-wave electrons and standing-wave electrons, because the reflectivity of such a contact is usually small. In contrast, in the vicinity of higher resistance contacts like a tunnel barrier contact or a semiconductor-metal contact almost all electrons are standing-wave electrons.
Yes. Both the standing-wave electron and the running-wave electrons are described by the spin-statistics,
It is because of a very large difference between scattering probabilities between a running-wave electron to a standing-wave electron and a running-wave electron to a localized electron. The sizes of a running-wave electron are a standing-wave electron are nearly the same. In contrast, the size of a localized electron is much smaller. The scattering probability between a running-wave electron to a standing-wave electron is nearly the same as scattering probability between two running-wave electrons. The scattering probability between a running-wave electron to a localized electron is very small.
![]() It is important that the scattering probability between a standing-wave electron to a localized electron is higher than between a running-wave electron to a localized electron. This is the reason why existence of the standing-wave electron enhances the sp-d exchange interactions
It is important that the scattering probability between a standing-wave electron to a localized electron is higher than between a running-wave electron to a localized electron. This is the reason why existence of the standing-wave electron enhances the sp-d exchange interactions
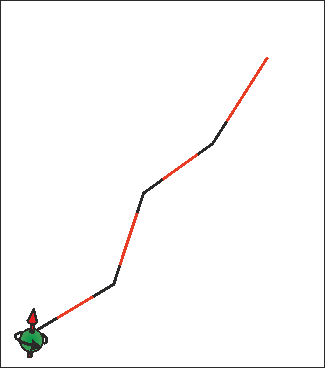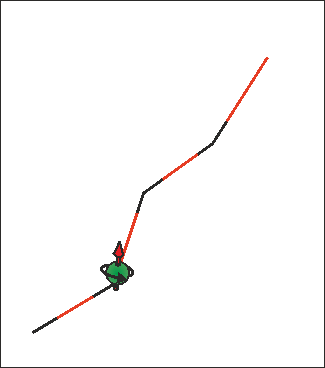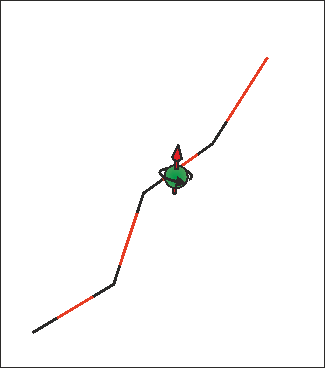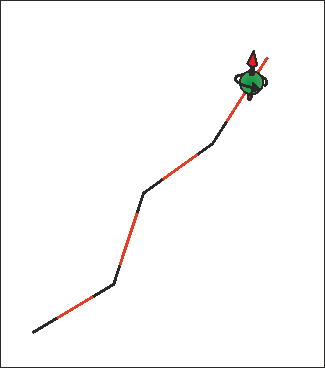 |
Simplified picture describing two conductivities in a solid. The running-wave electron transport corresponds to electron movement between two consequent scatterings over the length of electron mean free path (red line). The scattering transport occurs over a short distance during electron scattering (blue line) |

1. Band current. This transport occurs because of movement of electrons between scatterings. Only running-wave electrons contributes to this transport.
2. Scattering current. This transport occurs because of movement of electrons due to scatterings. Both the running-wave and standing-wave electrons contributes to this current.
There are two major mechanisms of electron transport in an electron gas. A running-wave electrons transport the spin and charge, because they move along crystal. If there is a smaller amount of electrons moving in one direction than the amount of electrons moving in the opposite direction, there is a flow of charge and/or spin in this direction. Such a type of current is defined as the band current. This transport describes the transport of the charge and the spin by electrons between scatterings. It is important to emphasis that only the running-wave electrons, but not standing-wave electrons contribute to this current.
In the electron gas the electrons are continuously scattered from one quantum states to another quantum state. When the probability for an electron to be scattered in one direction is higher than the probability to be scattered in the opposite direction, there is a flow of the spin and charge in this direction. This current is defined as the scattering current. Both the standing-wave and running-wave electrons may contribute to this current. Usually the scattering current is significantly less efficient than the running-wave-electron current for the charge and spin transport
Reason 1: It is simpler to calculate each current separately.
Reason 2: The path of each electron in the phase space can be separated when it is scattered and when it moves between scatterings. Therefore, the contribution of each transport mechanisms should be treated in the Boltzmann transport equation individually and separately.
Reason 3: From a case to a case the contributions of the band and scattering currents are very different.
Reason 4: Spin properties
Two mechanisms of the Spin&Charge transport by Running-Wave Electrons.(1) Band Current . Origin: Constant movement of electrons in a metal (2) Scattering current. Origin: electron scattering from one state to another |
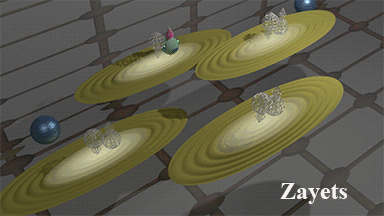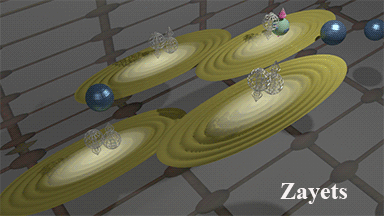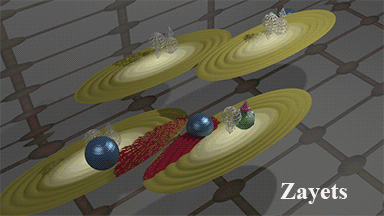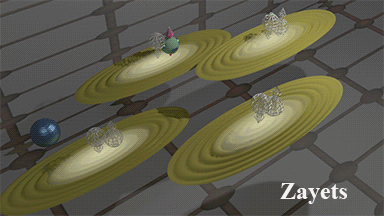 |
Click on image to enlarge it. Blue balls represent defects. Brown ellipse shows distribution of wave function of the running-wave electrons. A scattering occurs when wave functions of two states are overlapped (shown in red color) Note: An electron current is shown. A hole current of the running wave electrons is shown here. |
Spin/Charge Transport Equations |
 |
From the Boltzmann Transport Equations, the charge conductivity ![]() , spin conductivity
, spin conductivity ![]() , detection conductivity
, detection conductivity ![]() and injection conductivity
and injection conductivity![]() can be found
can be found
What we are looking for???
The Boltzmann transport equations are the equations to find the distribution function ![]() . Knowing the distribution function
. Knowing the distribution function ![]() , it is straightforward to calculate spin and charge currents.
, it is straightforward to calculate spin and charge currents.
The probability to find an electron at a point r with pulse p at time t is described by the distribution function ![]() (often it is called the density function, but instead I will use the more-common quantum-mechanical name the distribution function).
(often it is called the density function, but instead I will use the more-common quantum-mechanical name the distribution function).
How to include spin in the transport equations???
![]() three distribution functions are used, which describes the probabilities for a quantum state to be filled by one, two or none electrons correspondingly. Also, the half-filled states can be filled either by an electron from group of spin-polarized or spin-unpolarized electrons. The condition that a quantum state can be only in one of these three states gives
three distribution functions are used, which describes the probabilities for a quantum state to be filled by one, two or none electrons correspondingly. Also, the half-filled states can be filled either by an electron from group of spin-polarized or spin-unpolarized electrons. The condition that a quantum state can be only in one of these three states gives
![]()
Instead of one classical Boltzmann transport equation, it should be used a system of three transport equations for half-filled “full” and “empty” states.
Factors, which can change the distribution functions![]() :
:
1) movement of electrons in the space (diffusion). The running-wave electrons are always moving. Additionally the electrons can move by scattering from a state to a state.
2) changing of electrons energy and momentum under an applied field.
3) The conversion of electrons from one assembly to another assembly.
4) The relaxation of a distribution function to an equilibrium distribution function.
The Boltzmann equation, which describes a temporal evolution of the distribution function, is given as as
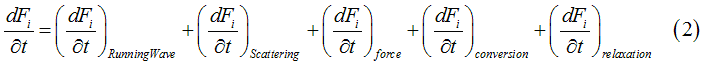

where "i" labels the distribution functions for half-filled “full” and “empty” states; 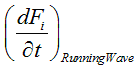 is the term, which describes a change of the distribution function due to the movement of the running-wave electrons;
is the term, which describes a change of the distribution function due to the movement of the running-wave electrons; 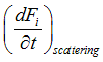 is the scattering term, which describes the changing of the distribution function due to movement of the electrons by scatterings from a quantum state to a quantum state;
is the scattering term, which describes the changing of the distribution function due to movement of the electrons by scatterings from a quantum state to a quantum state;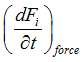 is the force term, which describes the changing of the distribution function due an external field;
is the force term, which describes the changing of the distribution function due an external field; 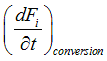 describes electron conversion between assemblies;
describes electron conversion between assemblies;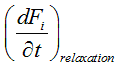 is the relaxation terms, which describes relaxation of the distribution function to an equilibrium distribution function due to the electron scatterings.
is the relaxation terms, which describes relaxation of the distribution function to an equilibrium distribution function due to the electron scatterings.
In equilibrium the distributions of spin-polarized electrons ![]() , the distributions of spin-unpolarized electrons, electrons in full-filled states and "empty" states
, the distributions of spin-unpolarized electrons, electrons in full-filled states and "empty" states ![]() are determined by the spin statistics. and the spin polarization sp of the electron gas. The spin polarization sp of the electron gas can be calculated as
are determined by the spin statistics. and the spin polarization sp of the electron gas. The spin polarization sp of the electron gas can be calculated as
.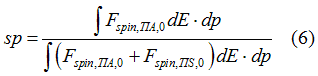
Since usually the conversion rate between TIA and TIS assemblies are not energy dependent, from Eq (6) the spin polarization of electron gas is given as
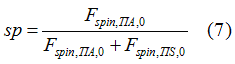
Since a quantum state can be filled either by one, none or two conduction electrons, the equilibrium distribution functions are satisfied to the condition:
![]()
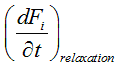 without specifying the mechanisms of the scatterings.
without specifying the mechanisms of the scatterings. In this approximation two facts are used:
1) When all external perturbations are switched off, the distribution functions relax to the equilibrium distribution functions.
2) The speed of the relaxation is linearly proportional to a deviation of the distribution function from equilibrium
Except for some special cases it is safe to assume that an external perturbation (applied electrical and magnetic fields, a thermo gradient, a gradient of spin accumulation, a sp-d exchange effective field and a spin-orbit effective magnetic field) is sufficiently small so that under the perturbation the distribution function is only slightly different from the distribution function in an equilibrium. In this case the distribution function can be represented as
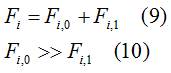
where ![]() is the distribution function in equilibrium and condition (8) is valid for any point of the phase space.
is the distribution function in equilibrium and condition (8) is valid for any point of the phase space.
In the case when the applied field is switched off, the distribution function F_i relaxes to the equilibrium distribution function F_i,0 Then
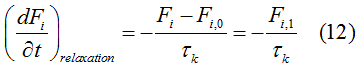
where "i" corresponds to half-filled, full-filled and "empty" states" and![]() is the momentum relaxation time.
is the momentum relaxation time.
Since electrons are constantly scattered between assemblies at a high rate, ![]() is the same for both assemblies and
is the same for both assemblies and ![]() is the same for distributions of half-filled, full-filled and "empty" states.
is the same for distributions of half-filled, full-filled and "empty" states.
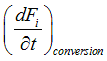 terms describes the conversion of electrons between assemblies
terms describes the conversion of electrons between assemblies
The electrons of the TIA assembly are converted into the TIS assembly, because of the different mechanisms of spin relaxation. The rate of the conversion due to spin relaxation is proportional to the number of spin states in the TIA assembly:
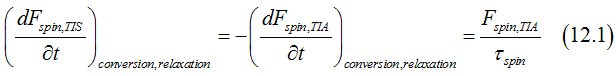
where ![]() is the spin life time.
is the spin life time.
The electrons of the TIS assembly are converted into the TIA assembly due to the spin pumping. The conversion rate is linearly proportional to the number of the spin states in the TIS assembly:
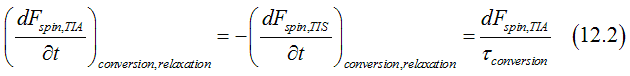
where ![]() is effective time of conversion, which is reverse proportional to
is effective time of conversion, which is reverse proportional to
From Eqs.(12.1) and (12.2), the conversion rate between the TIS and TIA assemblies is obtained as
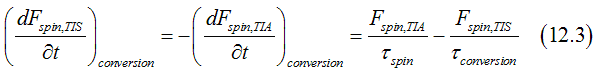

Knowing the distribution functions for spin, full and empty states allows to calculate the number of states in the assemblies
The number of quantum states in a volume of the phase space ![]() is equal to
is equal to 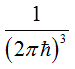 . Integrating over all states in a special volume
. Integrating over all states in a special volume![]() , the number of electron is calculated as
, the number of electron is calculated as
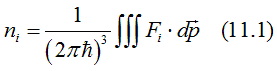
where index "i" indicates half-filled states of the TIS assembly, full-filled states, "empty" states and half-filled states of the TIA assembly. For example, the number of half-filled states in the TIA assembly (spin accumulation) can be calculated as
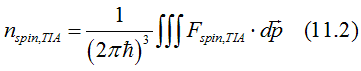
where F_spin is the distribution function for the half-filled states in the TIA assembly.
Since each state transforms charge "-e", the current of the i- states can be calculated as


In an equilibrium the number of the i- states in an assembly can be calculated as
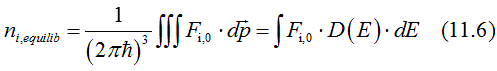
where D(E) is the density of states. Since in the equilibrium there is no current
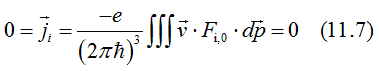
where e is the charge of an electron
When an external perturbation applied to the electron gas, the currents may flow in a metal and and the electron can be converted either in or out from the an assembly
The currents can be calculated as
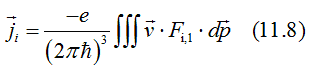
It is often important to calculate a current along some direction, for example, along a gradient of the chemical potential or a gradient of the spin polarization. In this case the cylindrical coordinate needs to be used. If theta is the angle between the electron movement direction and the direction of the current flow. Then, the number of electrons can be calculated as
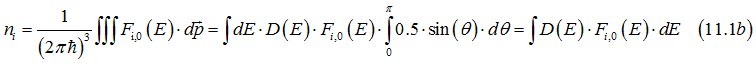
where D(E) is the density of the states.
The current can be calculated as
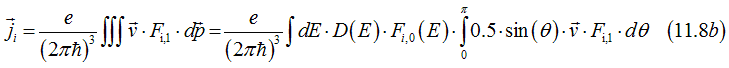

The conversion rate can be calculated as
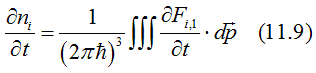
Note the currents Eq.(11.8) is not zero only in the case when the distribution function depends on electron speed as

The running-wave-electron term and the scattering term of the Boltzmann transport equation are of this type.
The conversion is not zero only in the case when the distribution function depends on electron speed as
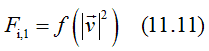
The conversion terms of the Boltzmann transport equation is of this type.
The spin/charge transport equations are derived from the Boltzmann transport equations using the continuity equations.
The charge and spin are conserved values. They can not appear from nowhere and can not disappear to nowhere. The half-filled states can be converted between assemblies. The spin and/or charge can diffuse from a point to a point. This continuity equations describes these facts as
![]()
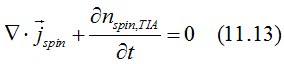
where the spin conversion and the spin and charge currents can be calculated from Eqs. (11.4), (11.5), (11.8), (11.9). For details, see here.
![]() (about mixing conductance) Is there any chapter about spin mixing conductance?i dont understand the meaning of spin mixing conductance which appears in many literatures about the spin transport
(about mixing conductance) Is there any chapter about spin mixing conductance?i dont understand the meaning of spin mixing conductance which appears in many literatures about the spin transport
I think the term of the mixing conduction was introduced by Prof. Ralph in order to solve obvious problems of the spin-up/spin-down diffusion model. I don't think the mixing conduction has some physical meaning. It is just a mathematical trick to fix the obvious problem of the old approximate model of the spin transport. I do not recommend using it.
There are two possible methods for describing the spin transport. The 1st old method is using the currents of the spin projections meaning spin-down and spin-up spin projections. The 2nd method is the use of currents of spin-polarized and spin-unpolarized electrons. Since the are two groups of the conduction electrons in a ferromagnetic metal: spin-polarized and spin-unpolarized electrons, there are only two possible currents in the metal: the current of the spin-polarized electrons and the current of spin-unpolarized electrons. Of course, it is possible to use spin projections instead of the true spin direction for a description of the spin current. For the simplest cases, it could be easier. However, even for a case of moderate difficulty, the description based on the spin projections hits its limit and becomes incorrect.
There is no such thing as a current of spin projection. There is no such thing as a current of the spin-up or spin-down electrons, there are currents of spin-polarized and spin-unpolarized electrons. Each of these currents can be represented as a sum of the spin-up and spin-down currents.
The problem of the spin-up/spin-down model is that it is based on nonexistent objects (spin-up and spin-down currents). When a calculation is based on something non-existent, it is easy to violate a basic Law of Physics and get a wrong result. Next, in order to fix it one needs to introduce something.
In contrast, when one works with truly- existed objects like the currents of spin-polarized and spin- unpolarized electrons, everything is simple and straightforward. There are only simple and understandable parameters like conductivities of the spin-polarized and spin-unpolarized electrons, it is not necessary to introduce new strange parameters and the theory perfectly fits to experiment without usage of any mathematical tricks.
![]() The same content can be found in this paper
The same content can be found in this paper ![]() and this paper
and this paper![]()
I will try to answer your questions as soon as possible