Dr. Vadym Zayets
v.zayets(at)gmail.com
My Research and Inventions
click here to see all content |

Dr. Vadym Zayetsv.zayets(at)gmail.com |
|
 |
more Chapters on this topic:IntroductionTransport Eqs.Spin Proximity/ Spin InjectionSpin DetectionBoltzmann Eqs.Band currentScattering currentMean-free pathCurrent near InterfaceOrdinary Hall effectAnomalous Hall effect, AMR effectSpin-Orbit interactionSpin Hall effectNon-local Spin DetectionLandau -Lifshitz equationExchange interactionsp-d exchange interactionCoercive fieldPerpendicular magnetic anisotropy (PMA)Voltage- controlled magnetism (VCMA effect)All-metal transistorSpin-orbit torque (SO torque)What is a hole?spin polarizationCharge accumulationMgO-based MTJMagneto-opticsSpin vs Orbital momentWhat is the Spin?model comparisonQuestions & AnswersEB nanotechnologyReticle 11
more Chapters on this topic:IntroductionTransport Eqs.Spin Proximity/ Spin InjectionSpin DetectionBoltzmann Eqs.Band currentScattering currentMean-free pathCurrent near InterfaceOrdinary Hall effectAnomalous Hall effect, AMR effectSpin-Orbit interactionSpin Hall effectNon-local Spin DetectionLandau -Lifshitz equationExchange interactionsp-d exchange interactionCoercive fieldPerpendicular magnetic anisotropy (PMA)Voltage- controlled magnetism (VCMA effect)All-metal transistorSpin-orbit torque (SO torque)What is a hole?spin polarizationCharge accumulationMgO-based MTJMagneto-opticsSpin vs Orbital momentWhat is the Spin?model comparisonQuestions & AnswersEB nanotechnologyReticle 11
more Chapters on this topic:IntroductionTransport Eqs.Spin Proximity/ Spin InjectionSpin DetectionBoltzmann Eqs.Band currentScattering currentMean-free pathCurrent near InterfaceOrdinary Hall effectAnomalous Hall effect, AMR effectSpin-Orbit interactionSpin Hall effectNon-local Spin DetectionLandau -Lifshitz equationExchange interactionsp-d exchange interactionCoercive fieldPerpendicular magnetic anisotropy (PMA)Voltage- controlled magnetism (VCMA effect)All-metal transistorSpin-orbit torque (SO torque)What is a hole?spin polarizationCharge accumulationMgO-based MTJMagneto-opticsSpin vs Orbital momentWhat is the Spin?model comparisonQuestions & AnswersEB nanotechnologyReticle 11
|
Band Current (Ordinary Electrical Current). Non-Magnetic Material. Spin and Charge TransportThe band current is the major electron transport mechanism in the bulk metal. It occurs because of movent of electrons between scatterings. An electron moving along electrical field gains the energy. In contrast, an electron moving opposite to electrical field loses the energy. As a result, the number of electrons, which move along and opposite to the electrical field, becomes different. It results a electron current flow along (or opposite) to the applied electrical field.It is very efficient transport mechanism.
|
| Origin of Band (Ordinary) Electrical Current in a Metal and a Semiconductor |
|---|
| (main fact): The electrical current flows in a metal and a semiconductor under an applied electrical filed, because the number of conduction electrons moving along and opposite to the electrical field becomes different due to the electrical field |
| The current is not because the electrons move faster along the electrical field |
| click on image to enlarge it |
| Numbers nforward and nbackward of forward- and backward- moving electrons vs. electron energy under an electrical field |
|---|
| Energy distribution of electrons under an electrical field. Right part (blue) shows electrons nforward moving along the electrical field (forward). Left part (green) shows electrons nbackward moving opposite to the electrical field (backward). The total number of electrons moving in each direction is calculated by an integration over all energies. |
| (note): The "electrons" are electrons, which energy is larger than the Fermi energy EF (shown in upper graph). The "holes" are electrons, which energy is smaller than the Fermi energy EF (shown in upper graph). |
| (note): The energy distributions of "electrons" and "holes" describes by the same distribution, the center of which is shifted under an electrical field. |
| (note): An electron moving along electrical field gains the energy. In contrast, an electron moving opposite to electrical field loses the energy. As a result, the electron distribution of "electrons" and "holes" is shifted towards the electrical field. |
(electron current): More electrons move along the electrical field than opposite to the field (upper graph) |
(hole current): More electrons move opposite to the electrical field than along the field (upper graph) |
| click on image to enlarge it |
(transport mechanism of a current of conduction electrons):![]()
![]()
![]() The transport mechanism of a current of the conduction electrons is different from mechanism a water flow in river, but it is the similar as the mechanism of a slow wind flow in air. There are many conduction electrons and they all move in all different directions. In equilibrium, for any direction, the numbers of electrons moving in the forward and backward directions are precisely equal. Therefore, even though the conduction electrons are moving, in total as assembly they don't transfer the charge or the spin. When an electrical field or another distortion is applied, the subtle balance is broken and there are more condition electrons moving in one direction (e.g. along the electrical field) than in the opposite direction. As a result, the conduction electrons begin to transfer the charge and the spin.
The transport mechanism of a current of the conduction electrons is different from mechanism a water flow in river, but it is the similar as the mechanism of a slow wind flow in air. There are many conduction electrons and they all move in all different directions. In equilibrium, for any direction, the numbers of electrons moving in the forward and backward directions are precisely equal. Therefore, even though the conduction electrons are moving, in total as assembly they don't transfer the charge or the spin. When an electrical field or another distortion is applied, the subtle balance is broken and there are more condition electrons moving in one direction (e.g. along the electrical field) than in the opposite direction. As a result, the conduction electrons begin to transfer the charge and the spin.
 Even in absence of an electron current, the conduction electrons are moving in directions, but there is an equal amount of electrons moving in any two opposite directs and therefore there is no net current. E.g. an applied electrical field is creates electron current. The electron current means that there are more conduction electrons, which move in the direction of the current, than the electrons, which move in the opposite direction.
Even in absence of an electron current, the conduction electrons are moving in directions, but there is an equal amount of electrons moving in any two opposite directs and therefore there is no net current. E.g. an applied electrical field is creates electron current. The electron current means that there are more conduction electrons, which move in the direction of the current, than the electrons, which move in the opposite direction. Electron current for "holes" and "electrons" |
||||||||||||||
|
||||||||||||||
|
||||||||||||||
| (electrons) |
||||||||||||||
| (holes) |
||||||||||||||
| Full description of the electron and hole current is here | ||||||||||||||
| click on image to enlarge it |
(fact 1): ![]() Even in an absence of an electron current, the conduction electrons are moving in all directions at a substantial speed. However, there is an equal amount of electrons moving in each two opposite directions.
Even in an absence of an electron current, the conduction electrons are moving in all directions at a substantial speed. However, there is an equal amount of electrons moving in each two opposite directions.
(fact 2): ![]() (about "electron current) In a conductor with an electron conductivity, there are more conduction electrons, which move along an electrical field, than electrons, which move opposite to the electrical field. As a result, there is an electron current along the electrical field.
(about "electron current) In a conductor with an electron conductivity, there are more conduction electrons, which move along an electrical field, than electrons, which move opposite to the electrical field. As a result, there is an electron current along the electrical field.
(fact 3): ![]() (about a hole current). Only negatively- charged conduction electrons are carriers for the charge and the spin in a solid. There is no any type of positively charged particle, which are able to carry the charge and the spin in a solid.
(about a hole current). Only negatively- charged conduction electrons are carriers for the charge and the spin in a solid. There is no any type of positively charged particle, which are able to carry the charge and the spin in a solid.
(fact 4): ![]() (about a hole current). In a conductor with an hole conductivity, there are more conduction electrons, which move opposite to an electrical field, than electrons, which move along the electrical field. As a result, there is an electron current opposite to the electrical field, which is called a hole current and which is very similar to a flow of positively charged particles.
(about a hole current). In a conductor with an hole conductivity, there are more conduction electrons, which move opposite to an electrical field, than electrons, which move along the electrical field. As a result, there is an electron current opposite to the electrical field, which is called a hole current and which is very similar to a flow of positively charged particles.
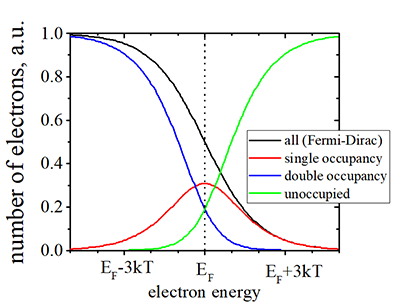 |
(black line) Number of electrons vs. the electron energy. The Fermi- Dirac distribution. |
| (red line) Number of electrons in states, which are occupied by one electron |
| (blue line) Number of electrons in states, are occupied by two electrons of opposite spins |
| (black line)= (red line)+(blue line) |
| (green line) Number of of empty places in states, which is not occupied either by one or two electrons |
| Check more details here or math to obtain the distribution |
| Click on image to enlarge it |
(fact 1) ![]() The transport properties of a conduction electron are different whether it solely occupies a quantum state or together with another electron of opposite spin
The transport properties of a conduction electron are different whether it solely occupies a quantum state or together with another electron of opposite spin
(fact 2) ![]() The electron spin substantially influence the transport properties even in a non-magnetic metal, in which the electron gas is not spin polarized
The electron spin substantially influence the transport properties even in a non-magnetic metal, in which the electron gas is not spin polarized
(quantum nature of electron) (restriction 1): The energy distribution of electrons is the Fermi- Dirac distribution.
(restriction 1): The energy distribution of electrons is the Fermi- Dirac distribution.
(quantum nature of electron) (restriction 2): Each conduction electron occupies one quantum state. Each quantum state can be occupied by one or two conduction electrons
(restriction 2): Each conduction electron occupies one quantum state. Each quantum state can be occupied by one or two conduction electrons
(quantum nature of electron)  (restriction 3): The electron can change its energy (e.g. in an electrical field) or movement direction only if the corresponded quantum state is unoccupied and available for the electron to be scattered into.
(restriction 3): The electron can change its energy (e.g. in an electrical field) or movement direction only if the corresponded quantum state is unoccupied and available for the electron to be scattered into.
(quantum nature of electron) (restriction 4): The conduction electron can be scattered in a quantum state, which is already occupied by one electron, only when its spin is opposite to the spin of the already- existing electron
(restriction 4): The conduction electron can be scattered in a quantum state, which is already occupied by one electron, only when its spin is opposite to the spin of the already- existing electron
![]() The energy distributions of conduction electrons in a quantum states, which occupied by a single electron and by two electrons of opposite spins, are different, because of difference of their scattering probabilities.
The energy distributions of conduction electrons in a quantum states, which occupied by a single electron and by two electrons of opposite spins, are different, because of difference of their scattering probabilities.
( Scattering path) ![]() From a solely- occupied state to an "empty" state
From a solely- occupied state to an "empty" state
(major contribution): E>2kT;. In this energy range: slope of scattering probability: negative. The electrons behaves as: a negatively-charged particle
(result in an electrical field) ![]() (moving along field) a solely- occupied state gains its energy. As a result, number of a higher energy solely- occupied states becomes larger
(moving along field) a solely- occupied state gains its energy. As a result, number of a higher energy solely- occupied states becomes larger ![]() (moving opposite to field) a solely- occupied state losses its energy. As a result, number of a lower energy solely- occupied states becomes smaller
(moving opposite to field) a solely- occupied state losses its energy. As a result, number of a lower energy solely- occupied states becomes smaller

( Scattering path) ![]() From a doubly- occupied state to an solely- occupied state
From a doubly- occupied state to an solely- occupied state
(major contribution): E< -2kT; In this energy range: slope of scattering probability: positive. The electrons behaves as: a positively-charged particle
(result in an electrical field) ![]() (moving along field) a doubly- occupied state gains its energy. As a result, number of a higher energy doubly- occupied states becomes larger, but there is no change for solely- occupied states
(moving along field) a doubly- occupied state gains its energy. As a result, number of a higher energy doubly- occupied states becomes larger, but there is no change for solely- occupied states ![]() (moving opposite to field) a doubly- occupied state losses its energy. As a result, number of a higher energy solely- occupied states becomes larger, but there is no change for solely- occupied states.
(moving opposite to field) a doubly- occupied state losses its energy. As a result, number of a higher energy solely- occupied states becomes larger, but there is no change for solely- occupied states.

( Scattering path) ![]() From a doubly- occupied state to an "empty" state
From a doubly- occupied state to an "empty" state
(major contribution): -2kT <E< +2kT; In this energy range: slope of scattering probability: both positive and negative. The electrons behaves as: a positively- and negatively- charged particle

( Scattering path) ![]() From a solely- occupied state to an solely- occupied state
From a solely- occupied state to an solely- occupied state
(major contribution): -2kT <E< +2kT; In this energy range: slope of scattering probability: both positive and negative. The electrons behaves as: a positively- and negatively- charged particle
Electron and Hole currents. Quantum limitations |
||||||||||||||||
(fact) |
||||||||||||||||
|
||||||||||||||||
| click on image to enlarge it. |
 Calculation of electrical current from Boltzmann equations
Calculation of electrical current from Boltzmann equations
 See general calculation of electron current from Boltzmann equation here
See general calculation of electron current from Boltzmann equation here
![]()
![]()
![]()
![]()
![]()
![]() See calculation of electron and spin currents in a magnetic material here.
See calculation of electron and spin currents in a magnetic material here.
When an electrical field is applied, the electron distribution is modified: numbers of electrons moving in opposite directions become different.
(step 1a)![]() Calculation of energy, which each electron gains or loses in an electrical field.
Calculation of energy, which each electron gains or loses in an electrical field.
The electron gains/ losses energy during time interval between scatterings. The scatterings redistribute the electrons according to their energy with respect to Fermi- Dirac distribution
(fact) ![]() The energy change for each electron depends on the electron moving direction with respect to the applied field
The energy change for each electron depends on the electron moving direction with respect to the applied field
(step 1b)![]() Calculation of the mean-free path. (See here)
Calculation of the mean-free path. (See here)
The energy, which electron gains/loses between consequent scatterings, is proportional to the mean- free path. The longer the the mean-free path is, the longer the time interval between two consequent scatterings and therefore the larger energy the electron gains/loses from the electrical field.
(step 1c)![]() Calculation of the electron scattering probability.
Calculation of the electron scattering probability. ![]()

 The electron can occupy a quantum state only if it is not yet occupied by another electron (The quantum state is empty).
The electron can occupy a quantum state only if it is not yet occupied by another electron (The quantum state is empty).An electron can gain/loss energy only if the state of the higher/lower energy is not yet occupied. (The state is empty). Otherwise, the electrical field has no influence on the electron. For example, the electrons of inner orbitals, which energy is substantially lower than the Fermi energy, are not influenced at all by the electrical field, because all their neighbor states of a slightly different energy are fully occupied.
(step 2) ![]()
![]() : Calculation of number difference of electrons moving in each two opposite directions
: Calculation of number difference of electrons moving in each two opposite directions
(step 3) ![]()
![]() : Calculation of of current by integrating over all contributions from electrons moving in each specific direction
: Calculation of of current by integrating over all contributions from electrons moving in each specific direction
In a metal (also in a semiconductor) the electron and hole are the same object. They are both the negatively- charged electrons. The electrons and holes are distinguished by their energy with respect to the Fermi energy and more specific how their energy distribution (= number of electrons at at a specific energy) changes with an electron energy change. The energy of "electrons" is above Fermi energy and the energy of holes is below the Fermi energy. The number of "electrons" decrease when the electron energy increases. The number of "holes" decreases when the energy increases.
In a metal, the Fermi energy is inside of the electron band and therefore the number of electrons and the holes is nearly equal.
In a semiconductor, the Fermi energy is inside the bandgap and there is a large difference in number of electrons above and below the Fermi energy. In a n-type semiconductor, the Fermi energy is just below the conduction band and there are many "electrons", but there are nearly no "holes". In a p-type semiconductor, the Fermi energy is just above the valence band and there are many "holes", but there are nearly no "electrons".
 Why the electron energy with respect to the Fermi energy defines whether an electron is the "electron" or the "hole"
Why the electron energy with respect to the Fermi energy defines whether an electron is the "electron" or the "hole"
One can distinguish between the "electron" and the "hole" by the direction, into which the electrons move in an electrical field. A negatively-charged particle moves from "-" to "+", but a positively-charged particle moves in the opposite direction from "+" to "-". Even though for both cases of the "electrons" and "holes", a single electron is always accelerated in an electrical field in the same direction from "-" to "+", this acceleration of a single electron courses different direction of the collective movement of the "electron" and "holes".
(where is the magic?) ![]() Why the same direction of movement of a single electron causes different direction of collective movement of many electrons?
Why the same direction of movement of a single electron causes different direction of collective movement of many electrons?
In a solid the conduction electrons move at a high speed in all directions even without an external electrical field. In absence of an external magnetic field, the numbers of electrons, which move in two opposite directions, are exactly equal. E.g. the number of conduction electrons, which move to the left, are the same as the number of the electrons, which move to the right. When an external electrical field is applied, for example, to the left direction, an electron, which moves along the field, accelerates and gains the energy. In contrast, an electron, which moves opposite to the electrical field, slows down and loses its energy. As a result, the electrical field makes an energy shift between the energy distributions for the electrons moving to the left and to the right and therefore the number of the electrons moving to the left and moving to the right, becomes different and therefore there is an electron current along the electrical field. The direction of the current is determined in which direction the left or the right there are more electrons moving.
| Numbers nforward and nbackward of forward- and backward- moving electrons vs. electron energy under an electrical field |
|---|
| Energy distribution of electrons under an electrical field. Right part (blue) shows electrons nforward moving along the electrical field (forward). Left part (green) shows electrons nbackward moving opposite to the electrical field (backward). The total number of electrons moving in each direction is calculated by an integration over all energies. |
| (note): The "electrons" are electrons, which energy is larger than the Fermi energy EF (shown in upper graph). The "holes" are electrons, which energy is smaller than the Fermi energy EF (shown in upper graph). |
| (note): The energy distributions of "electrons" and "holes" describes by the same distribution, the center of which is shifted under an electrical field. |
| (note): The An electron moving along electrical field gains the energy. In contrast, an electron moving opposite to electrical field loses the energy. As a result, the electron distribution of "electrons" and "holes" is shifted towards the electrical field. |
(electron current): More electrons move along the electrical field than opposite to the field (upper graph) |
(hole current): More electrons move opposite to the electrical field than along the field (upper graph) |
| click on image to enlarge it |
In the case of the "electrons" (the electrons, which energy are larger than the Fermi energy), the number of electrons decreases when the electron energy increases. It means that the number of the accelerated electrons, which moves to the left and gain the energy, becomes larger. In contrast, the number of the slowed electrons, which moves to the right and loss their energy, becomes smaller. This imbalance of the electron numbers results in the flow of the electron current to the left.
In the case of the "holes" (the electrons, which energy are smaller than the Fermi energy), the number of electrons increases when the electron energy increases. It means that the number of the accelerated electrons, which moves to the left and gain the energy, becomes smaller. In contrast, the number of the slowed electrons, which moves to the right and loss their energy, becomes smaller. This imbalance of the electron numbers results in the flow of the electron current to the right.
(it is the magic!!!) ![]() (Acceleration of a single electron along an electrical field causes the collective movement of electrons in the opposite direction)
(Acceleration of a single electron along an electrical field causes the collective movement of electrons in the opposite direction) ![]() As explained in above paragraph, the acceleration of electrons in one direction may decrease the decrease of the total number of the electrons, which is moving in this direction (the case of holes) and therefore create an electron current in the opposite direction. It happens due to frequent electron scatterings and redistribution of the electrons according the Fermi- Dirac energy distribution.
As explained in above paragraph, the acceleration of electrons in one direction may decrease the decrease of the total number of the electrons, which is moving in this direction (the case of holes) and therefore create an electron current in the opposite direction. It happens due to frequent electron scatterings and redistribution of the electrons according the Fermi- Dirac energy distribution.
(fact) ![]() The number of electrons, which participate in the electron transport, decreases when the electron energy becomes either larger or smaller than the Fermi energy (See here)
The number of electrons, which participate in the electron transport, decreases when the electron energy becomes either larger or smaller than the Fermi energy (See here)
(fact) ![]() The flow of the electron current in an electrical field occurs not because the electron move faster along the field, but because the number of electrons moving along the electric field becomes larger than the number of the electron moving opposite to the field.
The flow of the electron current in an electrical field occurs not because the electron move faster along the field, but because the number of electrons moving along the electric field becomes larger than the number of the electron moving opposite to the field.
![]() From Ken: Dear Sir, thank you for writing on this subject. It does not make sense to me and the explanations I have read on the Web dodge the real question. I would like to build on the question raised by Prameela, and add more details. (1) Please imagine a block of pure Silicon. The Silicon lattice locks the atoms in place and the atoms lock the electrons in place: the electrons therefore stay with their host atoms, bound by the positive charge of the nucleus. (As far as I know there are no free electrons moving around within the Silicon lattice.) Every atom in the block of Silicon has the same number of electrons and protons. Every Silicon atom in the block of Silicon is electrically neutral and the block itself is electrically neutral. There are no ions in the block. (1) Please imagine that this block is now doped with Boron atoms. In terms of electrical neutrality, nothing has changed. All Silicon atoms are neutral; all Boron atoms are neutral; the block itself is neutral. There are no ions in the block. (3) Magically a new entity appears in the block that wasn't there before. People call it a "hole" for no particular reason. The hole is not an atom, it is not an electron. I don't think it is any known subatomic particle. And magically these "holes' carry a positive charge. If you go back slightly in time, the Silicon atoms did not carry any electrical charge; the Boron atoms did not carry any electrical charge; the block of Silicon did not carry any electrical charge; nobody has connected the block of Silicon to a battery. Yet magically there are undefinable entities inside the block that carry positive charges that were not there before. And these undefinable entities move around within the Silicon lattice. People refer to this as P- Type Silicon. I believe the P stands for "positive." And within semiconductors the positive charge of these undefinable entities in the P- Type Silicon is said to attract free electrons from the N-Side. And when the N-Side free electrons diffuse over to the P- Type Silicon they are said to "fill the hole" or neutralize the positive charges that magically appeared in the P- Side. (4) My question is, where did a metal block containing Silicon atoms and Boron atoms that are 100% electrically neutral suddenly acquire positive electrical charges strong enough to attract free electrons from the N-Type Silicon and then neutralize free electrons that come within proximity?
From Ken: Dear Sir, thank you for writing on this subject. It does not make sense to me and the explanations I have read on the Web dodge the real question. I would like to build on the question raised by Prameela, and add more details. (1) Please imagine a block of pure Silicon. The Silicon lattice locks the atoms in place and the atoms lock the electrons in place: the electrons therefore stay with their host atoms, bound by the positive charge of the nucleus. (As far as I know there are no free electrons moving around within the Silicon lattice.) Every atom in the block of Silicon has the same number of electrons and protons. Every Silicon atom in the block of Silicon is electrically neutral and the block itself is electrically neutral. There are no ions in the block. (1) Please imagine that this block is now doped with Boron atoms. In terms of electrical neutrality, nothing has changed. All Silicon atoms are neutral; all Boron atoms are neutral; the block itself is neutral. There are no ions in the block. (3) Magically a new entity appears in the block that wasn't there before. People call it a "hole" for no particular reason. The hole is not an atom, it is not an electron. I don't think it is any known subatomic particle. And magically these "holes' carry a positive charge. If you go back slightly in time, the Silicon atoms did not carry any electrical charge; the Boron atoms did not carry any electrical charge; the block of Silicon did not carry any electrical charge; nobody has connected the block of Silicon to a battery. Yet magically there are undefinable entities inside the block that carry positive charges that were not there before. And these undefinable entities move around within the Silicon lattice. People refer to this as P- Type Silicon. I believe the P stands for "positive." And within semiconductors the positive charge of these undefinable entities in the P- Type Silicon is said to attract free electrons from the N-Side. And when the N-Side free electrons diffuse over to the P- Type Silicon they are said to "fill the hole" or neutralize the positive charges that magically appeared in the P- Side. (4) My question is, where did a metal block containing Silicon atoms and Boron atoms that are 100% electrically neutral suddenly acquire positive electrical charges strong enough to attract free electrons from the N-Type Silicon and then neutralize free electrons that come within proximity?
![]() A. I would like to answer your question step by steps. At first, I will clarify some details, which you have mentioned in your question. Next, I will answer to the main part of your question.
A. I would like to answer your question step by steps. At first, I will clarify some details, which you have mentioned in your question. Next, I will answer to the main part of your question.
(detail 1)![]() You wrote: : the electrons therefore stay with their host atoms, bound by the positive charge of the nucleus. (As far as I know there are no free electrons moving around within the Silicon lattice.)
You wrote: : the electrons therefore stay with their host atoms, bound by the positive charge of the nucleus. (As far as I know there are no free electrons moving around within the Silicon lattice.)
(localized and conduction electrons)![]()

![]() It is not correct. Only electrons of inner shells (like f- and d- electrons) stay with their host atoms (positive nucleus). Such electrons are called the localized electrons. The electrons of outer shells always move along the crystal lattice and do not stay at one host nucleus. In a solid, the atoms are very close to each other, the outer shells are strongly overlapped with neighbor shells forming the electron states covering all nucleuses. Such electrons are called conduction electrons. In a high- crystal -quality Si, the size (length) of conduction electron can be longer than one micrometer (See my Web page on the mean free path). It means that one conduction electron covers simultaneously more than a million of nucleuses. Also, simultaneously billions of conduction electrons overlap each other. All conduction electrons always move along the crystal lattice (even in equilibrium when there is no electrical current).
It is not correct. Only electrons of inner shells (like f- and d- electrons) stay with their host atoms (positive nucleus). Such electrons are called the localized electrons. The electrons of outer shells always move along the crystal lattice and do not stay at one host nucleus. In a solid, the atoms are very close to each other, the outer shells are strongly overlapped with neighbor shells forming the electron states covering all nucleuses. Such electrons are called conduction electrons. In a high- crystal -quality Si, the size (length) of conduction electron can be longer than one micrometer (See my Web page on the mean free path). It means that one conduction electron covers simultaneously more than a million of nucleuses. Also, simultaneously billions of conduction electrons overlap each other. All conduction electrons always move along the crystal lattice (even in equilibrium when there is no electrical current).
(reason why conduction electrons exists in a solid) ![]()

![]() It is easier to understand the fact of existence of the conduction electron in a solid by imagining an electron as a photon in a simplified 2D world. When an electron is confined in a single atom (e.g. in an atom of atomic gas), it is confined in a small area of atom. The confinement means that the electron bouncing back and forward between two imaginary mirrors or two walls. Because of the confinement (which is created by the electrical field of the nucleus), the electron cannot escape the atom. When many atoms are pushed together, the electron orbitals are overlapped, the confinement is broken (the walls are broken), there is no more back/forward reflection and the electron freely moves through all atoms.
It is easier to understand the fact of existence of the conduction electron in a solid by imagining an electron as a photon in a simplified 2D world. When an electron is confined in a single atom (e.g. in an atom of atomic gas), it is confined in a small area of atom. The confinement means that the electron bouncing back and forward between two imaginary mirrors or two walls. Because of the confinement (which is created by the electrical field of the nucleus), the electron cannot escape the atom. When many atoms are pushed together, the electron orbitals are overlapped, the confinement is broken (the walls are broken), there is no more back/forward reflection and the electron freely moves through all atoms.
(differences between localized and conduction electrons)![]()
![]()

![]() There are many substantial differences between conduction and localized electrons. (difference 1) A conduction electron overlaps millions of nucleus and millions of other conduction electrons. A localized electron is bound to one host nucleus and it only slightly overlaps its close neighbor localized electrons. (difference 2) Conduction electrons are scattered very frequently (~ each 500 fs). In contrast, a scattering of a localized electron is a rare event. (~ each ms or even a second). (difference 4) The conduction electrons are main contributors to the charge, spin, heat transport in a solid. The localized electrons have nearly no contribution to the transport. (difference 4) the distributions of spin directions are very different for localized and conduction electrons (See my Web page on spin polarization)
There are many substantial differences between conduction and localized electrons. (difference 1) A conduction electron overlaps millions of nucleus and millions of other conduction electrons. A localized electron is bound to one host nucleus and it only slightly overlaps its close neighbor localized electrons. (difference 2) Conduction electrons are scattered very frequently (~ each 500 fs). In contrast, a scattering of a localized electron is a rare event. (~ each ms or even a second). (difference 4) The conduction electrons are main contributors to the charge, spin, heat transport in a solid. The localized electrons have nearly no contribution to the transport. (difference 4) the distributions of spin directions are very different for localized and conduction electrons (See my Web page on spin polarization)
(transport mechanism by conduction electrons):![]()
![]()
![]() The transport mechanism of the conduction electrons is different from mechanism a water flow in river, but it is the same as the mechanism of wind flow in air. There are many conduction electrons and they all move in all different directions. In equilibrium, for any direction, the numbers of electrons moving in the forward and backward directions are precisely equal. Therefore, even though the conduction electrons are moving, in total as assembly they don't transfer the charge or the spin. When an electrical field or another distortion is applied, the subtle balance is broken and there are more condition electrons moving in one direction (e.g. along the electrical field) than in the opposite direction. As a result, the conduction electrons begin to transfer the charge and the spin. This is the general transport mechanism in a solid (See more detail here).
The transport mechanism of the conduction electrons is different from mechanism a water flow in river, but it is the same as the mechanism of wind flow in air. There are many conduction electrons and they all move in all different directions. In equilibrium, for any direction, the numbers of electrons moving in the forward and backward directions are precisely equal. Therefore, even though the conduction electrons are moving, in total as assembly they don't transfer the charge or the spin. When an electrical field or another distortion is applied, the subtle balance is broken and there are more condition electrons moving in one direction (e.g. along the electrical field) than in the opposite direction. As a result, the conduction electrons begin to transfer the charge and the spin. This is the general transport mechanism in a solid (See more detail here).
Electron current for "holes" and "electrons" |
||||||||||||||
|
||||||||||||||
|
||||||||||||||
| (electrons) |
||||||||||||||
| (holes) |
||||||||||||||
| Full description of the electron and hole current is here | ||||||||||||||
| click on image to enlarge it |
(in which material conduction electron exist) ![]()

![]() There are conduction electrons in any solid: in a metal, in a semiconductor and even in a dielectric. The transport properties of conduction electrons depend on availability of unoccupied states for conduction electrons (or the same: the number of states at the Fermi energy). As I described above, in order to transport the charge and/or the spin it should be more electrons moving in one direction than in the opposite direction. Therefore, some unoccupied state should be available for conduction electrons moving in the forward direction. In a metal, there are plenty of unoccupied states and therefore the metal is a good conductor. In contrast, in a dielectric there is no any unoccupied state. Since all states are occupied, the electrons cannot be scattered from a state or into a state and therefore the number of moving in any specific direction cannot be changed. As a result, the conduction electrons cannot transport any charge or spin in a dielectric. It is the fact that there are lots of conduction electrons in a dielectric, all conduction electrons move in all directions, but they cannot transport any charge or spin. The existence of conduction electron in a dielectric is experimentally well- verified fact. For example, the light absorption and refractive index in the dielectric is purely determined by the conduction electrons for a short wavelengths when a photon energy is larger than the dielectric band gap.
There are conduction electrons in any solid: in a metal, in a semiconductor and even in a dielectric. The transport properties of conduction electrons depend on availability of unoccupied states for conduction electrons (or the same: the number of states at the Fermi energy). As I described above, in order to transport the charge and/or the spin it should be more electrons moving in one direction than in the opposite direction. Therefore, some unoccupied state should be available for conduction electrons moving in the forward direction. In a metal, there are plenty of unoccupied states and therefore the metal is a good conductor. In contrast, in a dielectric there is no any unoccupied state. Since all states are occupied, the electrons cannot be scattered from a state or into a state and therefore the number of moving in any specific direction cannot be changed. As a result, the conduction electrons cannot transport any charge or spin in a dielectric. It is the fact that there are lots of conduction electrons in a dielectric, all conduction electrons move in all directions, but they cannot transport any charge or spin. The existence of conduction electron in a dielectric is experimentally well- verified fact. For example, the light absorption and refractive index in the dielectric is purely determined by the conduction electrons for a short wavelengths when a photon energy is larger than the dielectric band gap.
(detail 2) ![]() You wrote: The hole is not an atom, it is not an electron.
You wrote: The hole is not an atom, it is not an electron.
The hole is the electron. The is not an atom, or a nucleus, or any type of a positively- charged particle or any kind of a void. The hole is a negatively- charged electron, which at condition in a solid behaves exactly as a positively- charged particle. A good example is a metal, in which the electrons of an energy higher than the Fermi energy are "electrons" and the electrons of an energy lower than the Fermi energy are "holes".
(the reason why the electron behaves as a positively-charged particle and why the Fermi energy is matter) ![]()
![]()
![]() As I explained above, an electron current flows when there are more electrons moving in the current direction than in the opposite direction. When the electrical field is applied, an electrons, which move along field, accelerate and gain energy. In contrast, the electrons, which move in the opposite direction, slow down and loss energy. The change of the electron energy changes the number of electrons moving in each direction. The dependence of the number of electrons on an electron energy is called the electron energy distribution (See here). As a result of applying of the electrical field, the energy distribution of electrons moving along the field shifts up and the energy distribution of electrons moving opposite to the field shifts down. It
As I explained above, an electron current flows when there are more electrons moving in the current direction than in the opposite direction. When the electrical field is applied, an electrons, which move along field, accelerate and gain energy. In contrast, the electrons, which move in the opposite direction, slow down and loss energy. The change of the electron energy changes the number of electrons moving in each direction. The dependence of the number of electrons on an electron energy is called the electron energy distribution (See here). As a result of applying of the electrical field, the energy distribution of electrons moving along the field shifts up and the energy distribution of electrons moving opposite to the field shifts down. It
(detail 3) ![]() You wrote: If you go back slightly in time, the Silicon atoms did not carry any electrical charge; the Boron atoms did not carry any electrical charge; the block of Silicon did not carry any electrical charge; nobody has connected the block of Silicon to a battery. Yet magically there are undefinable entities inside the block that carry positive charges that were not there before.
You wrote: If you go back slightly in time, the Silicon atoms did not carry any electrical charge; the Boron atoms did not carry any electrical charge; the block of Silicon did not carry any electrical charge; nobody has connected the block of Silicon to a battery. Yet magically there are undefinable entities inside the block that carry positive charges that were not there before.
(charge of doped silicon): Each the semi insulating (undoped) silicon, p-Si (e.g. silicon doped with boron) and n-Si (e.g. silicon doped with phosphorous) is absolutely uncharged. The total positive charge of all nucleases precisely equal to the total negative charge of all electrons.
(reason why undoped silicon is not conductive): The undoped silicon (i-Si) has a lot of conduction electrons, but there is no unoccupied state, the conduction electrons occupied all available quantum states and therefore the conduction electrons are unable to transport the Charge or the Spin. There is an energy band to the close unoccupied states and the conduction electrons cannot be scattered to those states. As I have explains above, in order to transport the Charge and the Spin, the conduction electrons should be able to change their direction distribution. In one direction it should be more electrons and in the opposite direction should be less. In the absence of unoccupied states the conduction electrons are unable to do that and therefore unable to do any transport. This reason id the same to the reason why the conduction electrons cannot make any current in a dielectric.
(reason why silicon doped with phosphorous becomes conductive): An atom of phosphorous has one more electron on outer shell than an atom of the Si and therefore one more of its electrons become a conduction electron . As a result of replacing a few of atoms of Si by atoms of phosphorous in the crystal of Si, the number of conduction electrons in Si slightly increases and the subtle balance between equal number of conduction electrons and their quantum states is broken. Since there are no available unoccupied states, these additional conduction electrons occupies the upper states (conduction band) of a higher energy, there is a huge number of unoccupied quantum states there. Therefore, these additional conduction electrons, which are provided by the phosphorous, are able to transport the Charge and the Spin.
(reason why silicon doped with boron becomes conductive): An atom of boron has a smaller number of electrons on outer shell than an atom of the Si and therefore less of its electrons become the conductive electrons. As a result of replacing a few of atoms of Si by atoms of boron in the crystal of Si, the number of conduction electrons in Si slightly decreases and the subtle balance between equal number of conduction electrons and their quantum states is broken. There are more available quantum states than the conduction electrons and some of quantum states become unoccupied. Since there are unoccupied states, the conduction electrons are are able to transport the Charge and the Spin. This main band is called the valence band and the unoccupied quantum states are called the holes.
(charged pn junction) At a contact of a p-Si and n-Si, some conduction electrons from n-Si move into p-Si and some non-conductive region is formed, in which the balance between the number of conduction electrons and quantum state become equal again. As a result, a tiny region at contact in the p-Si becomes negatively- charged and a tiny region in n- Si becomes positively- charged.
Energy distribution of solely- occupied and doubly- occupied states for conduction electrons in a non-magnetic material |
| A quantum state can be occupied either by one electron or two electrons of opposite spins. The energy distributions of these solely- and doubly- occupied states are different |
| The energy distribution of all conduction electrons are describes by the Fermi- Dirac distribution |
| (Why distributions are different) |
| (Difference of scattering probabilities) |
| method has been developed in 2014 by Zayets |
| Click on image to enlarge it |
I will try to answer your questions as soon as possible