Abstract:

Drift current is current drifted by an external applied electrical field. The drift current flows from a drain towards a source. Diffusion spin current is a diffusion of the spins from a region of the larger spin accumulation towards a region of the smaller spin accumulation. The properties of diffusion and drift currents are drastically different.

about difference between drift and diffussion currents you can read here

There is a significant difference between flows of the charge and the spin in a solid. In general the spins flow as a diffusion current and charge flows as a drift current

(There are some exceptions from this rule, but it is a good starting point to understand charge and spin transport)

Drift and Diffusion Currents

In a material there are two possible types of electron transport: diffusion and drift.

The drift current is a flow of charge particles along an applied electrical field.

The diffusion is a flow of uncharged particles from the region of their high concentration into regions of their lower concentration. In the case when the same amounts of electrons of opposite spins are flowing in opposite directions, in total there is no transport of charge, but there is only a transport of spin. Such transport can be considered as a flow of uncharged particles, which carry only spin. Therefore, spin may diffuse from region of higher spin concentrations into regions of smaller spin concentrations.

It should be noticed the fact that charged particles can not diffuse. The diffusion of charge particles creates a charge accumulation, which induces an electrical field, which stops further diffusion. However, the charged particles of opposite polarities can diffuse together in the same direction or the charged particles of same polarity can diffuse together in opposite directions. In both cases the diffusion does not cause a charge accumulation.

Drift and Diffusion Currents in Different materials

The properties of diffusion and drift currents may be substantially different for different materials. Generally, in metals the diffusion and drift currents do not interact with each other and they flow rather independently. In contrast, in the semiconductors there is a significant interaction between the diffusion and drift currents. It leads to several interesting effects. For example, in the semiconductors the spin diffusion length may be elongated or shorten, when drift charge current flows along spin diffusion. (Theory is here. Comparison with experiment is here).

The table below compares the basic properties of diffusion spin current and drift charge current in non-magnetic metals (near constant density of states near Fermi energy), ferromagnetic metals (different density of states for electrons with spin along and opposite to direction of the metal magnetization), non-degenerated semiconductor (any material, in which density of states is not constant near Fermi energy ) and the metals in which electron-electron interaction is strong.

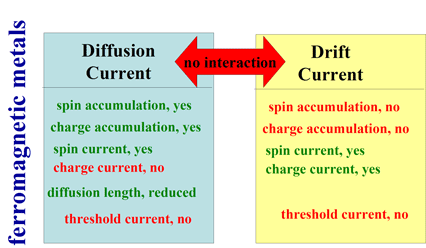
non-magnetic metals	ferromagnetic metals
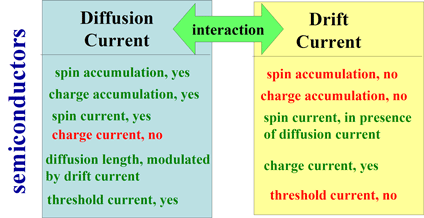
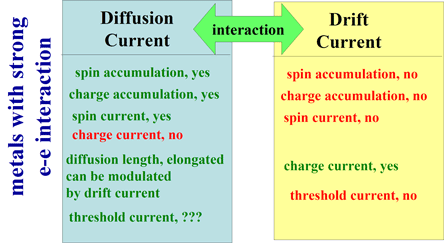
semiconductors	metals with strong e-e interaction

Spin chemical potential and charge chemical potential

Fig.1 The charge current flows between the charge source and the charge drain

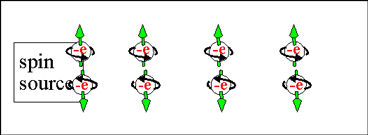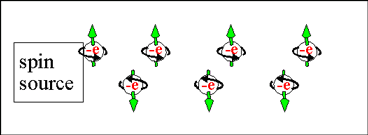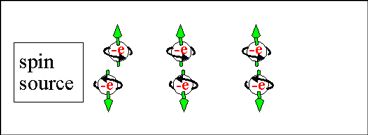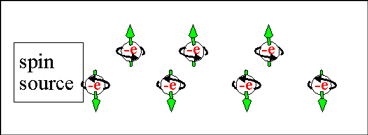Fig.2 The spin current is generated at the spin source and freely flows in all directions

The spin chemical potential describes the flow of the spin, the charge chemical potential 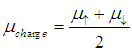describes flow of the charge. Theare the chemical potential for spin-up and spin-down electrons.

The spin chemical potential is linearly proportional to the spin accumulation in the material.

The charge chemical potential describes distribution of electrical field in the material and charge accumulation is proportional to the second derivation of the charge chemical potential.

External Electrical Field

When the external electrical field is applied, the chemical potentials for both spin-up and spin-down electrons obey

Therefore

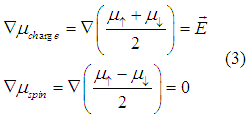
Form (3), there is a gradient of along electrical field. That means that the charge is drifted along the applied electric field.

describes a diffusion spin current. The diffusion spin current is a flow of spins from a region of the larger spin accumulation towards a region of smaller spin accumulation. and the spin current are not sensitive to the applied electrical field.

Source and Drain

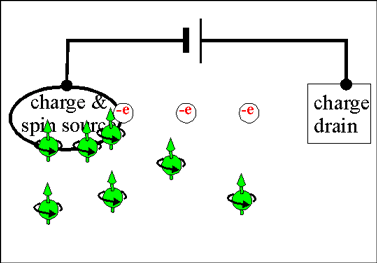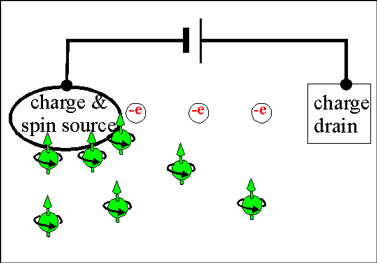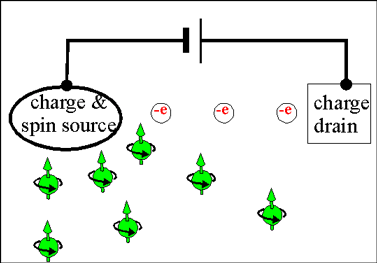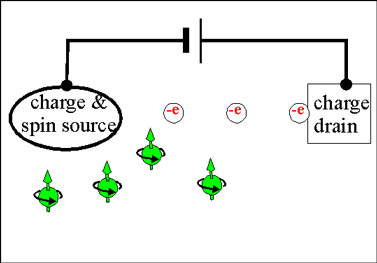Fig.2 The flows of spin and charge currents

Even though, spin and charge are undivided features of an electron, there is a significant difference between flows of charge and spin in a solid. In contrast to the charge current, which always flows from a source to a drain, the flow of the spin current does not require a drain. After having been emitted from a source, the spin current propagates in material until it decays.

Spin and charge accumulations

There is a significant difference between a charge accumulation and a spin accumulation. The charge accumulation induces an electrical field, which affects in the long range the charge distribution, electrical potential and charge current. In contrast, the magnetic field induced by the spin accumulation is weak. In the long range it does not affect the spin or charge distributions.

Intrinsic and effective spin diffusion lengths

The intrinsic spin diffusion length is the spin diffusion length, which is used in spin/charge transport equations. The intrinsic spin diffusion length is proportional to the spin relaxation rate as

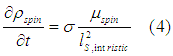
where 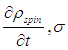are the spin relaxation rate and the conductivity, respectively.

The effective spin diffusion length is proportional to the decay rate of spin current, the spin accumulation and the spin chemical potential along the flow direction of the spin current.

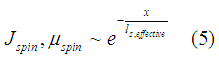
In the case of non-magnetic metals

In the case of ferromagnetic metals

In the case of semiconductors the effective spin diffusion length depends on the charge current flowing parallel to the spin current. Therefore, the effective spin diffusion length can be controlled by the charge current .

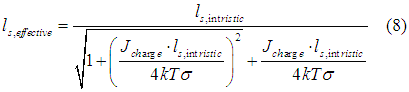
It should be noted that the spin drain affects the effective spin diffusion length.

When the spin flow in one direction (There is no flow in opposite direction)

Spin and charge currents in different materials

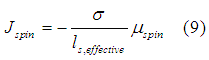
Non-magnetic metal

There is no interaction between the spin and charge currents.

Drift current.

Drift current does not induce a spin torque

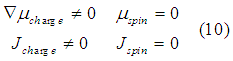
Diffusion current.

Diffussion current does induce a spin torque

ferromagnetic metal

There is no interaction between the dc spin and charge currents, but there is an interaction between the ac spin and charge currents, because of a charge accumulation along the flow direction of diffusion spin current.

Drift current.

Drift current does not induce a spin torque. It has a spin-current component.

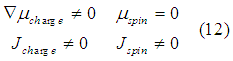
Diffusion current.

Diffusion current induces a spin torque. There is a charge accumulation along flow of diffusive current.

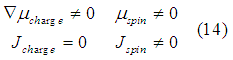
semiconductors

The conductivity of a semiconductor is spin-dependent only in the presence of a spin accumulation. There is a substantial interaction between the diffusion and drift currents in semiconductors.

Drift current.

The properties of the drift current differ depending on whether the drift current is flowing in the presence of a diffusive spin current or not.

When there is no diffusive current, the properties of the drift current is described by Eqn. (10). In the presence of a diffusive current, the properties of the drift current is described by Eqn. (12).

Diffusion current.

It induces a spin torque. There is a charge accumulation along flow of diffusive current. The properties of the diffusion current are different depending whether the diffusion current flowing in presence of drift spin current or not. For example, the effective diffusion length depends on the magnitude of the drift current.

The diffusion current in semiconductor satisfies

Ohm's law for spin current

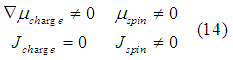
In the simplest case of spin/charge transport in non-magnetic metals, the charge and spin currents satisfy

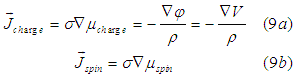
where are electrical potential, conductivity and voltage, respectively. Eqn. (9a) is the Ohm's law, which describes the relation between the electrical voltage and the charge current. Similarly, Eqn. (9b) could be considered as the Ohm's law for spin current. When the spin current flows only in one direction (There is no flow in opposite direction) , Eqn. (9b) is simplified to

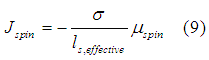
can be treated as spin voltage. Then, if we define spin resistance sR for non-magnetic metals as

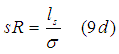
and the Ohm's law for spin current will be

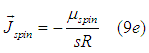
in the case of ferromagnetic metals the spin resistance sR will be

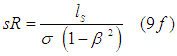
In materials with low spin resistance (low resistance +short spin diffusion length) (example: ferromagnetic metal Fe, Co or metals:Pt), a small induces a large spin current. These materials have a strong spin-drain strength, but a small spin-detection efficiency. In contrast, in materials with high spin resistance (high resistance +long spin diffusion length), even when spin current is weak the is large. These materials have a weak spin-drain strength, but a significant spin-detection efficiency.

Spin-transfer torque

Spin-transfer torque is proportional to the spin relaxation rate. It only occurs when there is a spin accumulation. Therefore, only a spin diffusive current induces the spin transfer torque. The drift current does not induce any spin-transfer torque. Even in case of ferromagnetic metals, when a drift current has a spin-current component, the drift current does not induce the spin-transfer torque.

The signs of the spin-transfer torque are different for material magnetizations aligned either parallel or anti-parallel to the spin polarization of diffusive spin current. In the case of ferromagnetic metals, which have a spontaneous magnetization, the spin-transfer torque may reverse the magnetization direction or may cause magnetization precession. In the case of semiconductors or non-magnetic metals, the spin-transfer torque may cause precession of the induced magnetization.

The spin transfer torque occurs because along the flow of spin diffusive current the number of electrons, which flip their spin in the direction parallel to the magnetization of material and in opposite direction, are different.

Let us consider a case of a spin accumulation when the number of spin up electrons is above equilibrium and the number of spin-down electrons is below equilibrium. Along the flow of the diffusion spin current the number of flipped spin-up electrons is larger than the number of flipped spin-down electrons. Therefore, the spin accumulation decays along the flow direction. The spin-transfer torque is proportional to the decay rate of the spin accumulation. Therefore, it is proportional to the difference of the flipped spin-down and spin-up electrons:

where DS is the density of states and is reduction of spin chemical potential per flow distance .

Substituting (5) into (15) gives

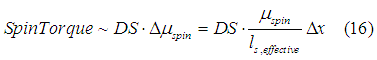
Therefore, the spin transfer torque is linearly proportional to 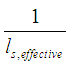. The shorter the effective spin diffusive length is, the stronger the spin transfer torque becomes. It should be noticed that the strength of the spin-transfer torque in semiconductors can be modulated by a charge current (See Eqn. (8) ).